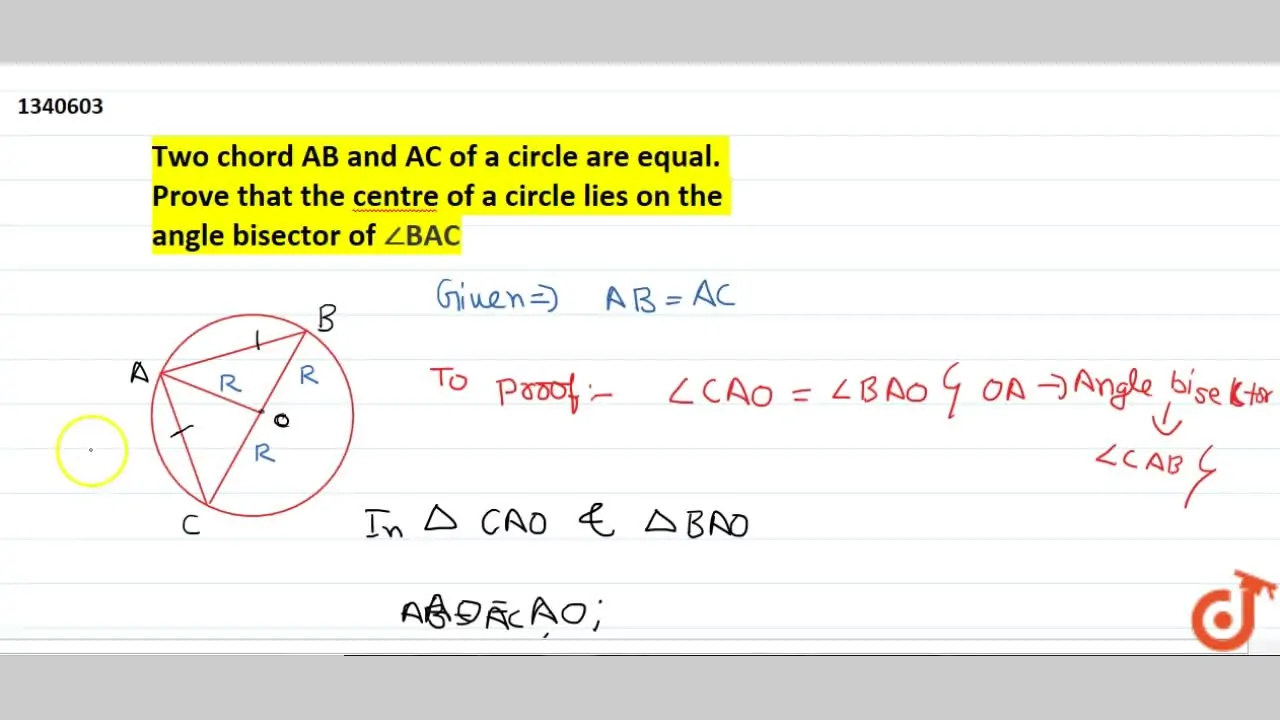
Two chord AB and AC of a circle are equal. Prove that the centre of a circle lies on the angle bisector of ∠BAC
Step by step video & image solution for Two chord AB and AC of a circle are equal. Prove that the centre of a circle lies on the angle bisector of /_BAC by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Similar Questions
Two chords AB and AC of a circle are equal. Prove that the centre of the circle lies on the angle bisector of ∠BAC.
View SolutionIf two chords AB and AC of a circle with centre O are such that the centre O lies on the bisector of ∠BAC, prove that AB=AC , i.e. the chords are equal.
View SolutionIf two chords AB and AC of a circle with centre O are such that the centre O lies on the bisector of ∠BAC; Prove that AB=AC
View SolutionTheorem:-4(iii) If two equal chords are drawn from a point on the circle; then the centre of a circle will lie on angle bisector of these two chords .
View SolutionIn the given figure, AB and AC are two equal chords of a circle with centre O. Show that O lies on the bisectors of ∠BAC.
View SolutionEqual chords of a circle subtend equal angles at the centre.
View SolutionProve that equal chords of a circle subtend equal angles at the centre.
View SolutionProve that the centre of a circle touching two intersecting lines lies on the angle bisector of the lines.
View SolutionIf the length of a chord of a circle is equal to that of the radius of the circle , then the angle subtended , in radians , at the centre of the circle by the chord is
View SolutionLet two chords AB and AC of the larger circle having same centre touch the smaller circle having same centre at X and Y. Then XY = ?
View Solution