The displacement by the bolt during its free fall time w.r.t. ground frame
0.3 m
0.7 m
0.9 m
1 m
The correct Answer is:B
Topper's Solved these Questions
- MOTION IN A STRAIGHT LINEBook:NARAYNAChapter:MOTION IN A STRAIGHT LINEExercise:Level 1 H.W
Explore 23 Videos
- MOTION IN A STRAIGHT LINEBook:NARAYNAChapter:MOTION IN A STRAIGHT LINEExercise:Level 2 H.W
Explore 29 Videos
- MOTION IN A STRAIGHT LINEBook:NARAYNAChapter:MOTION IN A STRAIGHT LINEExercise:Single Answer Question
Explore 8 Videos
- MOTION IN A PLANEBook:NARAYNAChapter:MOTION IN A PLANEExercise:Level-II(H.W)
Explore 31 Videos
- NEWTONS LAWS OF MOTIONBook:NARAYNAChapter:NEWTONS LAWS OF MOTIONExercise:PASSAGE TYPE QUESTION
Explore 6 Videos
Similar Questions
Knowledge Check
An elevator car whose floor to ceiling distance is equal to 2.7m starts ascendiung with constant acceleration 1.2ms2, 2 sec after the start a bolt begins falling from the ceiling of the car. Answer the following question g=9.8m/s2
Distance covered by the bolt during the free fall time w.r.t. ground frame.A0.7 mB0.9 mC1.1 mD1.3 mconsider the acceleration velocity and displacement of a tennis ball as it falls to the ground and bouces back. Derections of which of these changes in the process
Avelocity onlyBDisplacemetn and velocityCAcceleration, velocity and displacementDDisplacement and accelerationIf d denotes the distance covered by a car in time t and →s denotes the displacement by the car during the same time, then :
Ad≤∣∣→S∣∣Bd=∣∣→S∣∣ onlyCd≥∣∣→S∣∣Dd≤∣∣→S∣∣
The displacement x of the body is motion is given by x=A sin (omega t+ theta) , Determine the time at which the displacement is maximum.
View SolutionA man of mass m1 is standing on a platform of mass m2 kept on a smooth horizontal surface. If the man moves a distance d w.r.t., the platform, find the displacement of the platform w.r.t., ground.
View SolutionIf a person is pushing a box inside a moving train , the work in the frame of earth will be:
→s0= displacement of the train relative to ground.
→s=displacement of the box w.r.t. train.
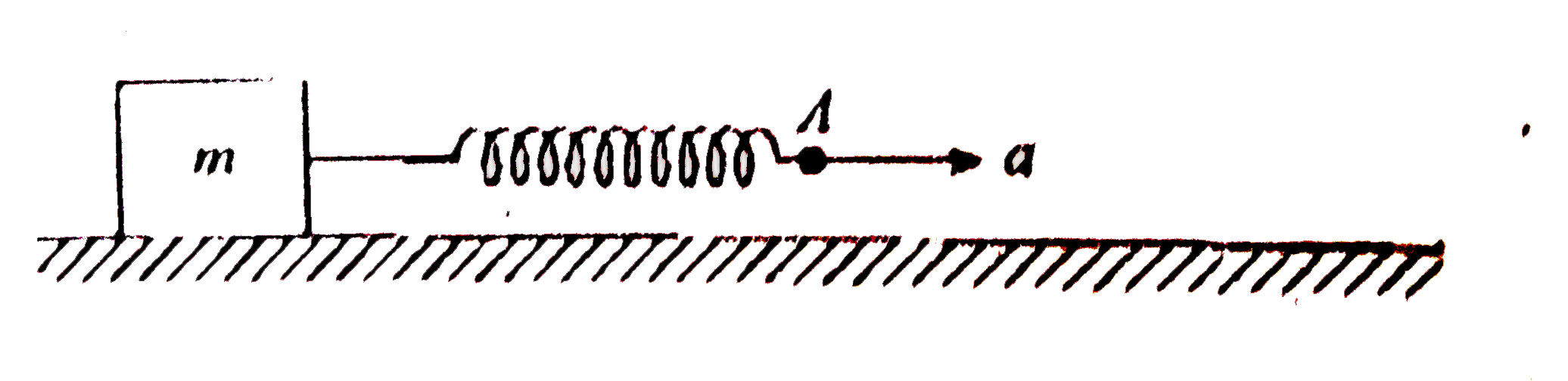
View SolutionA block ofmass m is attached with a spring in its natural length, of spring constant k. The other end A of spring is moved with a constant acceleration 'a' away from the block as shown in the figure−3.74. Find the maximum extension in the spring. Assume that initially block and spring is at rest w.r.t ground frame:
View SolutionThe displacement of a particle is given at time t, by:
x=Asin(−2ωt)+Bsin2ωt Then,
View Solution