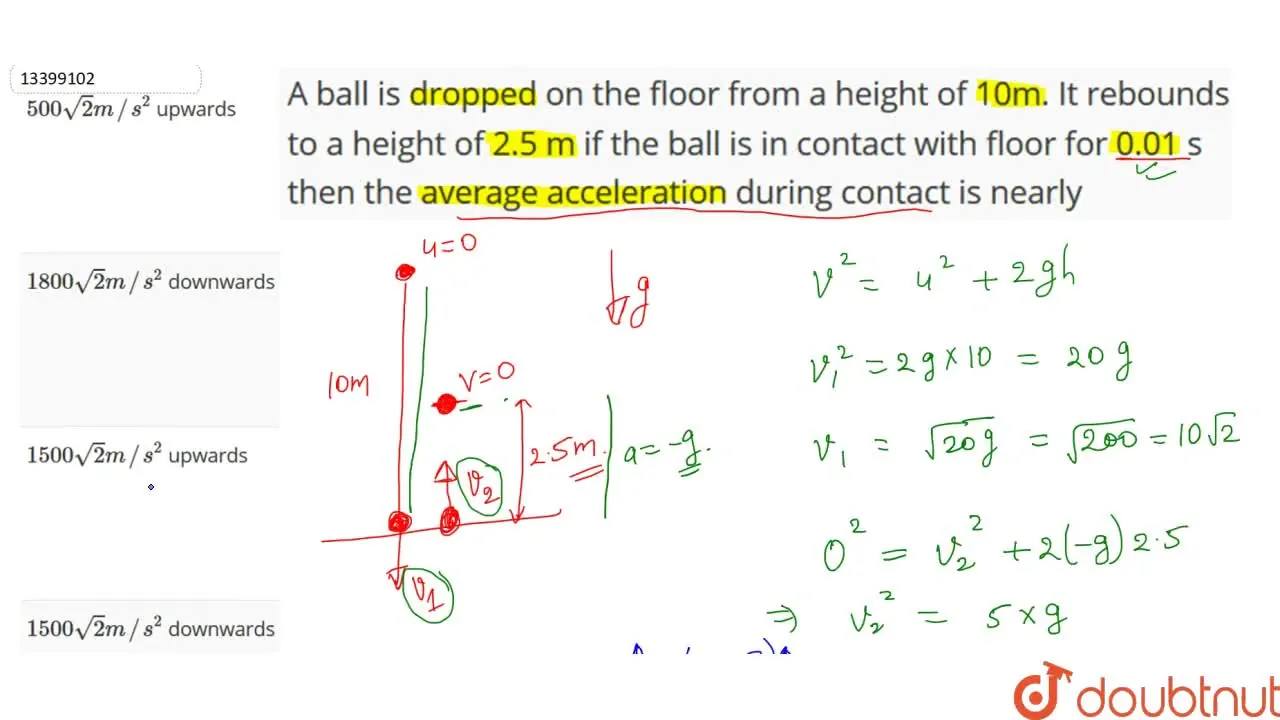
A ball is dropped on the floor from a height of 10m. It rebounds to a height of 2.5 m if the ball is in contact with floor for 0.01 s then the average acceleration during contact is nearly
500√2m/s2 upwards
1800√2m/s2 downwards
1500√2m/s2 upwards
1500√2m/s2 downwards
The correct Answer is:C
a=√2gh2−(−√2gh1)Δt
Related Playlists
- BOOK - NARAYNACHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - Level 2 C.W25videos
MOTION IN A STRAIGHT LINE
- BOOK - NARAYNACHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - Level 3 C.W25videos
MOTION IN A STRAIGHT LINE
- BOOK - NARAYNACHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - C.U.Q42videos
MOTION IN A STRAIGHT LINE
- BOOK - NARAYNACHAPTER - MOTION IN A PLANEEXERCISE - Level-II(H.W)31videos
MOTION IN A PLANE
- BOOK - NARAYNACHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - PASSAGE TYPE QUESTION6videos
NEWTONS LAWS OF MOTION
Similar Questions
A ball is dropped on the floor from a height of 10m. It rebounds to a height of 2.5 m if the ball is in contact with floor for 0.01 s then the average acceleration during contact is nearly
View SolutionA ball is dropped on to the floor from a height of 10 m. It rebounds to a height of 2.5 m. If the ball is in contact with the floor for 0.01 sec, what is the average acceleration during contact ?
View SolutionA ball is dropped on the floor a height of 80m rebounds to a height of 20m. If the ball is in contact with floor for 0.1 s, the average acceleration during contact is
View SolutionA tennis ball is dropped on the floor from a height of 20m. It rebounds to a height of 5m. If the ball was in contact with the floor for 0.01s. What was its average acceleration during contact? (g=10m/s2)
View SolutionA ball is dropped onto the floor from a height of 10 m. It rebounds to a height of 5 m. If the ball was in contact with the floor for 0.01s, what was its average acceleration during contact ? (Take g=10ms−2)
View SolutionA tennis ballis dropped on to the floor from a height of 4m. It rebounds to a height of 2m. If the ball is in contact with the floor for 12×10−3s, its average acceleration during
View SolutionA tennis ball is dropped on to the floor from a height of 4.00m. It rebounds to a height of 3.00m. If the ball was in contact with the floor for 0.010sec, what was its average acceleration during contact?
View SolutionTo test the quality of a tennis ball, you drop it onto the floor from a height of 4.00 m. It rebounds to a height of 2.00 m. If the ball is in contact with the floor for 12.0 ms, what is its average acceleration during that contact? Take g=98m/s2.
View SolutionA ball is dropped from a height of 10 m, as it falls,
View SolutionA ball is dropped form a height of 20 cm . Ball rebounds to a height of 10 cm . What is the loss of energy ?
View Solution