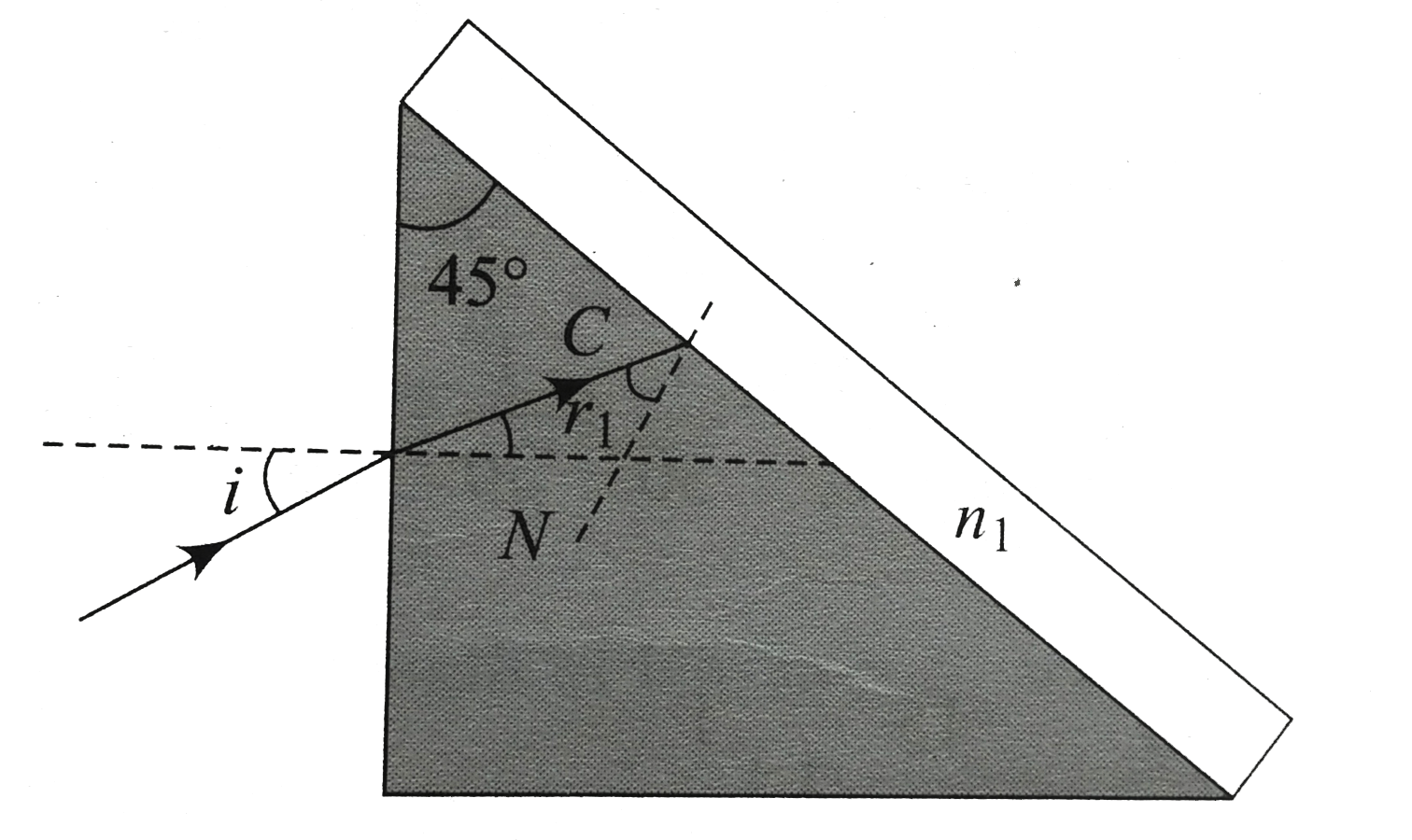
A right angles prism $(45^{\circ}, 90^{\circ}, 45^{\circ})$ of refractive index n has a plate of refractive index $(n_{1} < n)$ cemented to its diagonal face. The assembley is in air. A ray is incident on AB.
a. Calculate the angle of incidence at AB for which the ray strikes the diagonal face at the critical angle.
b. Assuming $n = 1.351$ , calculate the angle of incidence at AB for which the refracted rey passes through the diagonal face undeviated.
$A right angles prism (45^(@),90^(@), 45^(@)) of refractive index n has a plate of refractive index (n(1)ltn) cemented to its diagonal face. The assembley is in air. A ray is incident on AB. a. Calculate the angle of incidence at AB for which the ray strikes the diagonal face at the critical angle. b. Assuming n=1.351, calculate the angle of incidence at AB for which the refracted rey passes through the diagonal face undeviated.$

Video Solution

Text Solution

Verified by Experts

(a)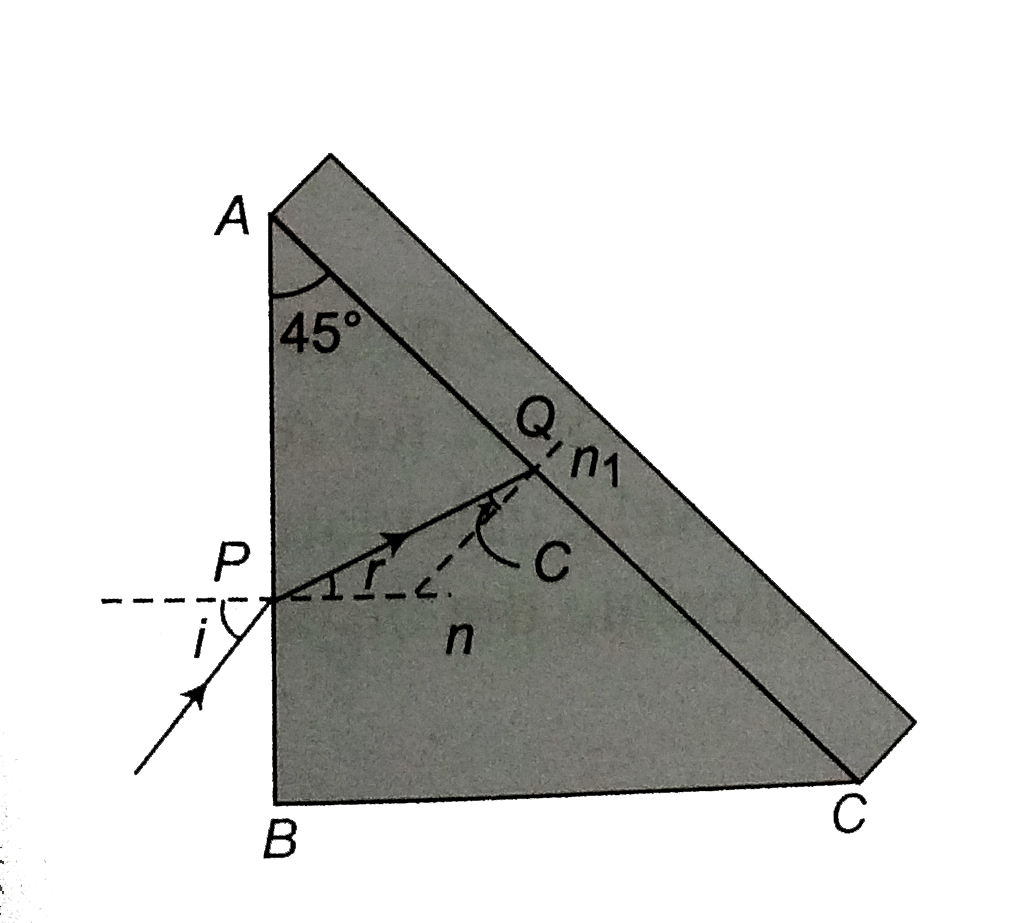
$(n_{1} < n)$
$n sin C = n_{1} {sin 90}^{\circ}$
$sin C = n_{1} / n$
In $Δ A P Q$ ,
$90^{\circ} - r + 90^{\circ} - C + 45^{\circ} = 180^{\circ}$
$r + C = 45^{\circ} \Rightarrow r = 45^{\circ} - C$
Refraction at AB:
$1 \times sin i = n sin r$
$= n sin (45^{\circ} - C)$
$= n {sin 45}^{\circ} cos C - {cos 45}^{\circ} sin C$
$= n ⎡ ⎣ \frac{1}{\sqrt{2}} \sqrt{1 - \frac{n_{1}^{2}}{n^{2}}} - \frac{1}{\sqrt{2}} \frac{n_{1}}{n_{2}} ⎤ ⎦$
$= \frac{1}{\sqrt{2}} [\sqrt{n^{2} - n_{1}^{2}} - n_{1}]$
$i = {sin}^{- 1} [\frac{1}{\sqrt{2}} {\sqrt{n^{2} - n_{1}^{2}} - n_{1}}]$
(b) If refracted ray passes undeviated through AC, for this ray must be incident normally on AC i.e. $r^{'} = 0$
$r + r^{'} = A \Rightarrow r = 45^{\circ}$
$1 \times sin i = n {sin 45}^{\circ} = \frac{1.352}{\sqrt{2}}$
$i = {sin}^{- 1} (\frac{1.352}{\sqrt{2}}) = {sin}^{- 1} (0.96)$

Updated on:21/07/2023