A mass m = 50 g is dropped on a vertical spring of spring constant 500 N nfl from a height h= 10 cm as shown in figure (18-E14). The mass sticks to the spring and executes simple harmonic oscillations after that. A concave mirror of focal length 12 cm facing the mass is fixed with its principasl axis coinciding with the lilne of motion of the mss, its pole being at a distance of 30 cm from the free end of the spring. Find the length in which the image of the mass oscillates

Video Solution

Text Solution

Verified by Experts

x: maximum compression of spring
Applying energy conservation (1) and (2)
$m g (h + x) = \frac{1}{2} k x^{2}$
$\frac{50}{1000} x 10 (0.1 + x) = \frac{1}{2} \times 500 x^{2}$
$500 x^{2} - x - 0.1 = 0$
$x = \frac{1 \pm \sqrt{1 + 4 \times 500 \times 0.1}}{2 \times 500} = 0.015 m = 1.5 c m$
The block is in equilibrium when $m g = k x_{0}$
$\frac{50}{1000} \times 10 = 500 x_{0} \Rightarrow x_{0} = 10^{- 3} m = 0.1 c m$
Amplitude of oscillation $= x - x_{0} = 1.5 - 0.1 = 1.4 c m$
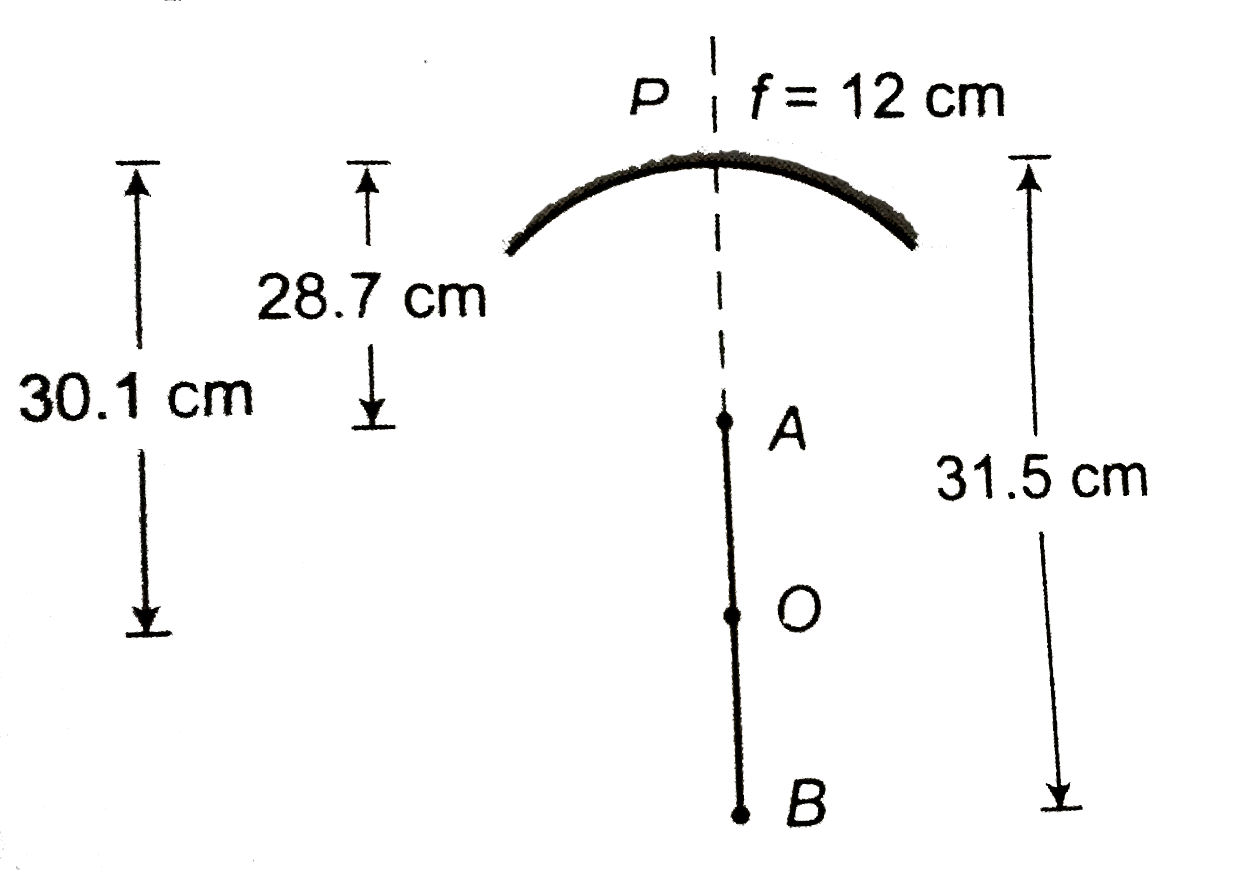
O: equilibrium position $P O = 30.1 c m, P B = 31.5 c m$
image of A:
$\frac{1}{v_{A}} + \frac{1}{- 28.7} = \frac{1}{- 12}$
$v_{A} = - 20.6 c m$
Image of B:
$\frac{1}{v_{B}} + \frac{1}{- 31.5} = \frac{1}{- 12}$
$v_{B} = - 19.4 c m$
The length in which image oscillates
$= 20.6 - 19.4 = 1.2 c m$

Updated on:21/07/2023