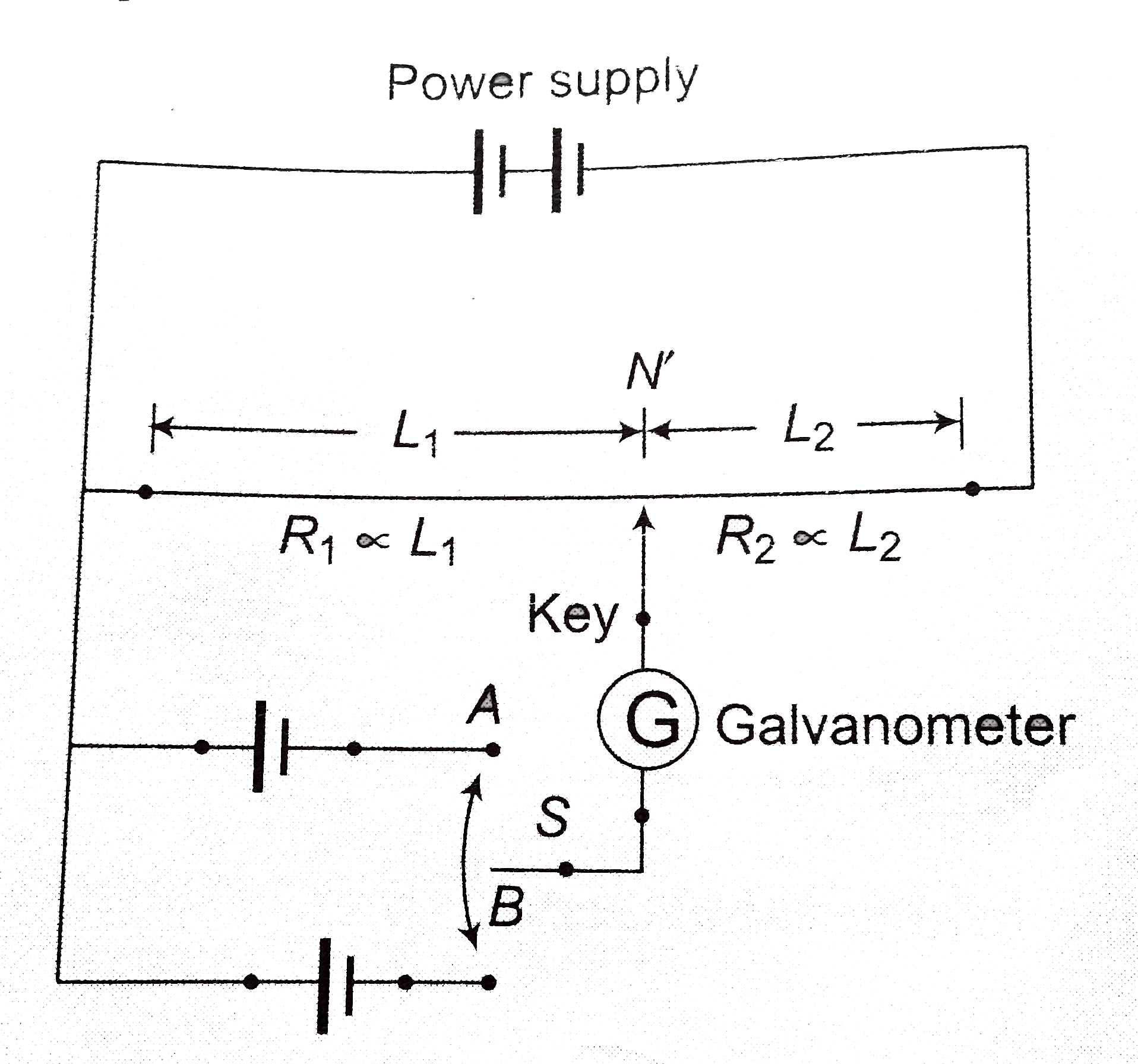
A slide-wire potentiometer is shown in the figure. In this device the resistances R1 and R2 of figure are actually a uniform wire 1.000m in length. Hence, the resistance R1 is alphaortional to length L1 and the resistance R2 is alphaortional to the length L2. the galvanometer junction N' in the figure is sharp-edged key that can slide along the wire and be made to make contact with ot at any position along its length. When a standard cell with an emf of 1.6V is used and the switch S is in position A, the length L1 is 40cm for no current to flow through the galvanometer. When the switch is moved to position B, the length L1 must be changed to 60cm for tge galvanometer to carry no current. What is the value of the emf E2 ?
E1E2=l1l2
1.6E2=4060⇒E2=2.4V.
Topper's Solved these Questions
Similar Questions
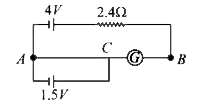
A simple potentiometer circuit is shown in the figure. The internal resistance of the battery is negligible. AB is a uniform wire of length 100cm and resistance 2Ω What would be the length AC for zero galvanometer deflection (in mm)?
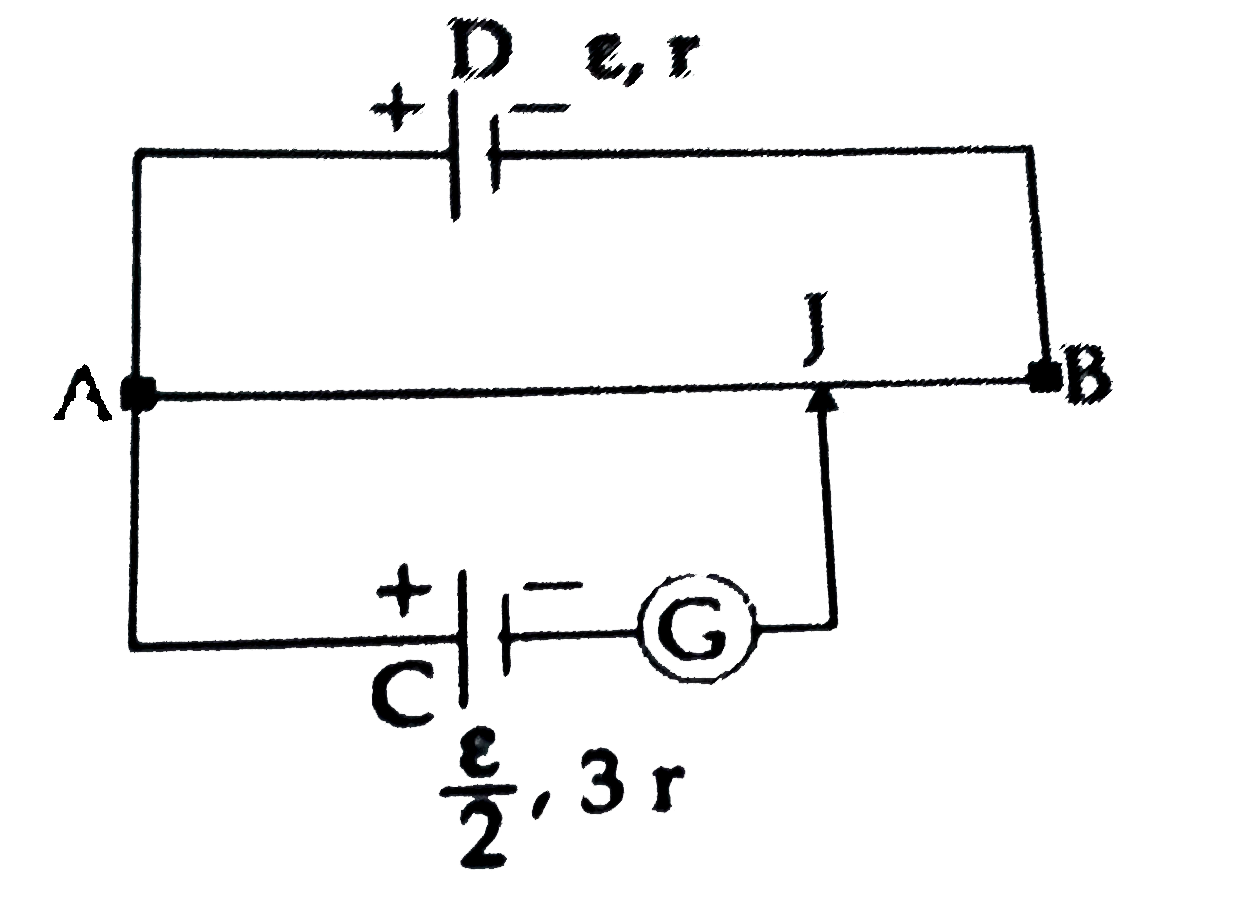
View SolutionA potentiometer wire AB having length L and resistance 12 r is joined to a cell D of emf ε/2 and internal resistance 3r is connected. The length AJ at which the galvanometer as shown in fig. Shows no deflection is :
View Solution
Knowledge Check
In the circuit shown in the given figure, the resistances R1andR2 are respectively
 A14Ωand40ΩB40Ωand44ΩC40Ωand30ΩD14Ωand30Ω
A14Ωand40ΩB40Ωand44ΩC40Ωand30ΩD14Ωand30ΩAB is a uniform resistance wire of 1 m length. The galvanometer G shows no deflection when the length AC = 20 cm. The resistance R is equal to
 A2ΩB8ΩC20ΩD40Ω
A2ΩB8ΩC20ΩD40ΩIf a uniform wire of resistance R is uniformly strethced to n times the orginal length , then new resistance of the wire becomes
AnRBn2RCR/nDR/n2
A potentiometer wire AB having length L and resistance 12 r is joined to a cell D of emf ε/2 and internal resistance 3r is connected. The length AJ at which the galvanometer as shown in fig. Shows no deflection is :

View SolutionWe have one wire of resistance R1 and it is stretched to n times its initial length to change its resistance to R2 calculate R2/R1 .
View SolutionA wire of length l has a resistance R. If half of the length is stretched to make the radius half of its original value, then the final resistance of the wire is

View SolutionIn the figure given below find the resistance between points A and B. Both the circle and the diatmeter are made of uniform wire of resistance rΩ per meter. The length AB is 2 metre.

View SolutionThe resistance of a wire of uniform diameter d and length L is R . The resistance of another wire of the same material but diameter 2d and length 4L will be
View Solution
