Two short dipoles , each of dipole moment P are placed at a large separation r. The force between them
is proportional to product of dipole momenta
is inversely proportional to r4
the force is attractive , if direction of dipole momenta are same , repulsive if opposite
all options are correct
The correct Answer is:4
Step by step video, text & image solution for Two short dipoles , each of dipole moment P are placed at a large separation r. The force between them by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
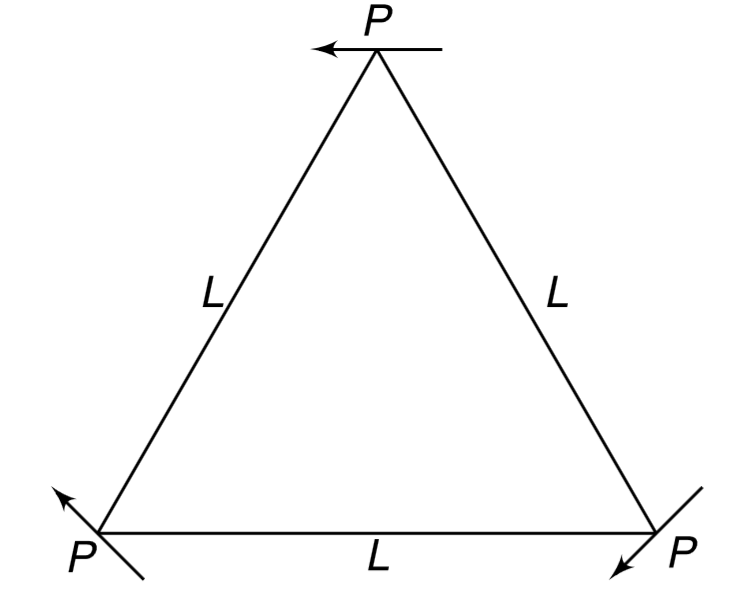
Three short electric dipoles, each of dipole moment P, are placed at the vertices of an equilateral triangle of side length L. Each dipole has its moment oriented parallel to the opposite side of the triangle as shown in the fig. Find the electric field and potential at the centroid of the triangle
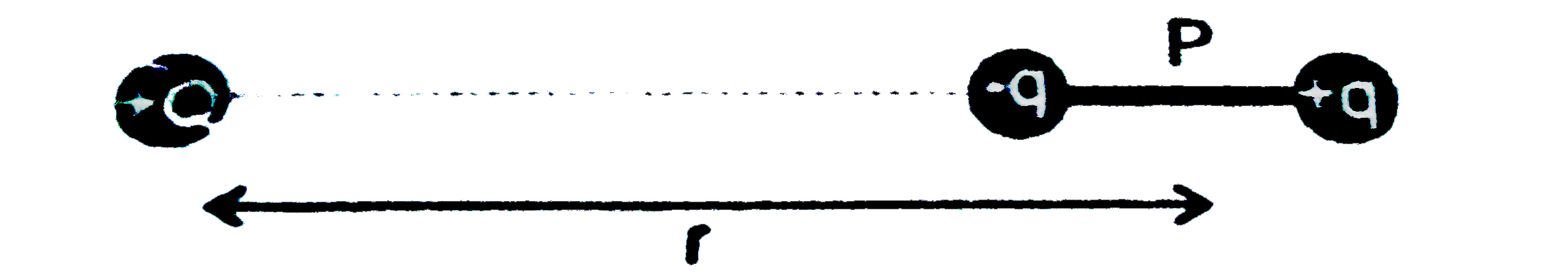
View SolutionA short dipole of dipole moment P is placed near a point charge Q as shown in figure. Find force on the dipole due to the point charge
View Solution
Knowledge Check
Two short dipoles, each of diple moment p , are placed at origin. The dipole moment of one dipole is along x-axis, while that of other is along y-axis . The electric field at a point (a,0) is given by
A(14πε0)2pa3B(14πε0)pa3C(14πε0)√5pa3DZeroFour short dipoles each of dipole moment 'p' are placed at the vertices of a square of side a. The direction of the dipole moments are shown in the figure.
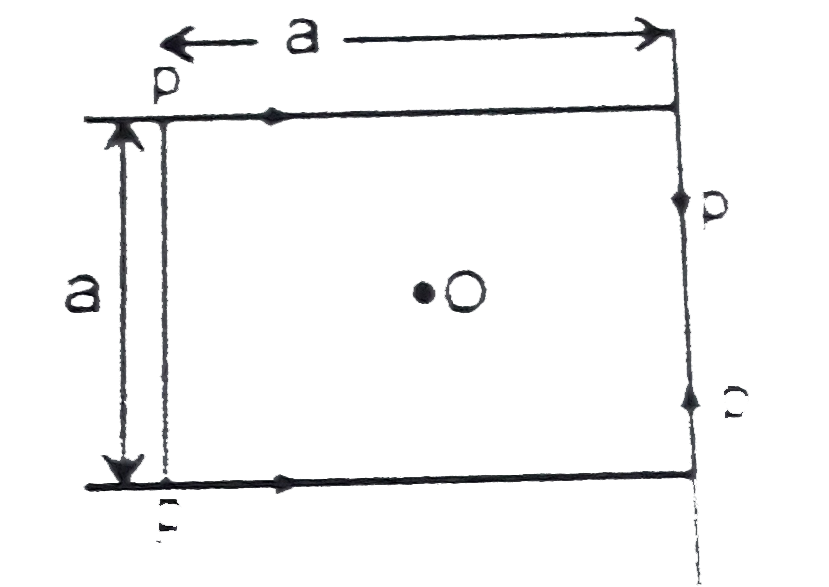 AElectric field at O is √2p2π∈0a3BElectric field at O is √2pπ∈0a3CElectrostatic potential at O is zeroDNet dipole moment is 2p
AElectric field at O is √2p2π∈0a3BElectric field at O is √2pπ∈0a3CElectrostatic potential at O is zeroDNet dipole moment is 2pTen dipoles ,each of dipole moment 'p' , are placed inside a hollow sphere . The total electric flux coming out of the sphere will be
A0B10p∈0Cp∈0D20p∈0
A short dipole of dipole moment P is placed near a point charge Q as shown in figure. Find force on the dipole due to the point charge

View SolutionTwo electric dipoles , each of dipole moment p=6.2×10−30 C m are placed with their axes along the same line their centre a distance d=10−8 m apart . Calculate the force of attraction between the dipoles .
View SolutionTwo electric dipoles are placed in such a manner that their electric dipole moments are directed towards each other. The force between them will be
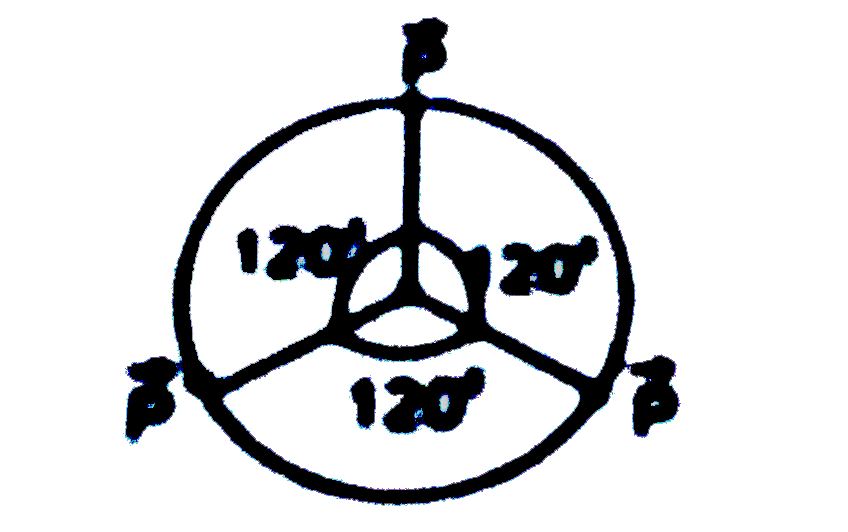
View SolutionThree dipoles each of dipole moment of magnitude p are placed tangentially on a circle of radius R in its plae positioned at equal angle from each other as shown in the figure. Then the magnitude of electric field intensity at the centre of the circle will be :
View SolutionTwo small electric dipoles each of dipole moment pˆi are situated at (0,0,0) and (r,0,0). The electric potential at a point (r2,√3r2,0) is:
View Solution