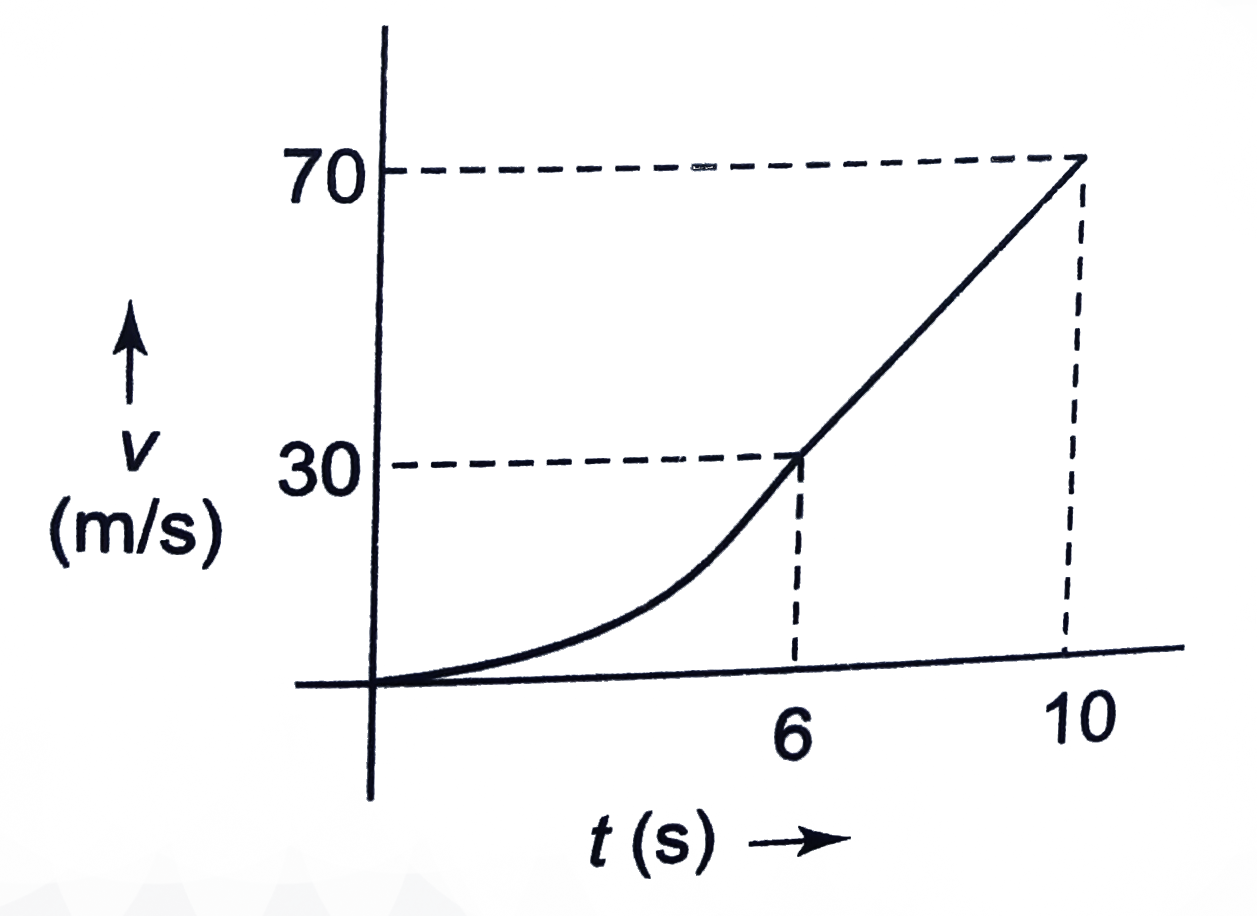
A particle is moving in a straight line and its acceleration-time graph is shown below. At t=0, the particle is at rest. Find the velocity of the particle at t=3s,6s and 8s. Also sketch the v-s graph.
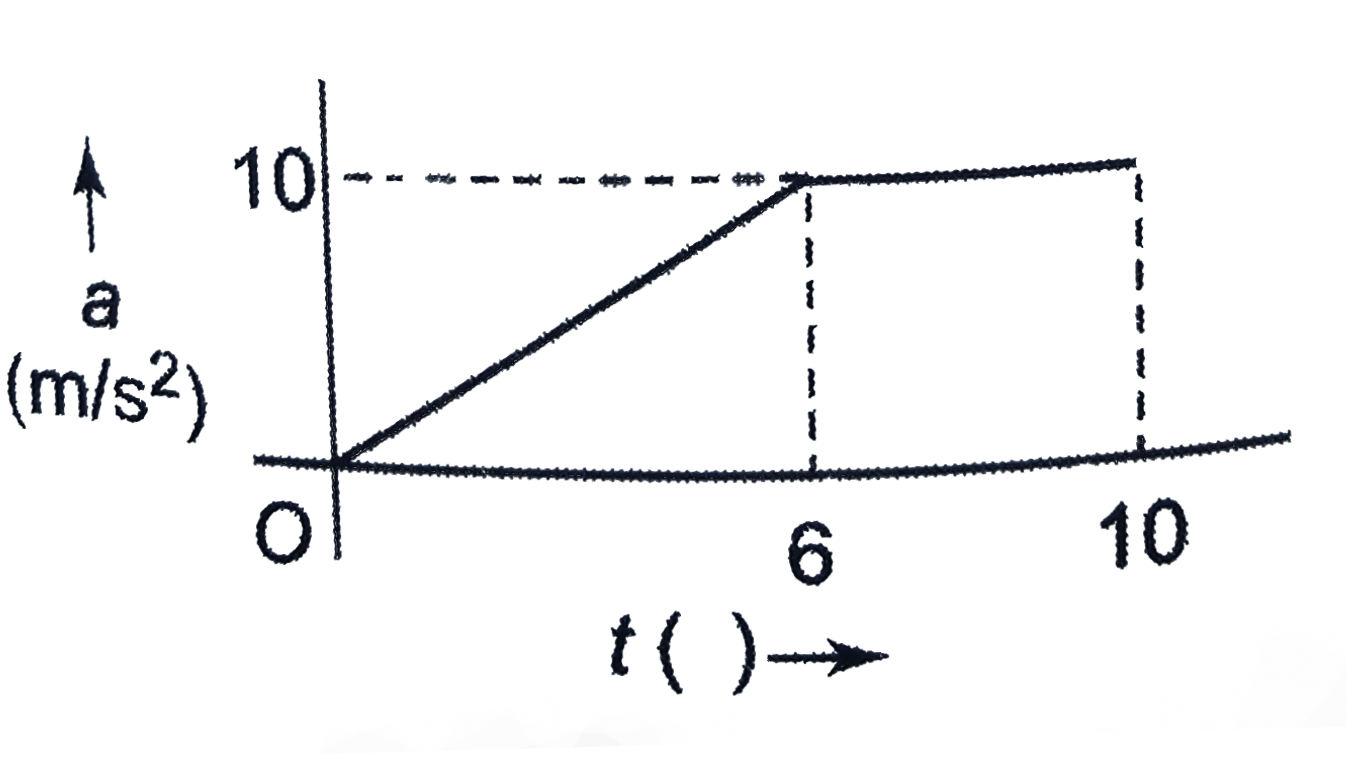
Given t=0,vt=0=0
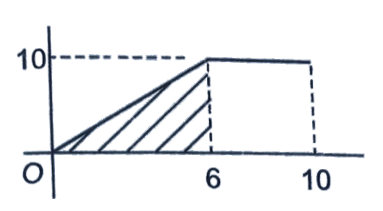
Area for the a-t graph for t=0 to t=6s=12×10×6=30
vt=6−vt=0=30⇒vt=6=30m/s
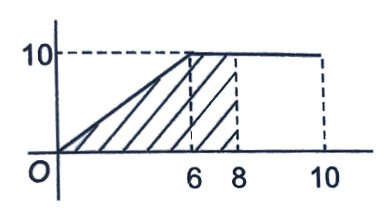
From t=0 to t=8s, Area=12×10×6+10×2=50
vt=8−vt=0=50⇒vt=8=50m/s
To calculate the velocity at t=3s, first find the acceleration at t=3s
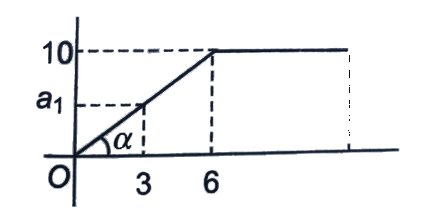
tanα=a13=106⇒a1=5m/s2
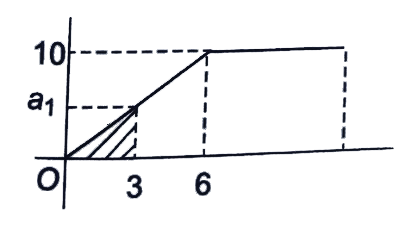
from t=0 to t=3s, Area=12×a1×3=12×5×3=7.5
vt=3−vt=0=7.5⇒vt=3=7.5m/s
vt=10−vt=0=Area for t=0 to t=10s=70
vt=10=70m/s
From t=0 to t=6s, a is +ve and increase and hence, the slope of the v-t graph is +ve and increasing.
From t=6 to t=10s, a is +ve and constant and hence, the slope of the v-t graph is +ve and constant, graph will be an inclined straight line.
Topper's Solved these Questions
Similar Questions
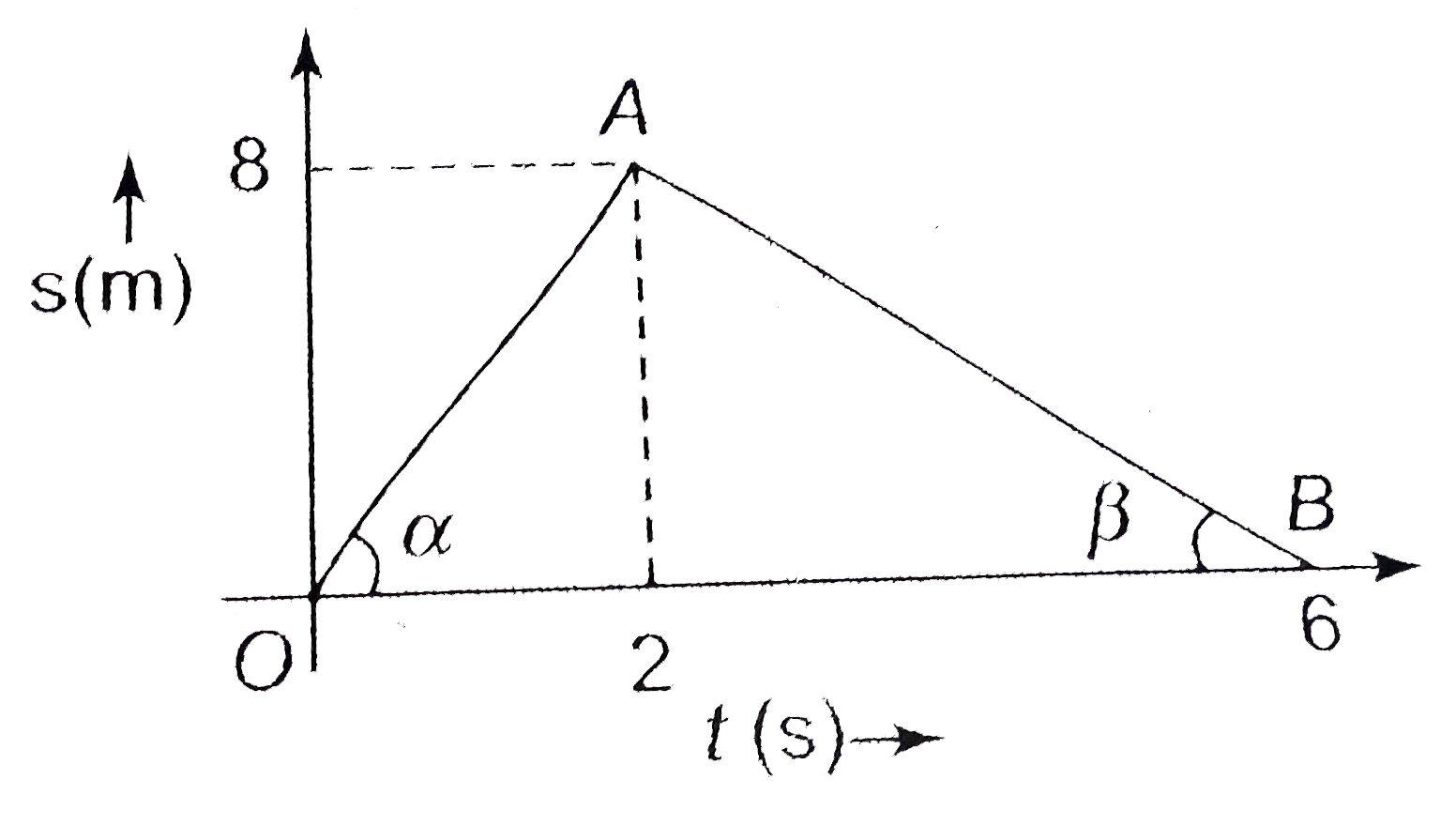
For a particle moving in a straight line, the position-time graph is as shown in the figure. Find the velocity of the particle at time t=1s and t=4s. Also sketch the v-t graph.
View SolutionA particle starts motion from rest and moves along a straight line. Its acceleration-time graph is shown. Find out speed of particle at t=2s and at t=3s.

View Solution
Knowledge Check
A particle starts from rest at t=0 and moves in a straight line with an acceleration as shown below. The velocity of the particle at t=3 s is
 A2m/sB3m/sC4m/sD6m/s
A2m/sB3m/sC4m/sD6m/sA particle starts from rest at t=0 and moves in a straight line with an acceleration as shown below. The velocity of the particle at t=3 s is
 A5m/s2B6m/s2C10m/s2D15m/s2
A5m/s2B6m/s2C10m/s2D15m/s2A particle is moving in a straight line according to graph given below : (a is acceleration, v is velocity, x is position, t is time) :
 A
A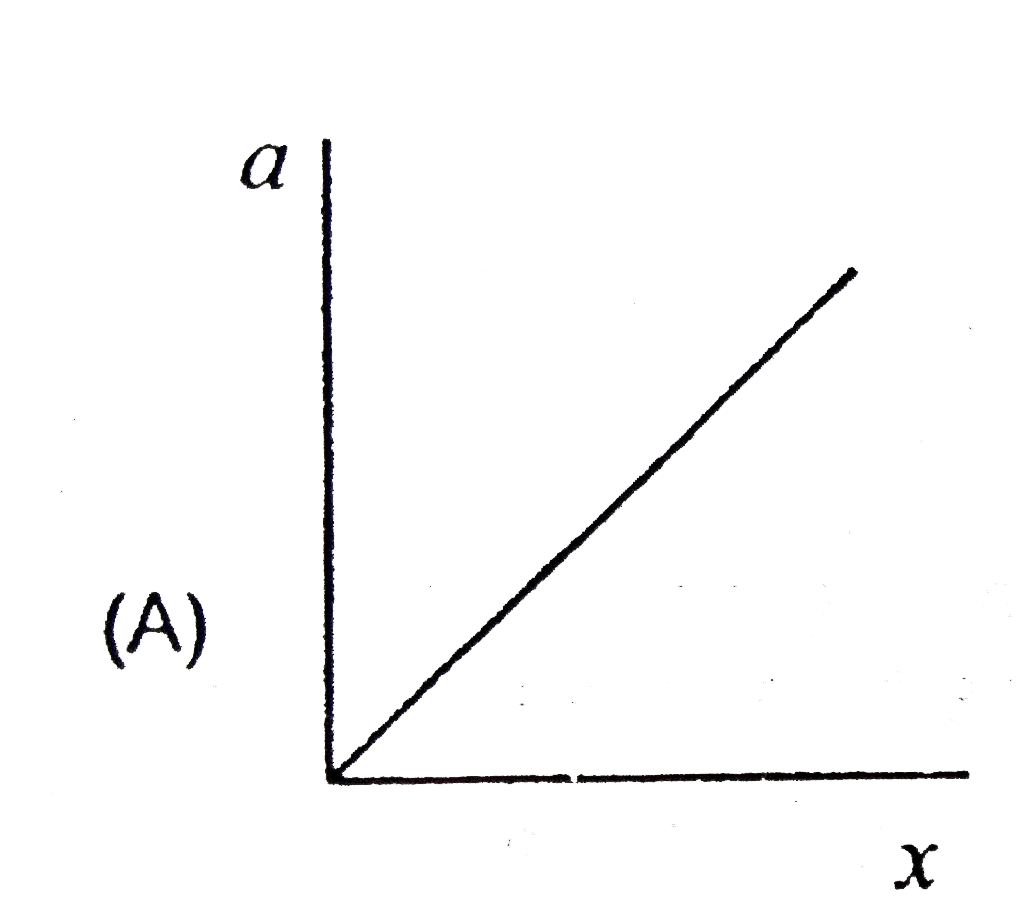 B
B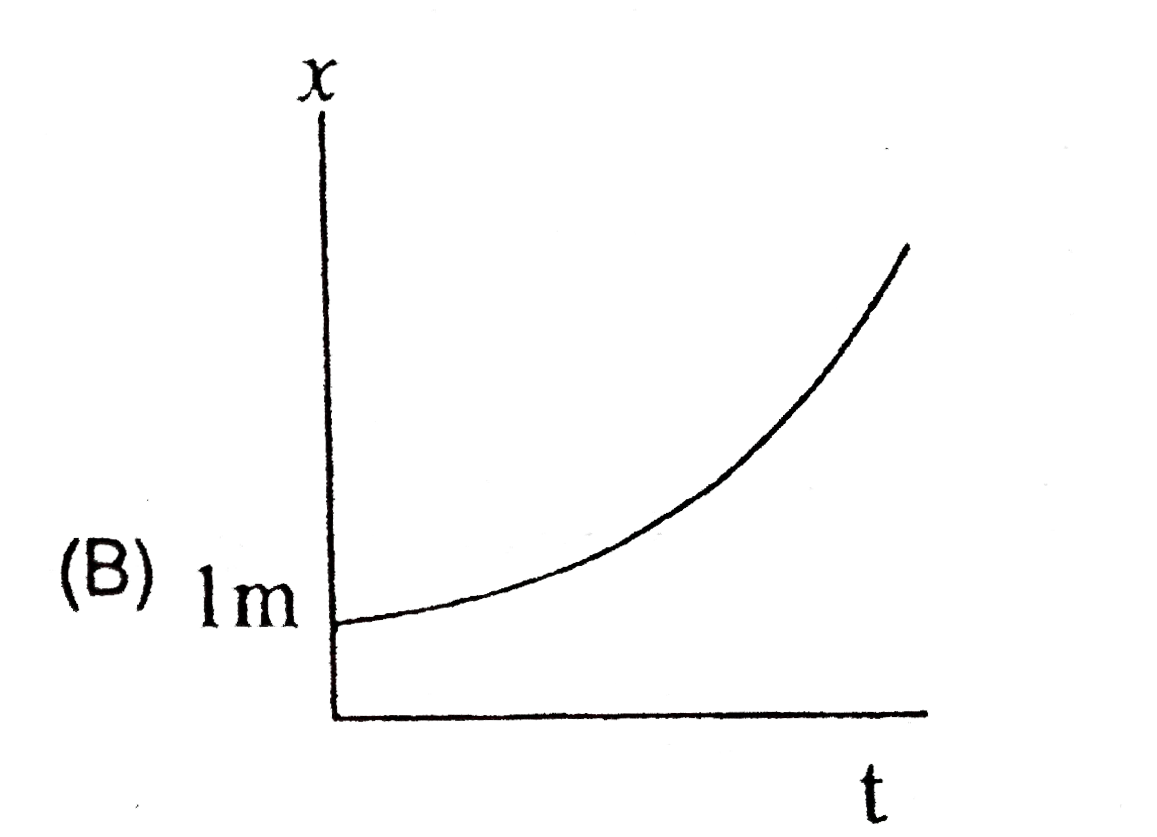 C
C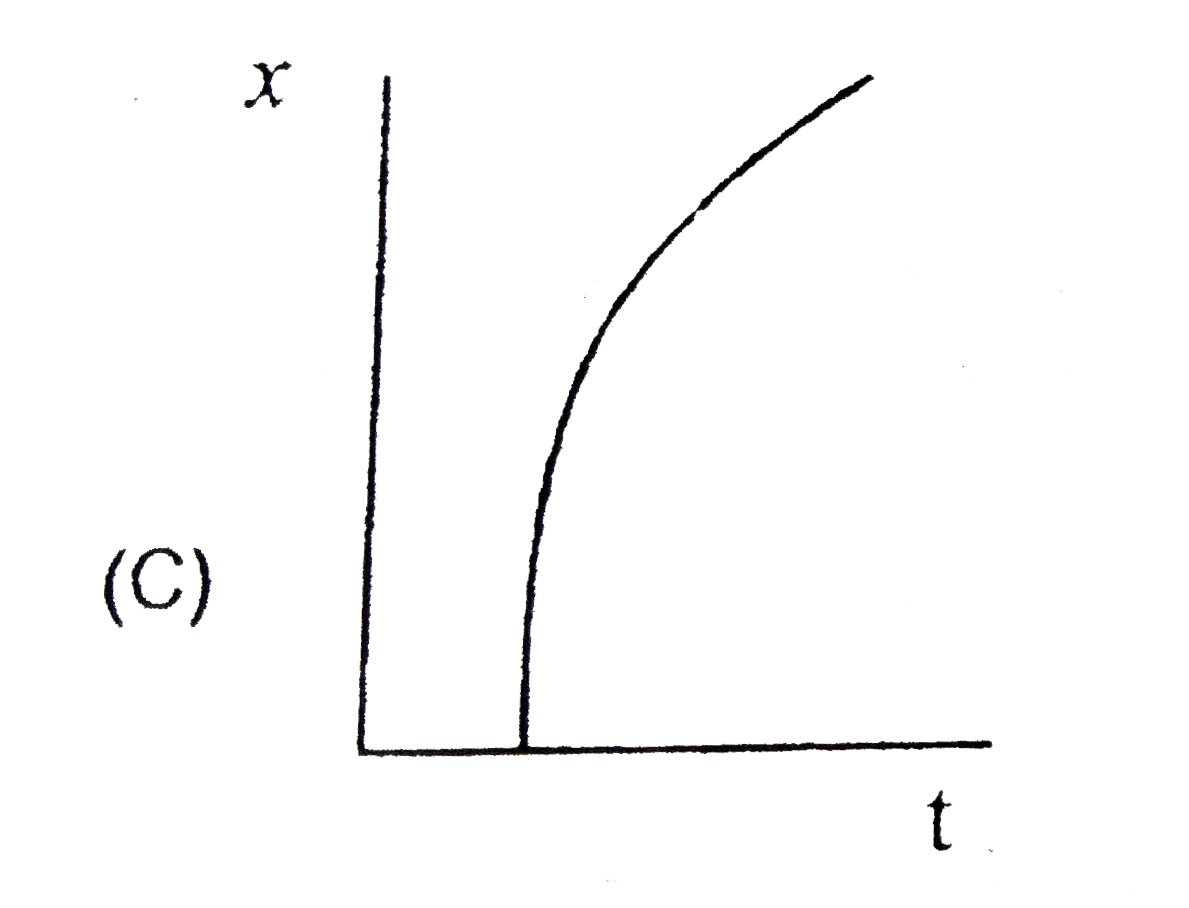 D
D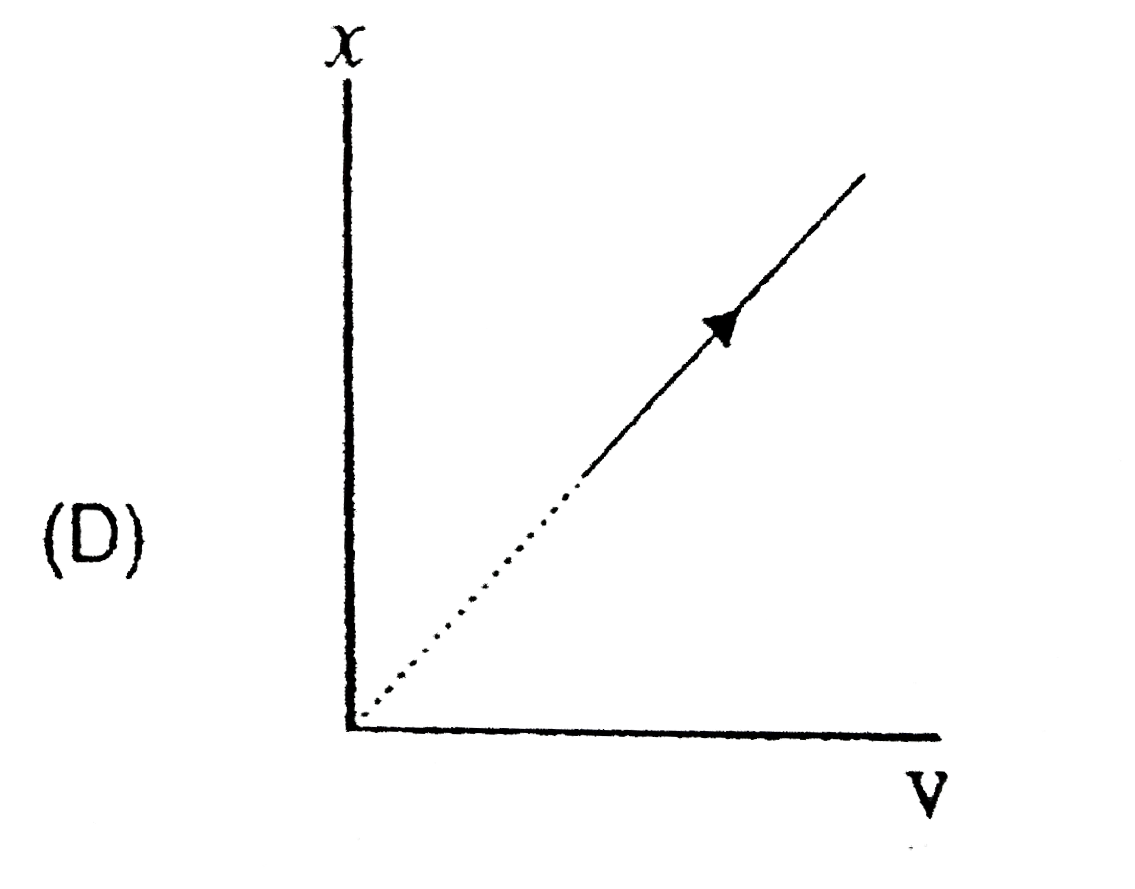
A particle starts motion from rest and moves along a straight line. Its acceleration-time graph is shown. Find out speed of particle at t=2s and at t=3s.

View SolutionThe displacement-time graph for a particle moving in a straight line is as shown in the figure. Determine the velocity of the particle at t=1s,3s and 6s. Sketch the velocity-time graph.
View SolutionA particle is moving in a straight line and its velocity varies with its displacement as v=√4+4s m/s. Assume s=0 at t=0. find the displacement of the particle in metres at t=1s.
View SolutionThe acceleration-displacement graph of a particle moving in a straight line is as shown in figure, initial velocity of particle is zero. Find the velocity of the particle when displacement of the particle is s=12m.
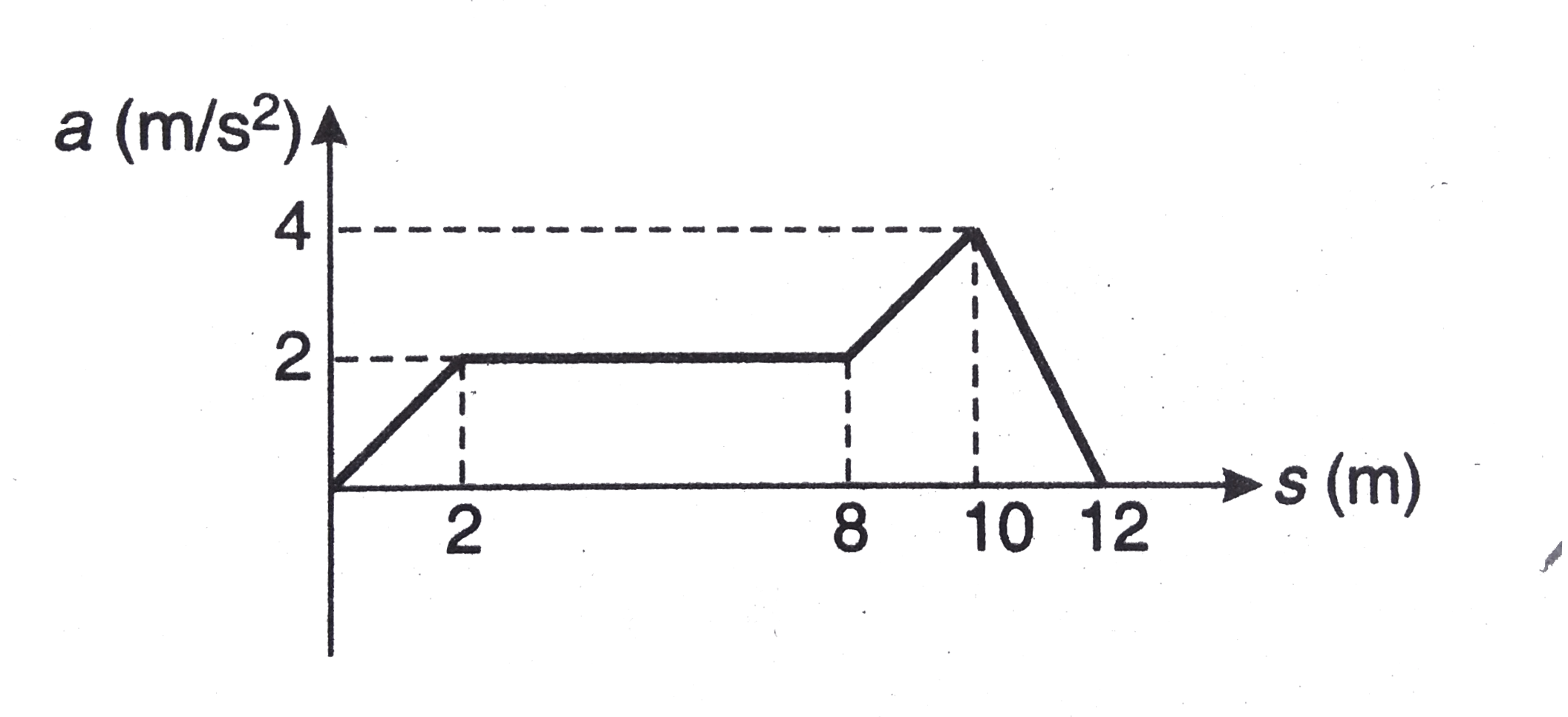
View SolutionA particle is moving in a straight line under constant acceleration alpha. If the intial velocity is v0, sketch the v-t and s-t graphs. (v0>0).
View Solution
