(a) A particle moving with constant acceleration from A to B in a straight line AB has velocities u and v at A and B respectively. Find the velocity of the particle at the midpoint of AB.
(b) If the time taken by the particle to go from A to the midpoint of AB is two times that from the midpoint of AB to B then find the value of v/u.
(a) 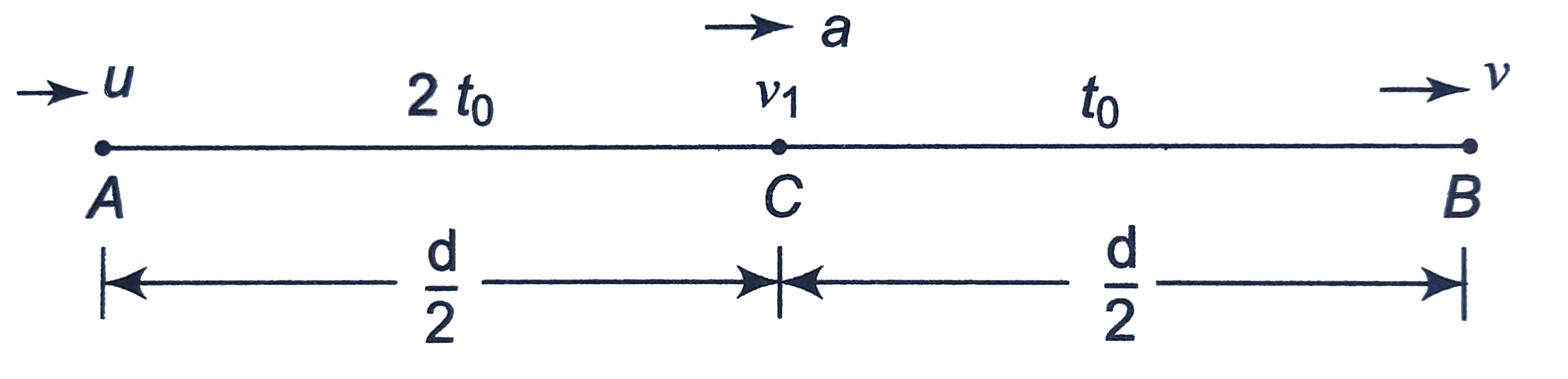
C: Midpoint of AB
v1: Velocity at C
A to C:v21=u2+2a.d2…(i)
A to B:v2=u2+2ad(ii)
From (i) and (ii), we get
v21−u2v2−u2=ad2ad=12
2v21=v2+u2
v1=√v2+u22
(b) A to C:v1=u+a.2t0⇒v1−u=2at0...(i)
Ato B:v=u+a.3t0⇒v−u=3at0...(ii)
Dividing (i) by (ii), we get
v1−uv−u=23⇒3v1−3u=2v−2u
v1=2v+u3⇒√v2+u22=2v+u3
squaring we get
v2+u22=4v2+u2+4vu9
9v2+9u2=8v2+2u2+8uv
v2−8uv+7u2=0
(v−u)(v−7u)=0⇒v=u, not possible
v=7u⇒vu=7
Topper's Solved these Questions
Similar Questions
A point moving with constant acceleration from A to B in the straight line AB has velocities u and v at and B respectively. Find its velocity at C, the mid point of AB. Also show that if the time from A to C is twice that from C to B, then v=7u.
View SolutionA particle moving with uniform acceleration from A to B along a straight line has velcities v1 and v2 at A and B respectively. If C is the mid-point between A and B then determine the velocity of the particle at C .
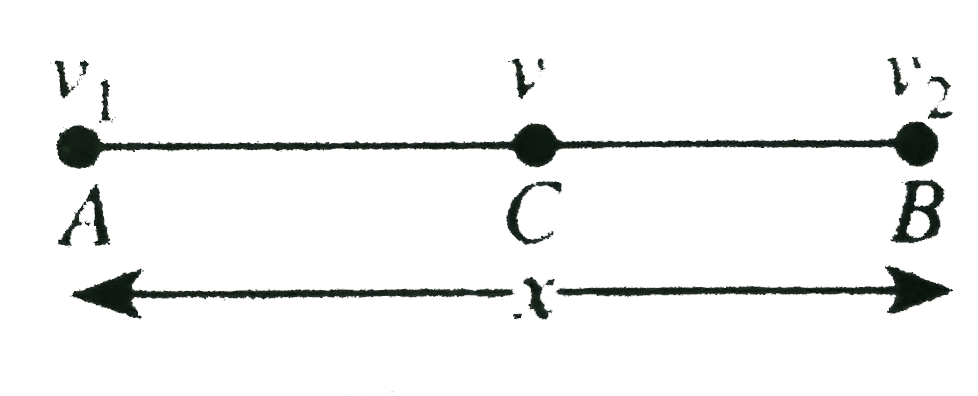 .
.
View Solution
Knowledge Check
A body is moving with constant acceleration, a=5ms2 from A to B in straight line AB. The velocity of particle at A and B are 5 m/s and 15 m/s respectively. The velocity at mid-point of AB is
A√125msB√250msC√550msD√600msA particle is moving with uniform acceleration along a straight line ABC. Its velocity at A and B are 6 m/s and 9 m/s respectively if AB:BC=5:16 then its velocity at C is
A9.6m/s2B12m/sC15m/sD21.5m/sTime taken by the particle to reach from A to B is t. Then the distance AB is equal to
 Aut√3B√3ut2C√3utD2ut
Aut√3B√3ut2C√3utD2ut
A particle is moving with uniform acceleration along a straight line "ABC" .Its velocity at A and B are 6m/s" and "9m/s respectively. If AB:BC=5:16" then its velocity at C is
View Solution.Point M is the midpoint of Seg AB.If AB=8 then find the length of AM.
View SolutionA particle moves along a straight line AB with constant acceleration. Its velocities are u and v at A and B, respectively. Show that its velocity at the mid-point of AB is √u2+v22.
View SolutionIn the time taken by the projectile to reach from A to B is t. Then the distance AB is equal to.
 .
.
View SolutionA particle moves with uniform acceleration along a straight line AB. Its velocities at AandB are 2m/sand14m/s respectively. M is the mid-point of AB. The particle takes t1 seconds to go from A to M and t2 seconds to go from M to B. Then t2:t1 is.
View Solution
