A bar magnet with poles 25cm apart and of strength 14.4amp−m rests with centre on a frictionless pivot. It is held in equilibrium aat an angle of 60∘ with respect to a uniform magnetic field of induction 0.25Wb/m2 , by applying a force F at right angles to its axis at a point 12cm from pivot. Calculate F. What will happen if the force F is removed?
The situation is shown in figure. In equilibrium the torque on M due to B is balanced by torque due to F,i.e.,i.e.,→M×→B=→r×→F
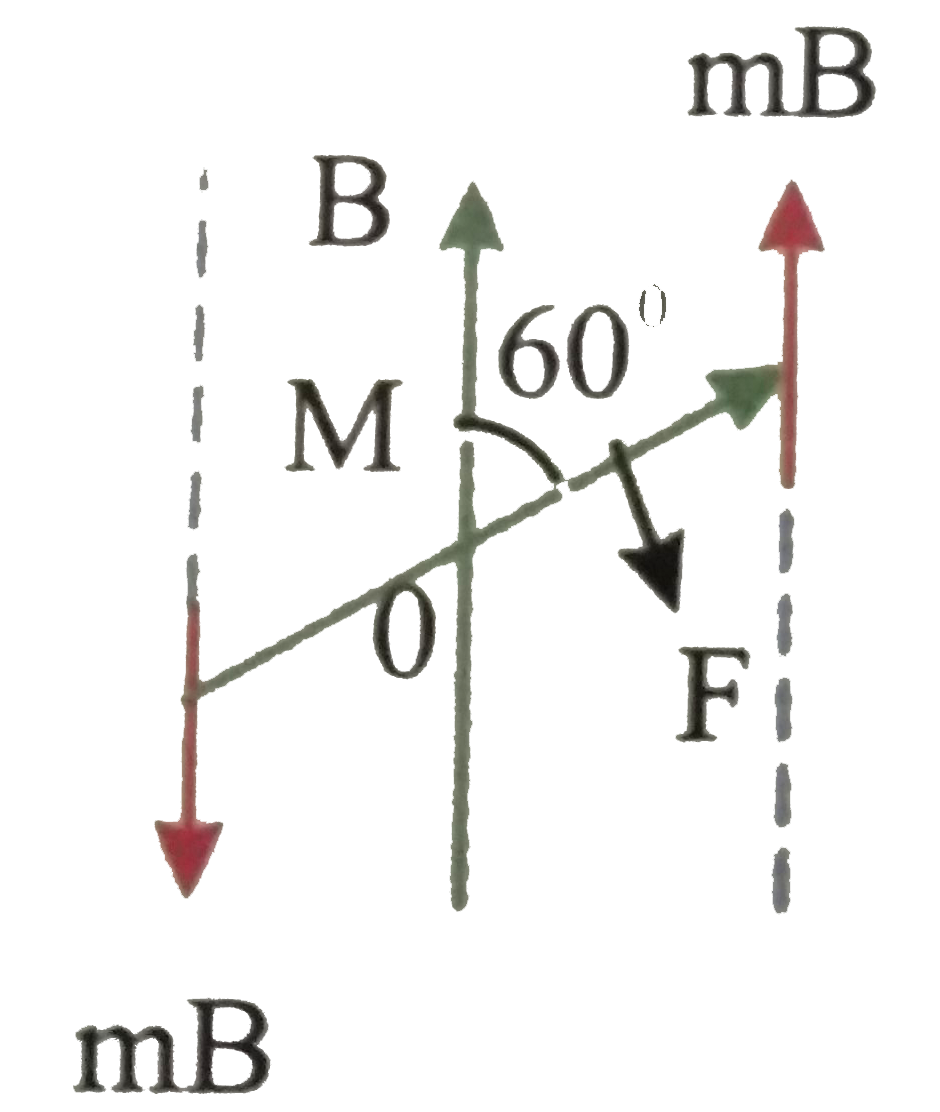
MBsinθ=Frsin90∘orF=(m×2l)Bsinθr
(asM=m×2l), So substituting the given data,
F=14.4×(25×10−2)×0.25(√3/2)10×10−2=7.8N
If the force →F is removed, the torque →M×→B will become unbalanced and under its action the magnet will execute oscillatory motion about the direction of B on its pivot O which will not be simple harmonic as sin theta cancel(=) theta
Topper's Solved these Questions
- MAGNETISMBOOK - NARAYNACHAPTER - MAGNETISMEXERCISE - C.U.Q
73 Videos
- MAGNETISMBOOK - NARAYNACHAPTER - MAGNETISMEXERCISE - C.U.Q (ASSERTION & REASON)
13 Videos
- EXPERIMENTAL PHYSICSBOOK - NARAYNACHAPTER - EXPERIMENTAL PHYSICSEXERCISE - Comprehension type
6 Videos
- MAGNETISM AND MATTERBOOK - NARAYNACHAPTER - MAGNETISM AND MATTEREXERCISE - EXERCISE - 4 (SINGLE ANSWER TYPE QUESTION)
17 Videos
Similar Questions
A bar magnetic with poles 25cm apart and pole strength 14⋅4A.m rests with its centre on a frictionless pivot. It is held in equilibrium at 60∘ to a uniform magnetic field of induction 0⋅25T by applying a force F at right angles to its axis, 10cm from the pivot. Calculate the value of F. What will happen if the force is removed?
View SolutionA bar magnet with poles √3cm apart and pole strength 14⋅4Am rests with its centre on a frictionless pivot. The magnet is held in equilibrium at an angle of 60∘ to a uniform magnetic field of intensity 0⋅20T by applying a force F at right angles to its axis, 0⋅1m from its pivot. What is the value of force F?
View Solution
Knowledge Check
A bar magnet with poles 25.0cm apart and of pole strength 14.4Am rests with its centre on a friction less point. It is held in equilibrium at 60∘ to a uniform magnetic field of induction 0.25T by applying a formce F at right angle to the axis, 12cm from its pivot. The magnitude of the force is
A15√3NB75√3NC3.75√3ND25√3NA bar magnet with poles 25cm apart and pole strength 14.4Am rests with its center on a frictionless pivot. If it is held in equilibrium at 600 to a uniform magnetic field on induction 0.25T by applying a force F at right angles to its axis 10cm from the pivolt, the value of F in newton is (nearly)
A3.9NB7.8NC15.6ND31.2NThe pole strength of a bar magnet is 48A-m and the distance between its poles is 25 cm . The torque by which it can be held at an angle of 30∘ with the uniform magnetic field of strength 0.15 Wb/m2 , will be
A0.90 N-mB1.80 N-mC2.70 N-mD3.6 N-m
A bar magnet with its poles 25 cm apart and of pole strength 24.0A−m rests with its centre on a frictionless pivot. A force F is applied on the magnet at a distance of 12 cm from the pivot, so that it is held in equilibrium at an angle of 30∘ with respect to a magnetic field of induction 0.25 T. The value of force F is
View Solution(a) Derive the experience for the forest acting between two long parallel current carrying conductors. Hence , defined 1 A current .
(b) A bar magnet of dipole moment 3 A m2 . rests with its centre on a frictionless pivot. A force F is applied at right angles to the axis of the magnet , 10 cm from the pivot . It is observed that an external magnetic field of 0.25 T is required to hold the magnet in equilibrium at an angle of 30∘ with the field.
Calculate the value of F.
How will the equilibrium be effected if F is withdrawn?
View SolutionA bar magnet of length 10cm and pole strength 2Am makes an angle 600 with a uniform magnetic field of induction 50T. The couple acting on it is
View SolutionA bar magnet of length 10cm experiences a torque of 0.141N−m in a uniform magnetic field of induction 0.4wb/m2, when it is suspended making an angle 45∘with the field, the pole strength of the magnet is
View SolutionA circular disc of radius 0.2m is placed in a uniform magnetic fied of induction 1π(Wbm2)
in such a way that its axis makes an angle of 60∘ with The magnetic flux linked with the disc is
View Solution
