If the resistance of a circuit having 12 V source is increased by the current drops by 0.5 A. What is the original resistance of circuit
The correct Answer is:B
To solve the problem, we need to find the original resistance of the circuit given the voltage and the change in current when the resistance is increased.
1. Understanding the Problem: We have a circuit with a voltage source of 12 V. When the resistance is increased by 4 Ω, the current decreases by 0.5 A. We need to find the original resistance R of the circuit.
2. Using Ohm's Law: According to Ohm's Law, the relationship between voltage (V), current (I), and resistance (R) is given by:
V=I×R
For our circuit, we can write:
12=I×R(Equation 1)
3. Setting Up the New Condition: When the resistance is increased by 4 Ω, the new resistance becomes R+4 and the new current becomes I−0.5. Applying Ohm's Law again:
12=(I−0.5)(R+4)(Equation 2)
4. Expanding Equation 2:
12=(I−0.5)(R+4)=I×R+4I−0.5R−2
Rearranging gives:
12=IR+4I−0.5R−2
Adding 2 to both sides:
14=IR+4I−0.5R(Equation 3)
5. Substituting Equation 1 into Equation 3: From Equation 1, we know IR=12. Substitute IR in Equation 3:
14=12+4I−0.5R
Simplifying gives:
2=4I−0.5R
Rearranging gives:
0.5R=4I−2
Therefore,
R=8I−4(Equation 4)
6. Substituting Equation 4 into Equation 1: Now we substitute R from Equation 4 back into Equation 1:
12=I(8I−4)
Expanding gives:
12=8I2−4I
Rearranging gives:
8I2−4I−12=0
Dividing the entire equation by 4:
2I2−I−3=0
7. Solving the Quadratic Equation: We can solve this quadratic equation using the quadratic formula:
I=−b±√b2−4ac2a
Here, a=2,b=−1,c=−3:
I=−(−1)±√(−1)2−4×2×(−3)2×2
I=1±√1+244=1±√254=1±54
This gives us two possible values for I:
I=64=1.5orI=−44=−1
Since current cannot be negative, we take I=1.5 A.
8. Finding the Original Resistance: Now substituting I back into Equation 4:
R=8(1.5)−4=12−4=8Ω
Final Answer:
The original resistance of the circuit is R=8Ω.
V=I×R
For our circuit, we can write:
12=I×R(Equation 1)
12=(I−0.5)(R+4)(Equation 2)
12=(I−0.5)(R+4)=I×R+4I−0.5R−2
Rearranging gives:
12=IR+4I−0.5R−2
Adding 2 to both sides:
14=IR+4I−0.5R(Equation 3)
14=12+4I−0.5R
Simplifying gives:
2=4I−0.5R
Rearranging gives:
0.5R=4I−2
Therefore,
R=8I−4(Equation 4)
12=I(8I−4)
Expanding gives:
12=8I2−4I
Rearranging gives:
8I2−4I−12=0
Dividing the entire equation by 4:
2I2−I−3=0
I=−b±√b2−4ac2a
Here, a=2,b=−1,c=−3:
I=−(−1)±√(−1)2−4×2×(−3)2×2
I=1±√1+244=1±√254=1±54
This gives us two possible values for I:
I=64=1.5orI=−44=−1
Since current cannot be negative, we take I=1.5 A.
R=8(1.5)−4=12−4=8Ω
The original resistance of the circuit is R=8Ω.
Topper's Solved these Questions
- CURRENT ELECTRICITYBook:NARAYNAChapter:CURRENT ELECTRICITYExercise:Level 3
Explore 46 Videos
- CURRENT ELECTRICITYBook:NARAYNAChapter:CURRENT ELECTRICITYExercise:NCERT Based Questions
Explore 13 Videos
- CURRENT ELECTRICITYBook:NARAYNAChapter:CURRENT ELECTRICITYExercise:Level 2 C.W
Explore 59 Videos
- COMMUNICATION SYSTEMBook:NARAYNAChapter:COMMUNICATION SYSTEMExercise:Level-II(H.W)
Explore 25 Videos
- DUAL NATURE OF RADIATION AND MATTERBook:NARAYNAChapter:DUAL NATURE OF RADIATION AND MATTERExercise:ASSERTION AND REASON
Explore 15 Videos
Similar Questions
When a Laclanche cell is connected to a 10Ω resistance then a current of 0.25 ampere flows in the circuit. If the resistance is reduced to 4Ω then current becomes 0.5ampere. The internal resistance of galvanometer will be-
View SolutionA battery of emf 10 V and internal resistance 3Ω is connected to a resistor. If the current in the circuit is 0.5 A, what is the resistance of the resistor ? What is the terminal voltage of the battery when the circuit is closed ?
View Solution
Knowledge Check
The current in a simple series circuit is 5 A. When an additional resistance of 2Ω is inserted, the current drops to 4 A. The original resistance of the circuit was
A8ΩB10ΩC1.25ΩD2.5ΩIf the resistance in a circuit with constant voltage increases, the current will:
AincreaseBdecreaseCstay the sameDnot enough informationThe current in a simple series circuit is 5.0 A. When an additional resistance of 2.0Ω is inserted, the current drops to 4.0 A. The original resistance of the circuit in ohms was
A1.25B8C10D20
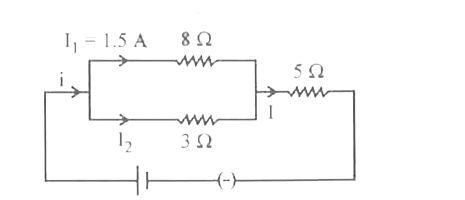
In the given circuit, the current in the 8Ω resistance is 1.5 A. What is the total current (I) flowing in the circuit ?
View SolutionThe current in a simple series circuit is 5.0 amp . When an additional resistance of 2.0 ohms is inserted, the current drops to 4.0 amp . The original resistance of the circuit in ohms was
View SolutionThe current in a circuit containing a battery connected to 2Ω resistance is 0.9A. When a resistance of 7Ω connected to the same battery, the current observed in the circuit is 0.3 A. Then the internal resistance of te battery is
View SolutionThe current in a simple series circuit is 5 A. On addition of 2 2 additional resistance, the current drops to 4 A. The original resistance was:
View SolutionWhat is the electrical energy of a circuit ? If the current flowing from the source is 5 A for duration of 2 s and the resistance of the circuit is 5Ω.
View Solution
