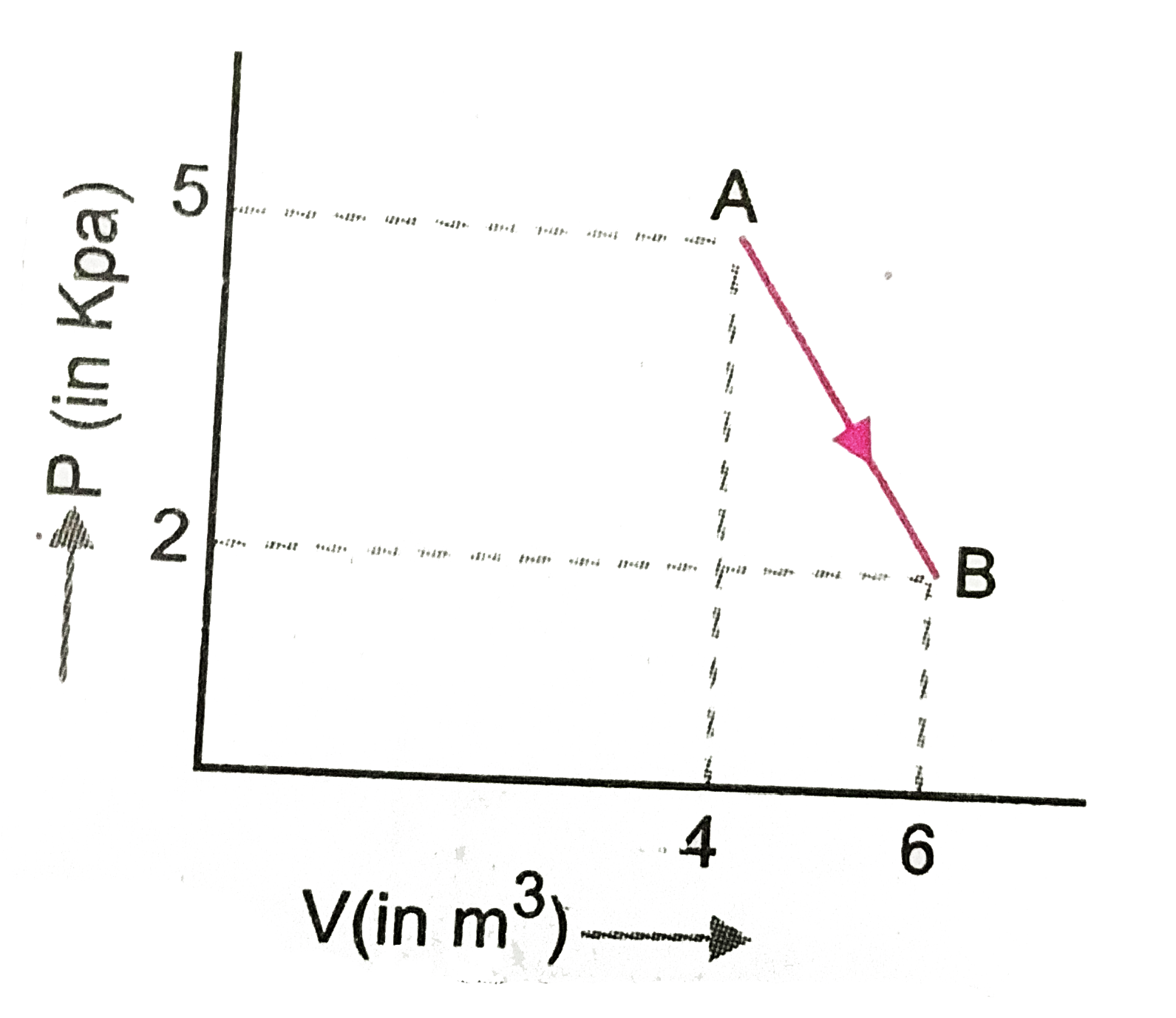
One mole of an ideal diatomic gas undergoes a transition from A to B along a path AB as shown in (figure). The change in internal energy of the gas during the transition is (γ=3/5)
A
−20kJ
B
20J
C
−12kJ
D
20kJ
Video Solution
Text Solution
TA=PAVAnR,TB=PBVBnR
(ΔU)A→B=nCVΔT=n.5R2(TB−TA)
=5nR2(PBVB−PAVAnR)
=52(PBVB−PAVA)
=52(2×6−5×4)×103=−20kJ
|
Topper's Solved these Questions
- NEET PREVIOUS YEARBook:CP SINGHChapter:NEET PREVIOUS YEARExercise:Solved Questions
Explore 64 Videos
- MOTION IN A STRAIGHT LINEBook:CP SINGHChapter:MOTION IN A STRAIGHT LINEExercise:EXERCISES
Explore 128 Videos
- NEWTONS LAWS OF MOTIONBook:CP SINGHChapter:NEWTONS LAWS OF MOTIONExercise:EXERCISES
Explore 84 Videos
Similar Questions
Knowledge Check
One mole of an ideal diatomic gas undergoes a transition from A to B along a path AB as shown in the figure. The change in internal energy of the gas during the transition is
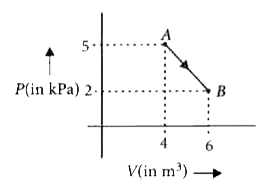 A20 JB−12kJC20 kJD−20kJ
A20 JB−12kJC20 kJD−20kJOne mole of an ideal diatomic gas undergoes a transition from A to B along a path AB as shown in the figure,
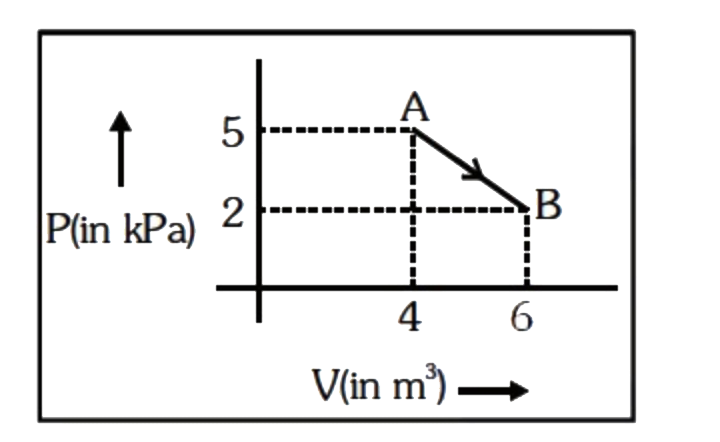
The change in internal energy of the gas during the transition is :A20 kJB−20kJC20 JD−20kJOne mole of an ideal diatomic gas undergoes a transition from A to B along a path AB as shown in (figure). The change in internal energy of the gas during the transition is (γ=3/5)
 A−20KJB20 JC−12KJD20 KJ
A−20KJB20 JC−12KJD20 KJ
One mole of an ideal monoatomic gas undergoes a cyclic process as shown in figure. Then the change in the energy in expanding the gas from a to c along the path abc is:
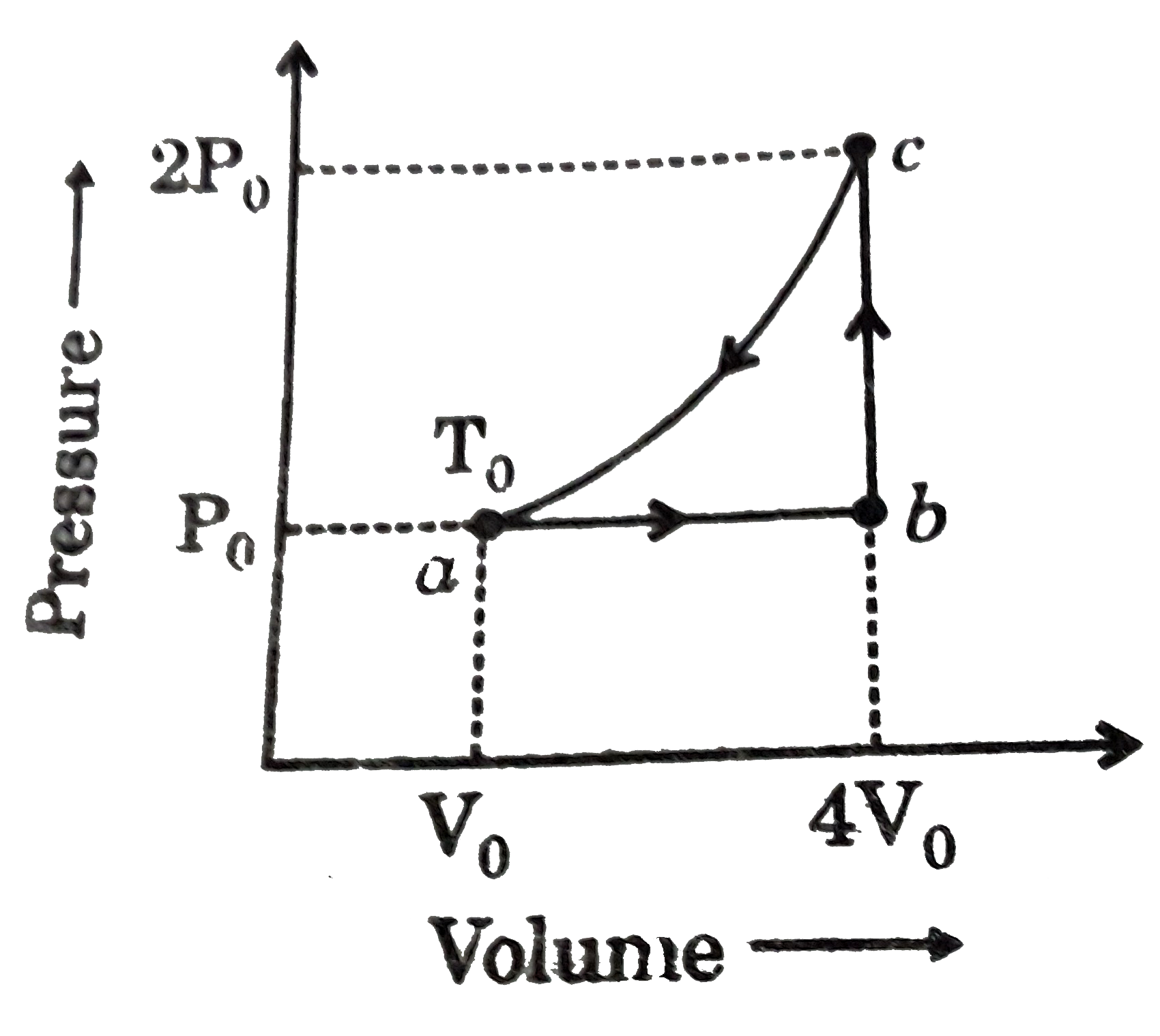
View SolutionFor one mole of a diatomic gas γ is equal to
View SolutionThe internal energy of one mole of a gas is
View SolutionOne mole of an ideal monoatomic gas is caused to go through the cycle shown in figure.Then the change in the internal energy of gas from a to b and b to c is respectively :

View SolutionOne mole of an ideal mono-atomic gas is caused to go through the cycle shown in the figure below. Then the change in the internal enegry in expanding the gas from a to c along the path abc is:
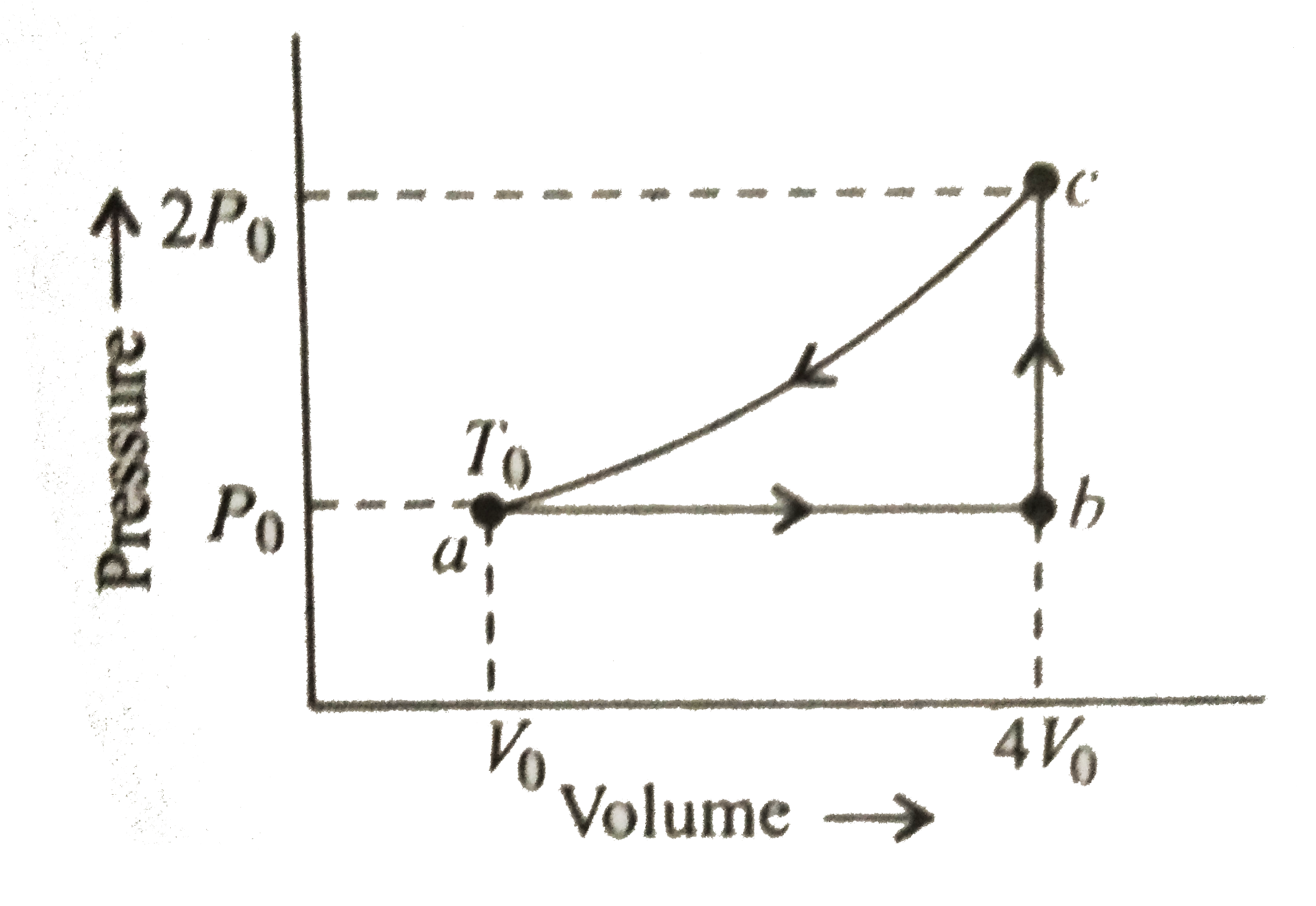
View Solution