A mono-atomic gas is taken along path AB as shown. Calculate change in internal energy of the gas.
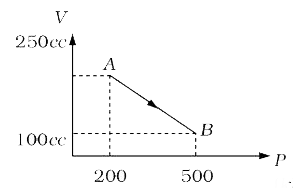
A
B
C
D
zero
Video Solution
Text Solution
The correct Answer is:D
zero
|
Similar Questions
Knowledge Check
In the diagrams (i) to (iv) of variation of volume with changing pressure is shown. A gas is taken along the path ABCD . The change in internal energy of the gas will be
 APositive in all cases (i) to (iv)BPositive in cases (i), (ii) and (iii) but zero in (iv) caseCNegative in cases (i), (ii) and (iii) but zero in (iv) caseDZero in all four cases
APositive in all cases (i) to (iv)BPositive in cases (i), (ii) and (iii) but zero in (iv) caseCNegative in cases (i), (ii) and (iii) but zero in (iv) caseDZero in all four casesIn following figs. Variation of volume by change of pressure is shown in Fig. A gas is taken along the path ABCDA. The change in internal energy of the gas will be:
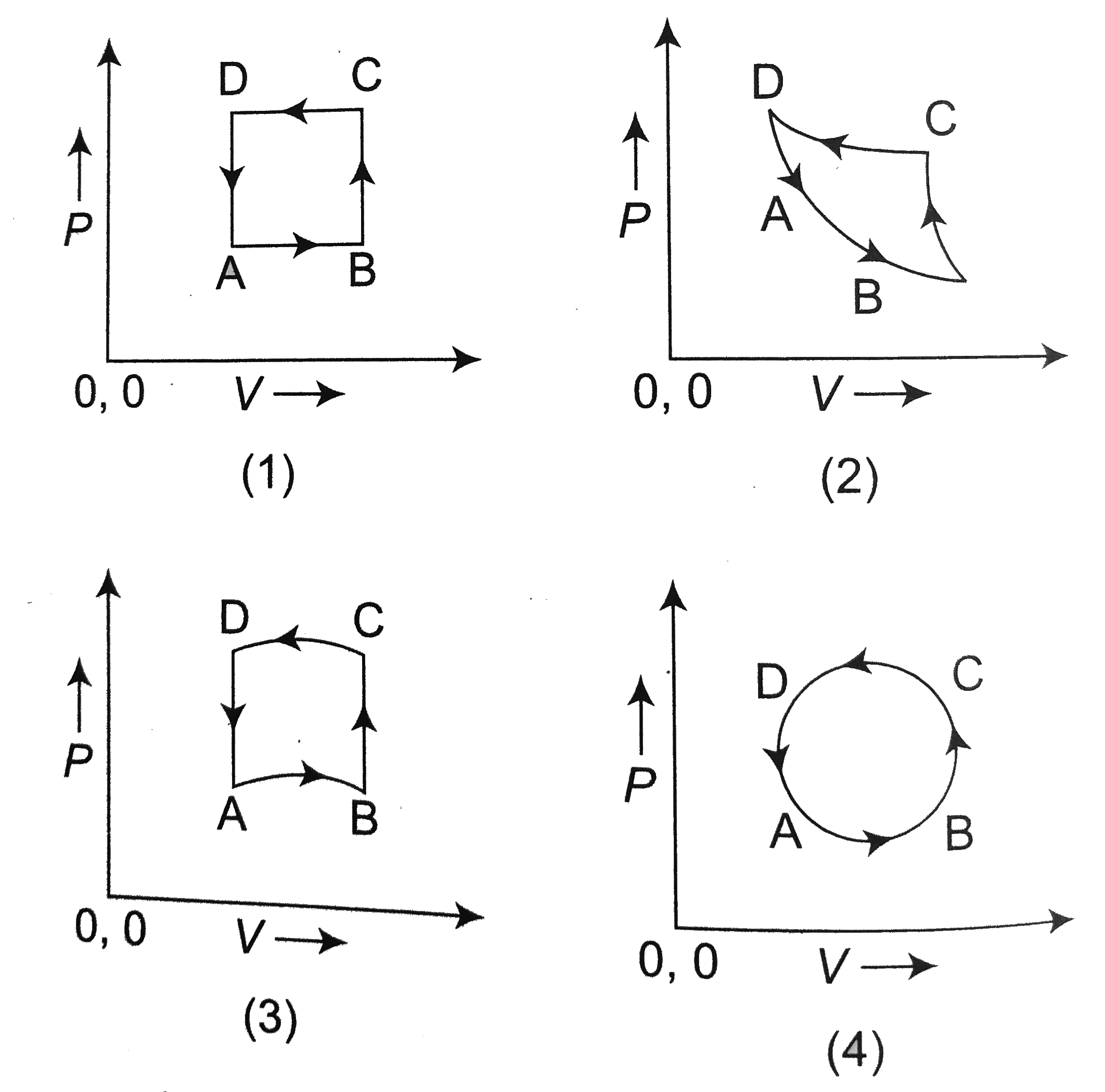 Apositive in all cases from (1)→(4)BPositive in cases (1),(2)and(3) but zero in case (4)CNegative in case (1),(2)and(3) but zero in case (4)Dzero in all the four cases
Apositive in all cases from (1)→(4)BPositive in cases (1),(2)and(3) but zero in case (4)CNegative in case (1),(2)and(3) but zero in case (4)Dzero in all the four casesOne mole of an ideal diatomic gas undergoes a transition from A to B along a path AB as shown in (figure). The change in internal energy of the gas during the transition is (γ=3/5)
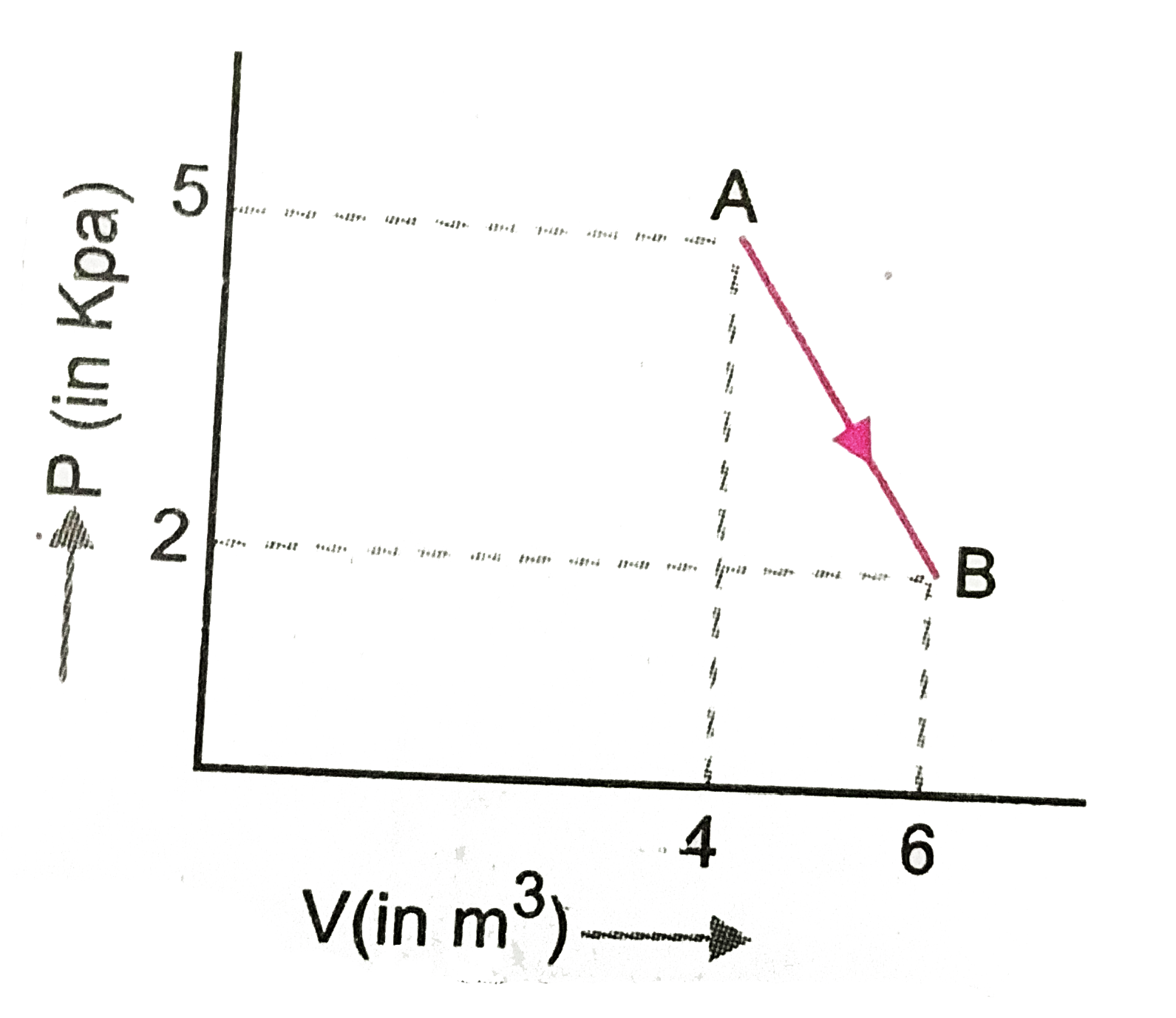 A−20KJB20 JC−12KJD20 KJ
A−20KJB20 JC−12KJD20 KJ
One mole of an ideal diatomic gas undergoes a transition from A to B along a path AB as shown in the figure. The change in internal energy of the gas during the transition is
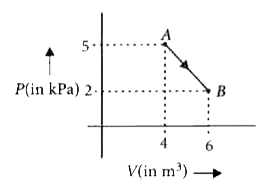
View SolutionOne mole of an ideal diatomic gas undergoes a transition from A to B along a path AB as shown in the figure,
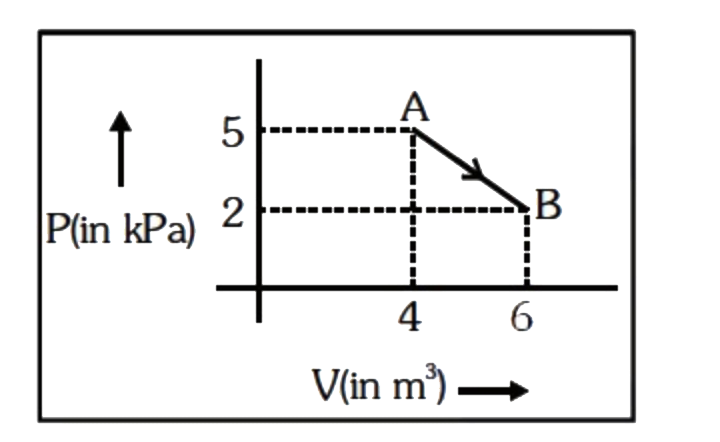
The change in internal energy of the gas during the transition is :
View SolutionOne mole of an ideal diatomic gas undergoes a transition from A to B along a path AB as shown in (figure). The change in internal energy of the gas during the transition is (γ=3/5)

View SolutionIn the above question, change in internal energy of gas is
View SolutionIn the above question, change in internal energy of gas is
View Solution

