If the ratio of coefficient of thermal conductivity of silver and copper is 10:9, then the ratio of the length upto which wax will melt in Ingen Hauze experiment will be
6:10
√10:3
100:81
81:100
The correct Answer is:B
(2) K∝l2
K1K2=(l1l2)2⇒l1l2=√K1K2=√105=√103
Topper's Solved these Questions
Similar Questions
In the Ingen hausz method to compare the thermal conductivities of differnet substances the length upto which wax melted in copper and zinc rods are 9.3cm and 5cm respectively Compare their thermal conductivities .
View SolutionIn Ingen Hauz's experiment ther are two identical rods, (idetical in respect of length and cross-section area) coated uniformly with wax. The material of one rod is copper, whose thermal conductivity, sp. heat capacity and density are 285, 285 and 8930 SI units, respectively and the material of the other is silver whose thermal conductivity sp. heat capacity and density are 419, 235 and 10500 SI units, respectively. Compare the lengths to which wax will melt in the two rods. In which case will the wax melt first?
View Solution
Knowledge Check
One end of a copper rod of length 1.0 m and area of cross-section 10−3 is immersed in boiling water and the other end in ice. If the coefficient of thermal conductivity of copper is 92cal/m−s−.∘C and the latent heat of ice is 8×104cal/kg, then the amount of ice which will melt in one minute is
A9.2×10−3kgB8×10−3kgC6.9×10−3kgD5.4×10−3kgThe ratio of the coefficient of thermal conductivity of two different materials is 5:3. If the thermal resistance of the rod of same thickness resistance of the rods of same thickness of these materials is same, then the ratio of the length of these rods will be
A3:5B5:3C3:4D3:2A cylindrical rod with one end in a steam chamber and the other end in ice results in melting of 0.1 g of ice per second. If the rod is replaced by another with half the length and double the radius of the first and if the thermal conductivity of material of second rod is 1/4 that of first, the rate at which ice melts in g/s will be
A0.4B0.05C0.2D0.1
The ratio of the areas of cross-section of two rods of different materials is 1:2, and the ratio of the thermal conductivities of their materials is 4:3. On keeping equal temperature-difference between the ends of these rods, the rate of conduction of heat are equal. Determine the ratio of the lengths of the rods.
View SolutionThe ratio of thermal conductivity of two rods of different materials is "5:4. The two rods of same area and same thermal resistance will have lengths in the ratio :-
View SolutionTwo conducting rods A and B of same length and cross-sectional area are connected (i) In series (ii) In parallel as shown. In both combination a temperature difference of 100°C is maintained. If thermal conductivity of A is 3 K and that of B is K then the ratio of heat current flowing in parallel combination to that flowing in series combination is
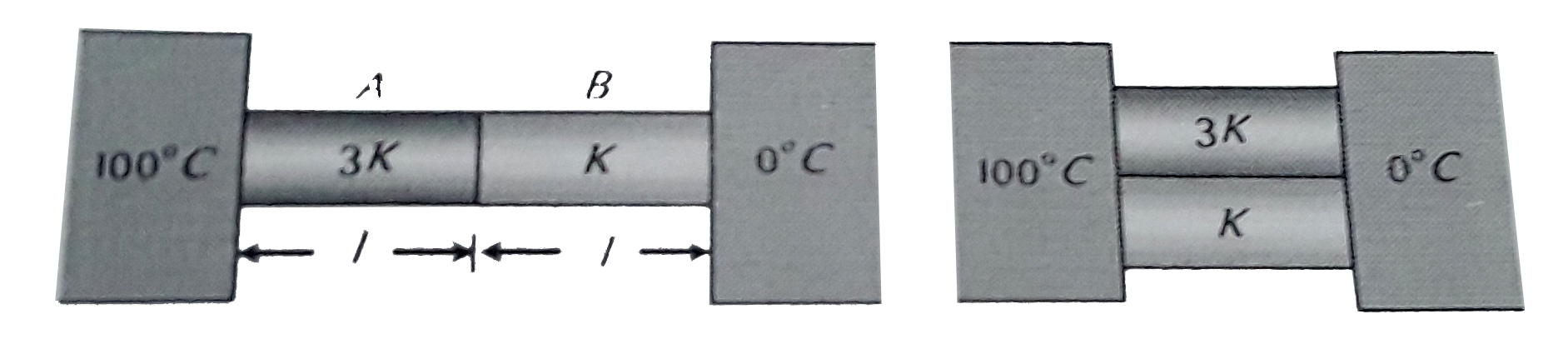
View SolutionAssertion : Greater is the coefficient of thermal conductivity of a material, smaller is the thermal resistance of a rod of that material.
Reason : Thermal resistance is the ratio of temperature difference between the ends of the conductor and rate of flow of heat.
View SolutionThree metal rods of coefficient of thermal conductivities K,2K,3K conducts heats of 3Q,2Q,Q per seconds through unit area then the ratio of temperature gradients .
View Solution
