A particle rotates in U.C.M with tengential velocity 'v' along a ghorizontal circle of diameter 'D' . Total angular displacement of the particle in time 't' is
vt
(vD)−t
vt2D
2vtD
The correct Answer is:D
In U.C.M . v=rω
∴ω=vr=vD2=2vD
∵ω=θtθ=ωt=(2vD)t
Topper's Solved these Questions
- CIRCULAR MOTIONBOOK - MARVEL PUBLICATIONCHAPTER - CIRCULAR MOTIONEXERCISE - TEST YOUR GRASP-1
1 Video
- CIRCULAR MOTIONBOOK - MARVEL PUBLICATIONCHAPTER - CIRCULAR MOTIONEXERCISE - TEST YOUR GRASP-2
1 Video
- ATOMS, MOLECULES AND NUCLEI BOOK - MARVEL PUBLICATIONCHAPTER - ATOMS, MOLECULES AND NUCLEI EXERCISE - TEST YOUR GRASP
30 Videos
- COMMUNICATION SYSTEMS BOOK - MARVEL PUBLICATIONCHAPTER - COMMUNICATION SYSTEMS EXERCISE - TEST YOUR GRASP -20
10 Videos
Similar Questions
A particle moves with an initial velocity v0 and retardation alphav, where alpha is a constant and v is the velocity at any time t. If the particle is at the origin at t=0, find
(a) (i) velocity as a function of time t.
(ii) After how much time the particle stops?
(iii) After how much time velocity decreases by 50%?
(b) (i) Velocity as a function of displacement s.
(ii) The maximum distance covered by the particle.
(c ) Displacement as a function of time t.
View SolutionA particle moves in a circle with constant angular velocity ω about a point P on its circumference. The angular velocity of the particle about the centre C of the circle is
View Solution
Knowledge Check
A particle rotates in U.C.M with tengential velocity 'v' along a ghorizontal circle of diameter 'D' . Total angular displacement of the particle in time 't' is
AvtB(vD)−1Cvt2DD2vtDA particle performing circular motion with its diameter d and velocity v. Then the angular displacement of the particle in time t is
AvtdB2vtdCvt2dDdvtThe tangential velocity of a particle making p rotations along a circle of radius π in t seconds is
A2πpt2B2πp2tCπ2p2tD2π2pt
The figure shows a velocity-time graph of a particle moving along a straight line
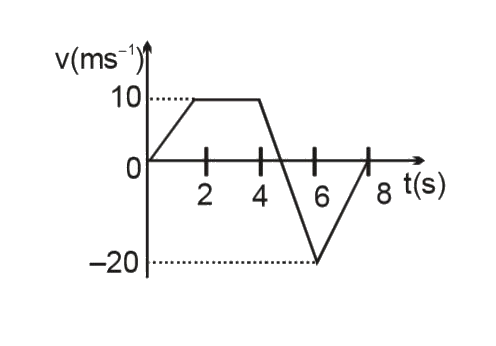
Q. The maximum of displacement of the particle is
View SolutionA particle moves along a circle of radius R with a constant angular speed ω. Its displacement (only magnitude) in time t will be
View SolutionA particle moves along a circle of radius R with a constant angular speed ω . Its displacement (only magnitude) in time t will be
View SolutionA particle moves along x-axis and its acceleration at any time t is a = 2 sin (πt), where t is in seconds and a is in m/s2 . The initial velocity of particle (at time t = 0) is u = 0.
Q. Then the magnitude of displacement (in meters) by the particle from time t = 0 to t = t will be :
View SolutionFor a particle moving along a straight line, its velocity 'v' and displacement 's' are related as v2=cs, here c is a constant. If the displacement of the particle at t=0 is zero, its velocity after 2 sec is:
View Solution
