Three condensers 1μF,2μF and 3μF are connected in series to a p.d of 330 volt. The p.d across the plates of 3μF is.
180 V
300 V
60 V
270 V
The correct Answer is:C
To solve the problem, we need to find the potential difference across the 3 µF capacitor when three capacitors (1 µF, 2 µF, and 3 µF) are connected in series to a potential difference (p.d) of 330 volts.
1. Calculate the Equivalent Capacitance (C_eq):
For capacitors in series, the formula for equivalent capacitance is given by:
1Ceq=1C1+1C2+1C3
Here, C1=1μF, C2=2μF, and C3=3μF.
1Ceq=11+12+13
1Ceq=1+0.5+0.3333=1.8333
Ceq=11.8333≈0.545μF
2. Calculate the Total Charge (Q):
The total charge (Q) on the capacitors can be calculated using the formula:
Q=Ceq×V
Where V=330V.
Q=0.545μF×330V=0.180 mC (or 180μC)
3. Calculate the Potential Difference across the 3 µF Capacitor:
The potential difference (V_3) across a capacitor can be calculated using the formula:
V=QC
For the 3 µF capacitor:
V3=QC3=180μC3μF=60V
Final Answer:
The potential difference across the plates of the 3 µF capacitor is 60 volts.
For capacitors in series, the formula for equivalent capacitance is given by:
1Ceq=1C1+1C2+1C3
Here, C1=1μF, C2=2μF, and C3=3μF.
1Ceq=11+12+13
1Ceq=1+0.5+0.3333=1.8333
Ceq=11.8333≈0.545μF
The total charge (Q) on the capacitors can be calculated using the formula:
Q=Ceq×V
Where V=330V.
Q=0.545μF×330V=0.180 mC (or 180μC)
The potential difference (V_3) across a capacitor can be calculated using the formula:
V=QC
For the 3 µF capacitor:
V3=QC3=180μC3μF=60V
The potential difference across the plates of the 3 µF capacitor is 60 volts.
Topper's Solved these Questions
- CAPACITANCEBOOK - NARAYNACHAPTER - CAPACITANCEEXERCISE - Level-II (C.W)
14 Videos
- CAPACITANCEBOOK - NARAYNACHAPTER - CAPACITANCEEXERCISE - Level-III (C.W)
41 Videos
- CAPACITANCEBOOK - NARAYNACHAPTER - CAPACITANCEEXERCISE - Assertion & Reasoning
16 Videos
- ATOMS BOOK - NARAYNACHAPTER - ATOMS EXERCISE - EXERCISE -4
47 Videos
- COMMUNICATION SYSTEMBOOK - NARAYNACHAPTER - COMMUNICATION SYSTEMEXERCISE - Level-II(H.W)
25 Videos
Similar Questions
Three capacitors 1, 2 and 4μ f are connected in series to a 10 volt source.The charge on the plate of middle capacitor is....
View SolutionThree capacitors of capacitances 3μF,9μF and 18μ F are connected once in series and another time in parallel.The ratio of equivalent capacitance in the two cases,[(CsCp) will be ?
View Solution
Knowledge Check
Three capacitors of 3μF,2μF and 6μF are connected in series. When a battery of 10V is connected to this combination then charge on 3μF capacitor will be.
A5μCB10μCC15μCD20μCTwo capacitors of 2μF and 3μF are connected in series. The potential at point A is 1000 volt and the outer plate of 3μF capacitor is earthed. The potential at point B is
 A300 voltB500 voltC600 voltD400 volt
A300 voltB500 voltC600 voltD400 voltTwo capacitors of capacitances 3μF and 6μF are connected in series and connected to 120V. The potential difference across 3μF is V0 and the charge here is q0. We have
(A) q0=40μC
(B) V0=60V
( C) V0=80V
(D) q0=240μC.AA,C are correctBA,B are correctCB,D are correctDC,D are correct
Three capacitors of a capacitances 3μF,9μF and 18μF are connected initially in series and subsequently in parallel . The ratio of equivalent capacitance in the two cases (CsCp) will be
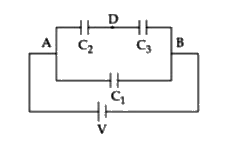
View SolutionThree capacitors C1=2μF,C26μFandC3=12μFare connected as shown in figure. Find the ratio of the charges on capacitors C1,C2andC3 respectively :
View SolutionFour capacitors 4μF,6μF,5μF,3μF are connected in series to a source of 150V. The potential difference in volt, the 5μF capacitor will be
View SolutionThree capacitors of 2μF,3μFand6μF are joined in series and the combination is charged by means of a 24 V battery. The potential difference between the plates of the 6μF capacitor is
View SolutionTwo capacitors of capacites 1μF and CμF are connected in series and the combination is charged to a potential difference of 120V. If the charge on the combination is 80μC, the energy stored in the capacitor C in micro joules is :
View Solution
