meter rod of area of cross section 4mc2 with K=0.5calg−1C−1 is observed that at steady state 360 cal of heat flows per minute The temperature gradient along the rod is .
3∘C/cm
6∘C/an
12∘C/m
20∘C/cm
The correct Answer is:A
To solve the problem, we will use the formula for heat conduction, which relates the heat flow (Q) through a rod to the temperature gradient (dT/dx), the thermal conductivity (K), and the cross-sectional area (A).
1. Identify the Given Values:
- Cross-sectional area, A=4cm2
- Thermal conductivity, K=0.5kcal/g°C
- Heat flow, Q=360kcal/min
2. Convert Heat Flow to Seconds:
- Since the heat flow is given per minute, we need to convert it to per second:
Q=360kcal60s=6kcal/s
3. Use the Heat Conduction Formula:
- The formula for heat conduction is given by:
Q=K⋅A⋅dTdx
Rearranging this formula to find the temperature gradient dTdx:
dTdx=QK⋅A
4. Substitute the Values into the Formula:
- Substitute Q=6kcal/s, K=0.5kcal/g°C, and A=4cm2:
dTdx=6kcal/s0.5kcal/g°C⋅4cm2
5. Calculate the Temperature Gradient:
- First, calculate the denominator:
K⋅A=0.5⋅4=2kcal/g°C
- Now substitute back:
dTdx=6kcal/s2kcal/g°C=3g°C/s
6. Convert Units:
- Since 1g°C/s can be interpreted in terms of temperature gradient, we can express it as:
dTdx=3°C/cm
Final Answer:
The temperature gradient along the rod is 3°C/cm.
- Cross-sectional area, A=4cm2
- Thermal conductivity, K=0.5kcal/g°C
- Heat flow, Q=360kcal/min
- Since the heat flow is given per minute, we need to convert it to per second:
Q=360kcal60s=6kcal/s
- The formula for heat conduction is given by:
Q=K⋅A⋅dTdx
Rearranging this formula to find the temperature gradient dTdx:
dTdx=QK⋅A
- Substitute Q=6kcal/s, K=0.5kcal/g°C, and A=4cm2:
dTdx=6kcal/s0.5kcal/g°C⋅4cm2
- First, calculate the denominator:
K⋅A=0.5⋅4=2kcal/g°C
- Now substitute back:
dTdx=6kcal/s2kcal/g°C=3g°C/s
- Since 1g°C/s can be interpreted in terms of temperature gradient, we can express it as:
dTdx=3°C/cm
The temperature gradient along the rod is 3°C/cm.
Topper's Solved these Questions
- TRANSMISSION OF HEATBook:NARAYNAChapter:TRANSMISSION OF HEATExercise:LEVEL - 2 (C.W)
Explore 1 Video
- TRANSMISSION OF HEATBook:NARAYNAChapter:TRANSMISSION OF HEATExercise:LEVEL - (C.W)
Explore 27 Videos
- TRANSMISSION OF HEATBook:NARAYNAChapter:TRANSMISSION OF HEATExercise:LEVEL -1 (C.W)
Explore 1 Video
- THERMODYNAMICSBook:NARAYNAChapter:THERMODYNAMICSExercise:Exercise
Explore 187 Videos
- UNITS AND MEASUREMENTSBook:NARAYNAChapter:UNITS AND MEASUREMENTSExercise:STATEMENT TYPE QUESTION
Explore 23 Videos
Similar Questions
The ends of a copper rod of length 1m and area of cross-section 1cm2 are maintained at 0∘C and 100∘C. At the centre of the rod there is a source of heat of power 25 W. Calculate the temperature gradient in the two halves of the rod in steady state. Thermal conductivity of copper is 400Wm−1K−1.
View SolutionHeat is flowing through a rod of length 25.0cm having cross-sectional area 8.80cm2. The coefficient of thermal conductivity for the material of the rod is K=9.2×10−2kcals−1m−1.∘C−1. The Temperatures of the ends of the rod are 125∘C and 0∘C in the steady state. Calculate (i) temeprature gradient in the rod (ii) temperature of a point at a distance of 10.0cm from the hot end and (iii) rate of flow of heat.
View Solution
Knowledge Check
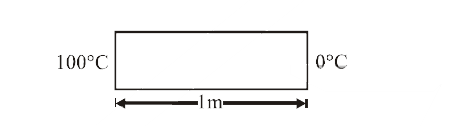
A rod of 1m length and area of cross-section 1cm2 is connected across two heat reservoirs at temperature 100∘C and 0∘C as shown. The heat flow per second through the rod in steady state will be [Thermal conductivity of material of rod =0.09kilocalm−1s−1('∘C)]
 A9×10−4kilocal/secB9kilocal/secC0.09kilocal/secD9×10−6kilocal/sec
A9×10−4kilocal/secB9kilocal/secC0.09kilocal/secD9×10−6kilocal/secIf the coefficient of conductivity of aluminium is 0.5calcm−1s−1.∘C−1, then the other to conductor 10cals−1cm−2 in the steady state, the temperature gradient in aluminium must be
A5∘C/cmB10∘C/cmC20∘C/cmD10.5∘C/cmOne end of a metal rod of length 1.0 m and area of cross section 100cm2 is maintained at . 100∘C. If the other end of the rod is maintained at 0∘C , the quantity of heat transmitted through the rod per minute is (Coefficient of thermal conductivity of material of rod =100W/m−K)
A3×103JB6×103JC9×103JD12×103J
A rod of 1 m length and area of cross-section 1cm2 is connected across two heat reservoirs at temperatures 100∘Cand0∘C as shown. The heat flow per second through the rod in steady state will be [Thermal conductivity of material of rod = 0.09 kilocal m−1s−1(∘C)]
View SolutionOne end of a copper rod of length 0.25m and area of cross-section 10−4m2 is immersed in a liquid boiling at 125∘C whereas the other end is kept cold by dipping It in an ice-cold bath. Find (i) temperature gradient, (ii) the rate of heat transfer (iii) the temperature at a point 0.1m from the higher temperature end. (λ of copper =92cals−1m−1K−1)
View SolutionThe heat is flowing through a rod of length 50 cm and area of cross-section 5cm2 . Its ends are respectively at 25∘C and 125∘C . The coefficient of thermal conductivity of the material of the rod is 0.092kcal/m×s×.∘C . The temperature gradient in the rod is
View Solution1 g of ice at 0∘C is added to 5 g of water at 10∘C. If the latent heat is 80 cal/g, the final temperature of the mixture is
View SolutionThe end of two rods of different materials with their thermal conductivities, area of cross-section and lengths all in the ratio 1:2 are maintained at the same temperature difference. If the rate of flow of heat in the first rod is 4cal/s. Then, in the second rod rate of heat flow in cal/s will be
View Solution