Question

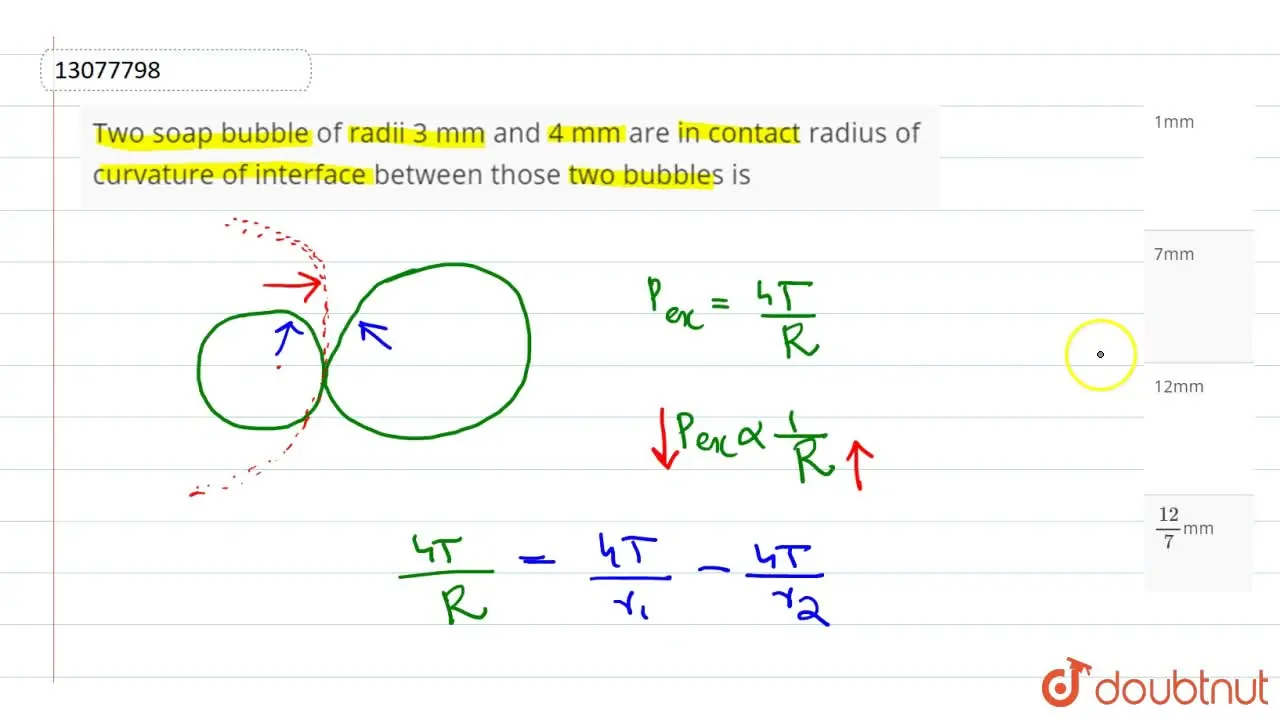
Two soap bubble of radii 3 mm and 4 mm are in contact radius of curvature of interface between those two bubbles is

Options

A. 1mm
B. 7mm
C. 12mm
D. `(12)/(7)`mm

Answer

3

Explanation

To find the radius of curvature of the interface between two soap bubbles with radii 3 mm and 4 mm, we can follow these steps:

1. Identify the Radii of the Bubbles:
- Let \( R_1 = 3 \, \text{mm} \) (radius of the first bubble).
- Let \( R_2 = 4 \, \text{mm} \) (radius of the second bubble).

2. Understand the Concept of Excess Pressure:
- The excess pressure inside a soap bubble is given by the formula:
\( \Delta P = \frac{4T}{R} \)
- Here, \( T \) is the surface tension of the soap solution, and \( R \) is the radius of the bubble.

3. Determine the Excess Pressures:
- For the first bubble (smaller radius):
\( \Delta P_1 = \frac{4T}{R_1} = \frac{4T}{3} \)
- For the second bubble (larger radius):
\( \Delta P_2 = \frac{4T}{R_2} = \frac{4T}{4} = T \)

4. Calculate the Pressure Difference:
- The pressure difference at the interface between the two bubbles is given by:
\( \Delta P = \Delta P_1 - \Delta P_2 = \frac{4T}{3} - T \)
- Simplifying this:
\( \Delta P = \frac{4T}{3} - \frac{3T}{3} = \frac{T}{3} \)

5. Relate the Pressure Difference to the Radius of Curvature:
- The pressure difference across the interface is also related to the radius of curvature \( R \) of the interface:
\( \Delta P = \frac{4T}{R} \)

6. Set the Two Expressions for Pressure Difference Equal:
- Equating the two expressions for pressure difference:
\( \frac{T}{3} = \frac{4T}{R} \)

7. Solve for the Radius of Curvature \( R \):
- Cancel \( T \) from both sides (assuming \( T \neq 0 \)):
\( \frac{1}{3} = \frac{4}{R} \)
- Rearranging gives:
\( R = 12 \, \text{mm} \)

Final Answer:
The radius of curvature of the interface between the two soap bubbles is \( R = 12 \, \text{mm} \).

---