A uniform rod of length L, has a mass per unit length λ and area of cross section A. The elongation in the rod is l due to its own weight, if it suspended form the celing of a room. The Young's modulus of the rod is
3λgL2Al
λgL22Al
2λgLAl
λgL2Al
The correct Answer is:B
To find the Young's modulus of a uniform rod of length L, mass per unit length λ, cross-sectional area A, and elongation l due to its own weight when suspended from the ceiling, we can follow these steps:
1. Understand the Problem:
The rod is uniform, meaning its mass is distributed evenly along its length. The weight of the rod causes it to elongate when suspended.
2. Define the Variables:
- Length of the rod: L
- Mass per unit length: λ
- Cross-sectional area: A
- Elongation due to its weight: l
- Young's modulus: Y
3. Calculate the Force on a Small Element:
Consider a small element of the rod at a distance x from the top, with thickness dx. The weight of the portion of the rod below this element is:
F=λ(L−x)g
where g is the acceleration due to gravity.
4. Determine the Stress and Strain:
The stress σ on the small element is given by:
σ=FA=λ(L−x)gA
The strain ϵ is defined as:
ϵ=dlL
5. Relate Stress and Strain:
According to Hooke's Law:
σ=Yϵ
Substituting the expressions for stress and strain, we have:
λ(L−x)gA=YdlL
6. Integrate to Find Total Elongation:
The total elongation l of the rod can be found by integrating the above equation from x=0 to x=L:
l=∫L0λ(L−x)gAYdx
7. Perform the Integration:
The integral simplifies to:
l=λgAY∫L0(L−x)dx
The integral ∫L0(L−x)dx=(Lx−x22)L0=L2−L22=L22.
8. Substituting Back:
Thus, we have:
l=λgAY⋅L22
9. Rearranging for Young's Modulus:
Rearranging the equation to solve for Y:
Y=λgL22Al
Final Answer:
The Young's modulus Y of the rod is given by:
Y=λgL22Al
The rod is uniform, meaning its mass is distributed evenly along its length. The weight of the rod causes it to elongate when suspended.
- Length of the rod: L
- Mass per unit length: λ
- Cross-sectional area: A
- Elongation due to its weight: l
- Young's modulus: Y
Consider a small element of the rod at a distance x from the top, with thickness dx. The weight of the portion of the rod below this element is:
F=λ(L−x)g
where g is the acceleration due to gravity.
The stress σ on the small element is given by:
σ=FA=λ(L−x)gA
The strain ϵ is defined as:
ϵ=dlL
According to Hooke's Law:
σ=Yϵ
Substituting the expressions for stress and strain, we have:
λ(L−x)gA=YdlL
The total elongation l of the rod can be found by integrating the above equation from x=0 to x=L:
l=∫L0λ(L−x)gAYdx
The integral simplifies to:
l=λgAY∫L0(L−x)dx
The integral ∫L0(L−x)dx=(Lx−x22)L0=L2−L22=L22.
Thus, we have:
l=λgAY⋅L22
Rearranging the equation to solve for Y:
Y=λgL22Al
The Young's modulus Y of the rod is given by:
Y=λgL22Al
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NARAYNACHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - NCERT BASED QUESTIONS
21 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NARAYNACHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - SINGLE ANSWER TYPE
15 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NARAYNACHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - LEVEL-II (C.W)
32 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NARAYNACHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - EXERCISE - III
30 Videos
- MOTION IN A PLANEBOOK - NARAYNACHAPTER - MOTION IN A PLANEEXERCISE - Level-II(H.W)
31 Videos
Similar Questions
A copper rod length L and radius r is suspended from the ceiling by one of its ends. What will be elongation of the rod due to its own weight when and Y are the density and Young's modulus of the copper respectively?
View SolutionA copper rod of length l is suspended from the ceiling by one of its ends. Find:
(a) the elongation Δl of the rod due to its own weight ,
(b) the relative increment of its volume ΔV/V.
View Solution
Knowledge Check
A uniform cylindrical rod of length L and cross-sectional area by forces as shown in figure.
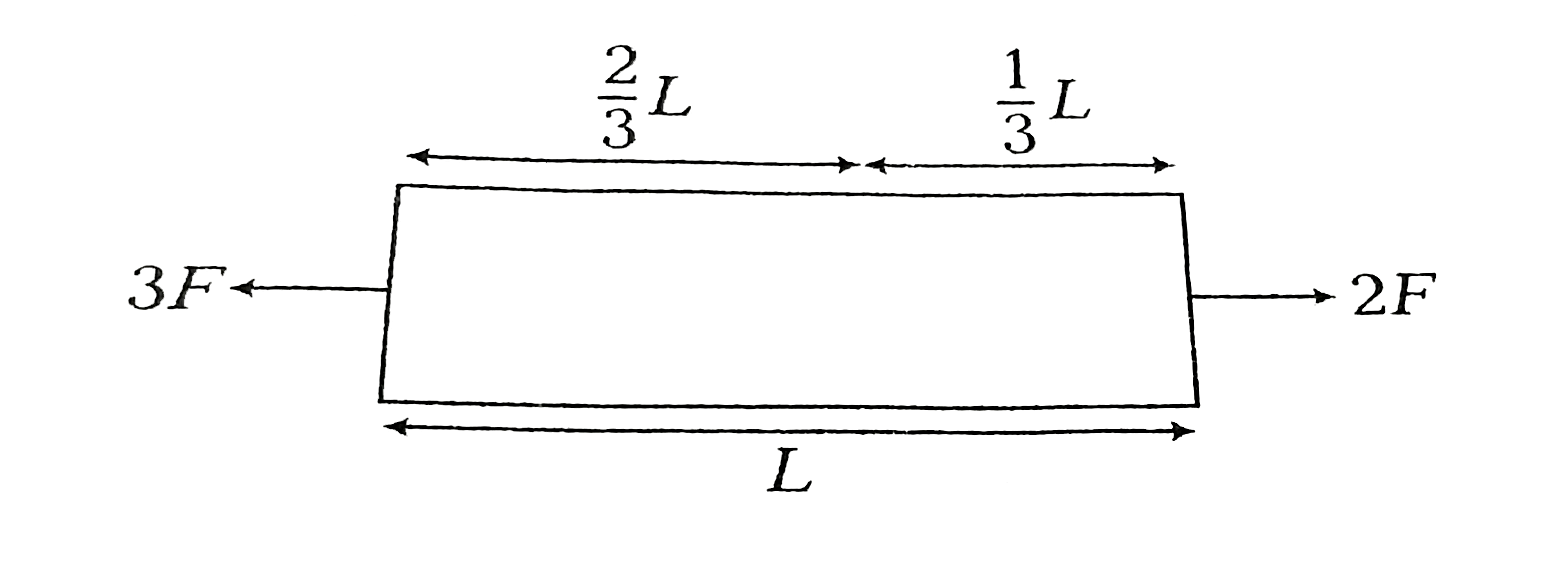
The elongation produced in the rod isA3FL8AYB3FL5AYC8FL3AYD5FL3AYYoung's modulus of a rod is AgL22l for which elongation is λ due to its own weight when suspended from the ceiling. L is the length of the rod and A is constant, which is:
AareaBmass per unit lengthCmass per unit length per unit areaDarea per unit massA uniform thin rod of length l is suspended from one of its ends and is rotated at f rotations per second. The rotational kinetic energy of the rod will be
A23π2f2ml2B43f2ml2C4π2f2ml2Dzero
wire of length I has a ear mass density λ and area of cross-section A and the Young's modulus y is suspended vertically from rigid support. The extension produced in wire due to its own weight
View SolutionA uniform thin rod of length l is suspended from one of its ends and is rotated at f rotations per second. The rotational kinetic energy of the rod will be
View SolutionA uniform rod of mass m. length L, area of cross- secticn A is rotated about an axis passing through one of its ends and perpendicular to its length with constant angular velocity o in a horizontal plane If Y is the Young's modulus of the material of rod, the increase in its length due to rotation of rod is
View SolutionA uniform wire of mass m, length L, area of cross-section A and Young's modulus Y hange from the ceiling. Its elongation under its own weight will be
View SolutionA wire of length L and area of cross-section A, is stretched by a load. The elongation produced in the wire is I. If Y is the Young's modulus of the material of the wire, then the torce corstant of the wire is
View Solution