Two blocks of masses 4 kg and 2 kg are in contact with each other on an inclined plane of inclination 30∘ as shown in the figure . The coefficient of friction between 4 kg mass and the inclined plane is 0.3 , whereas between 2 kg mass and the plane is 0.2 . find the contact force between the blocks .

The acceleration of 4kg mass
If θ=30∘,uk=0.3
a4=g(sinθ−ukcosθ)=10[12−0.3×√32]=2.6ms−2
The acceleration of 2kg mass
a2=10[12−0.2×√32]=3.27ms−2
∴a2<a4
Thus there will be contact force between the block and they move to together If 'a' is the common acceleration,
(m1+m2)a=
(m1+m2)gsinθ−(μ1m1+μ2m2)gcosθ
6a=6×10×12−(0.3×4+0.2×2)×10×√32
6a=30−13.856⇒a=2.7ms−2
For 4kg mass, mg in θ+fcontact−ffriction=ma
4×10×12+fc−0.3×10×10×√32=4×2.7
fc=10.8+10.4−20⇒fc=1.2N .
Topper's Solved these Questions
- NEWTONS LAWS OF MOTIONBOOK - NARAYNACHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - C.U.Q
73 Videos
- NEWTONS LAWS OF MOTIONBOOK - NARAYNACHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - LEVEL -I (C.W)
47 Videos
- MOTION IN A STRAIGHT LINEBOOK - NARAYNACHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - Level 2 H.W
29 Videos
- OSCILLATIONSBOOK - NARAYNACHAPTER - OSCILLATIONSEXERCISE - EXERCISE - IV
41 Videos
Similar Questions
Two block of masses 2kg and 4kg are released from rest over a smooth inclined place of inclination 30∘ as shown in figure what is the normal force between the two blocks?
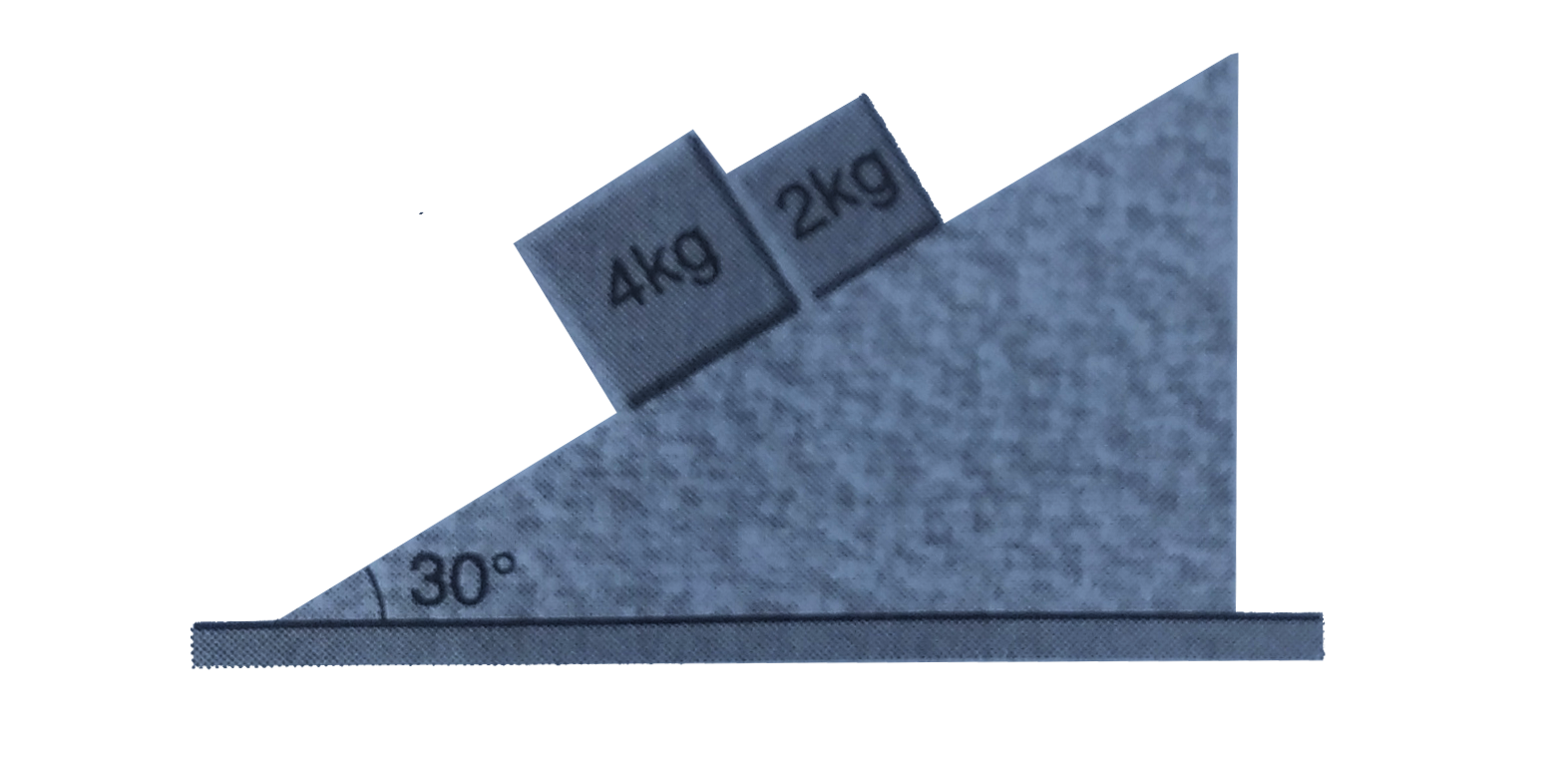
View SolutionTwo masses 6 kg and 4 kg are connected by a flexible inextensible string rest on an inclined plane inclined at 60∘ with the horizontal as shown in figure. The coefficient of friction between the plane and the 6kg mass is 0.1 and the between the plane and the 4 kg massis 0.6 .
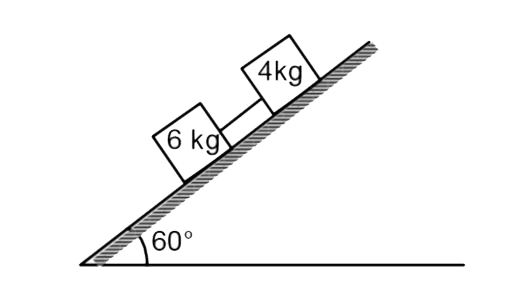
Find the tension in the connecting string.
View Solution
Knowledge Check
A block of mass m slides down an inclined plane of inclination θ with uniform speed The coefficient of friction between the block and the plane is μ. The contact force between the block and the plane is .
Amgsinθ√1+μ2B√(mgsinθ)2+(μmgcosθ)2CmgsinθDmgA block of mass m slides down an inclined plane of inclination θ with uniform speed. The coefficient of friction between the block and the plane is μ. The contact force between the block and the plane is
AmgBmgsinθ√1+μ2CmgsinθD√(mgsinθ)2+(μmgcosθ)2In the arrangement shown in figure coefficient of friction between 5kg block and incline plane is μ=0.5. Friction force acting on the 5kg block is
 A20NB15NC10ND5N
A20NB15NC10ND5N
In Fig. 6-52, two blocks, in contact, slide down an inclined plane AC of inclination 30∘ . The coefficient of kinetic friction between the 2.0 kg block and the incline is u1=0.20 and that between the 4.0 kg block and the incline is μ2=0.30. Find the magnitude of the acceleration.

View SolutionA block of mass m=4kg is placed over a rough inclined plane as shown in fig . The coefficient of friction between the block and the plane μ=0.5 A force F=10N is applied on the block at an angle of 30∘. Find the contact force between the block and the plane.
k

View SolutionA block of mass m=2kg is resting on a inclined plane of inclination 30∘ as shown in figure The coefficient of friction between the block and the plane is μ=0.5 what minimum force F (in newton) should be applied perpendicular to the plane on the block so that the does not slip on the plane?

View SolutionA block of mass m = 4 kg is placed over a rough inclined plane as shown in figure. The coefficient of friction between the block and the plane is u=0.5. A force F= 10 N is applied on the block at an angle of 30°. The friction force between the block and wedge is

View SolutionA system is shown in figure. Blocks 2 & 3 kg are at rest then co-efficient of friction between 2 kg & incline is
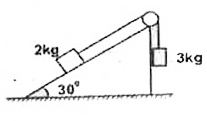
View Solution
