A block of mass 10kg is pushed by a force F on a horizontal rough plane is moving with acceleration 5ms−2 When force is doubled its acceleration becomes 18ms−2 Find the coefficient of friction between the block and rough horizontal plane (g=10ms−2) .
To solve the problem, we will follow these steps:
Step 1: Identify the given data
- Mass of the block, m=10kg
- Initial acceleration, a1=5m/s2
- Final acceleration when force is doubled, a2=18m/s2
- Acceleration due to gravity, g=10m/s2
Step 2: Write the equations of motion
Using Newton's second law, we can write the equations for both scenarios.
1. For the initial scenario where the force is F:
F−fk=m⋅a1
where fk is the frictional force, which can be expressed as:
fk=μk⋅N=μk⋅m⋅g
Therefore, the equation becomes:
F−μk⋅m⋅g=m⋅a1
Substituting the values:
F−μk⋅10⋅10=10⋅5
Simplifying:
F−100μk=50(Equation 1)
2. For the second scenario where the force is doubled (2F):
2F−fk=m⋅a2
This gives us:
2F−μk⋅m⋅g=m⋅a2
Substituting the values:
2F−μk⋅10⋅10=10⋅18
Simplifying:
2F−100μk=180(Equation 2)
Step 3: Solve the equations
Now we have two equations:
1. F−100μk=50 (Equation 1)
2. 2F−100μk=180 (Equation 2)
From Equation 1, we can express F:
F=50+100μk
Substituting this expression for F into Equation 2:
2(50+100μk)−100μk=180
Expanding:
100+200μk−100μk=180
This simplifies to:
100+100μk=180
Subtracting 100 from both sides:
100μk=80
Dividing by 100:
μk=0.8
Final Answer
The coefficient of friction between the block and the rough horizontal plane is μk=0.8.
---
- Mass of the block, m=10kg
- Initial acceleration, a1=5m/s2
- Final acceleration when force is doubled, a2=18m/s2
- Acceleration due to gravity, g=10m/s2
Using Newton's second law, we can write the equations for both scenarios.
F−fk=m⋅a1
where fk is the frictional force, which can be expressed as:
fk=μk⋅N=μk⋅m⋅g
Therefore, the equation becomes:
F−μk⋅m⋅g=m⋅a1
Substituting the values:
F−μk⋅10⋅10=10⋅5
Simplifying:
F−100μk=50(Equation 1)
2F−fk=m⋅a2
This gives us:
2F−μk⋅m⋅g=m⋅a2
Substituting the values:
2F−μk⋅10⋅10=10⋅18
Simplifying:
2F−100μk=180(Equation 2)
Now we have two equations:
1. F−100μk=50 (Equation 1)
2. 2F−100μk=180 (Equation 2)
F=50+100μk
2(50+100μk)−100μk=180
Expanding:
100+200μk−100μk=180
This simplifies to:
100+100μk=180
Subtracting 100 from both sides:
100μk=80
Dividing by 100:
μk=0.8
The coefficient of friction between the block and the rough horizontal plane is μk=0.8.
Topper's Solved these Questions
- NEWTONS LAWS OF MOTIONBOOK - NARAYNACHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - C.U.Q
73 Videos
- NEWTONS LAWS OF MOTIONBOOK - NARAYNACHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - LEVEL -I (C.W)
47 Videos
- MOTION IN A STRAIGHT LINEBOOK - NARAYNACHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - Level 2 H.W
29 Videos
- OSCILLATIONSBOOK - NARAYNACHAPTER - OSCILLATIONSEXERCISE - EXERCISE - IV
41 Videos
Similar Questions
Knowledge Check
A block of weight 100N is pushed by a force F on a horizantal rough plane moving with an acceleration 1m/s2 when force is doubled its acceleration becomes 10m/s2. The coefficient of friction is (10m/s−2)
A0.4B0.6C0.5D0.8A block of weight 100N is pushed by a force F on a horizontal rough plane moving with an acceleration 1m/s2, when force is doubled its acceleration becomes 10m/s−2. The coefficient of friction is (g=10m/s−2)
A0.4B0.6C0.5D0.8A body of mass 10 kg lies on a rough horizontal surface. When a horizontal force of F newtons acts on it, it gets an acceleration of 5m/s2. And when the horizontal force is doubled, it gets an acceleration of 18m/s2. The coefficient of friction between the body and the horizontal surface is
A0.2B0.4C0.6D0.8
Find the force required to move a train of mass 105 kg up an incline of 1 in 50 with an acceleration of 2ms−2. Coefficient of friction between the train and rails is 0.005. Take g=102.
View SolutionA body of mass 8kg lies on a rough horizontal table. It is observed that a certain horizontal force gives the body an acceleration of the body is 16ms−2 . The coefficient of friction is.
View SolutionA block of mass 1 kg is placed on a truck which accelerates with acceleration 5ms−2. The coefficient of static friction between the block and truck is 0.6. The frictional force acting on the block is
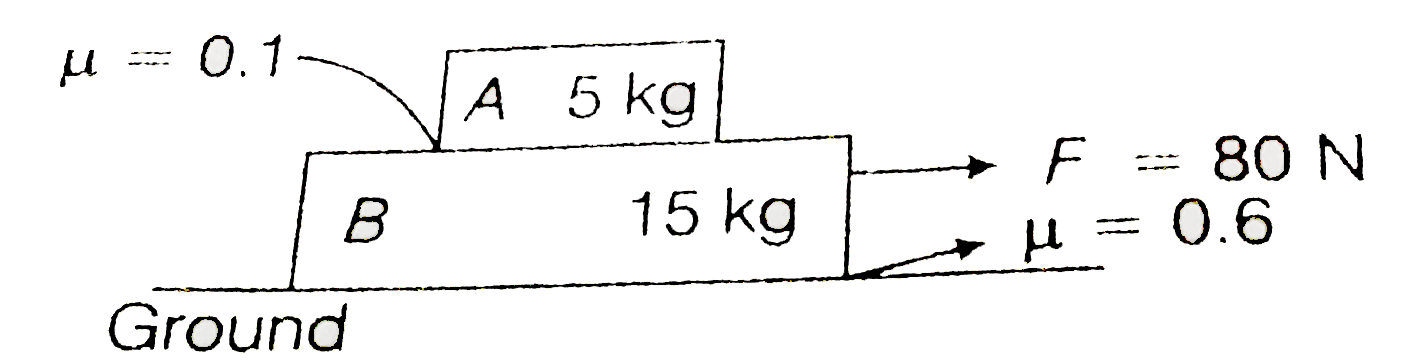
View SolutionFind the value of friction forces between the blocks A and B and between B and ground. ( Take , g=10ms−2)
View SolutionA block of mass m=5kg is resting on a rough horizontal surface for which the coefficient of friction is 0.2 . When a force F=40N is applied, the acceleration of the block will be (g=10m/s2) .

View Solution