Rain is falling vertically downward with velocity A man is moving horizontally with velocity , the velocity of rain with respect to man is
at an angle with horizontal
at an angle with vertical
at an angle with vertical
Both and
The correct Answer is:D
To find the velocity of rain with respect to the man, we can follow these steps:
Step 1: Understand the given velocities
- The rain is falling vertically downward with a velocity of 4m/s.
- The man is moving horizontally with a velocity of 3m/s.
Step 2: Represent the velocities in vector form
- The velocity of the rain can be represented as:
→Vrain=0^i−4^jm/s(downward direction)
- The velocity of the man can be represented as:
→Vman=3^i+0^jm/s(horizontal direction)
Step 3: Calculate the relative velocity of rain with respect to the man
- The relative velocity of the rain with respect to the man is given by:
→Vrain, man=→Vrain−→Vman
- Substituting the values:
→Vrain, man=(0^i−4^j)−(3^i+0^j)
=−3^i−4^jm/s
Step 4: Find the magnitude of the relative velocity
- The magnitude of the relative velocity can be calculated using the Pythagorean theorem:
|→Vrain, man|=√(−3)2+(−4)2
=√9+16=√25=5m/s
Step 5: Find the direction of the relative velocity
- The direction can be found using the tangent function:
tan(θ)=oppositeadjacent=−4−3=43
- Therefore, the angle θ with respect to the horizontal can be calculated as:
θ=tan−1(43)
Final Result
- The velocity of the rain with respect to the man is:
5m/s at an angle of tan−1(43) with the horizontal.
- The rain is falling vertically downward with a velocity of 4m/s.
- The man is moving horizontally with a velocity of 3m/s.
- The velocity of the rain can be represented as:
→Vrain=0^i−4^jm/s(downward direction)
- The velocity of the man can be represented as:
→Vman=3^i+0^jm/s(horizontal direction)
- The relative velocity of the rain with respect to the man is given by:
→Vrain, man=→Vrain−→Vman
- Substituting the values:
→Vrain, man=(0^i−4^j)−(3^i+0^j)
=−3^i−4^jm/s
- The magnitude of the relative velocity can be calculated using the Pythagorean theorem:
|→Vrain, man|=√(−3)2+(−4)2
=√9+16=√25=5m/s
- The direction can be found using the tangent function:
tan(θ)=oppositeadjacent=−4−3=43
- Therefore, the angle θ with respect to the horizontal can be calculated as:
θ=tan−1(43)
- The velocity of the rain with respect to the man is:
5m/s at an angle of tan−1(43) with the horizontal.
Topper's Solved these Questions
Similar Questions
The rain is falling vertically downward with velocity 6m/s and a man is moving horizontally with velocity 8m/s. Find the velocity of rain with respect to the man.
View Solution(A): Rain is falling vertically downwards with velocity 6 km/hr. A man walks with a velocity of 8 km/hr. Relative velocity of rain w.r.t. the man is 10km/hr.
(R): Relative velocity is the ratio of two velocities.
View Solution
Knowledge Check
Rain is falling vertically with velocity 10ms and a man is moving horizontally with velocity 6ms . The angle with which umbrella should be hold (with vertical) by a man to avoid getting wet is
Atan−1(53)Btan−1(23)Ctan−1(35)Dtan−1(32)An object is falling vertically downwards with velocity 10m/s.In terms ofˆiandˆj find the image velocity. M
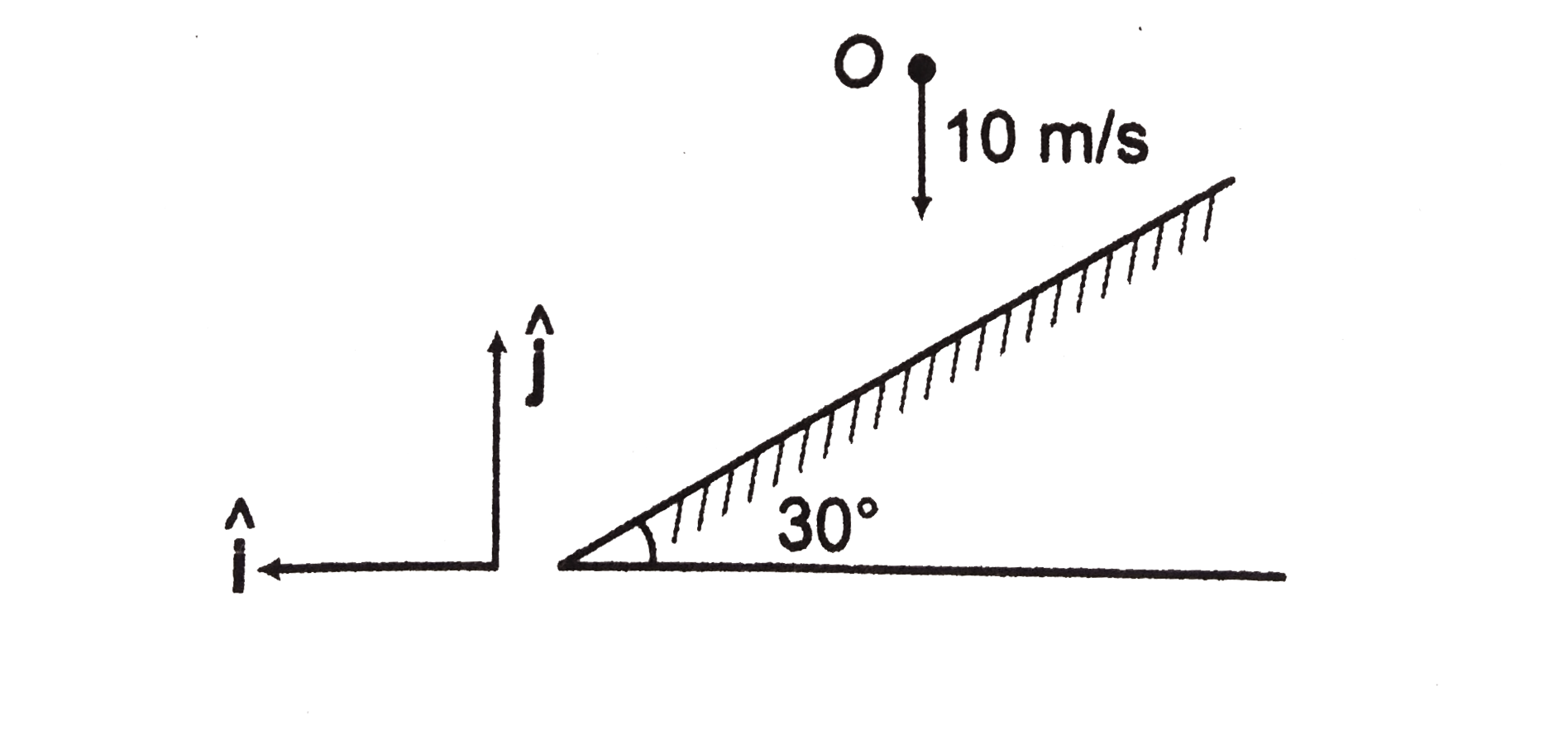 A(5√3ˆi−5ˆj)m/sB(5√3ˆi+5ˆj)m/sC(√3ˆi+2ˆj)m/sDnone
A(5√3ˆi−5ˆj)m/sB(5√3ˆi+5ˆj)m/sC(√3ˆi+2ˆj)m/sDnoneA car is moving on horizontal ground with a velocity vm s−1 with respect to earth. The driver of the car observes the rain to be falling vertically downwards with velocity 2vm s−1 . What is the velocity of the rain with respect to the driver ?
A3vBv√3Cv√5Dnone of these
Statement-1 : Rain is falling vertically downwards with velocity 6 km/h. A man walks with a velocity of 8 km/h. Relative velocity of rain w.r.t. the man is 10 km/h. Statement-2 : Relative velocity is the ratio of two velocities
View SolutionRain is falling vertically downwards with a velocity of 2m//s. A person is runing to the North with a velocity of 2√3m/s. Find out the velocity and direction of rain as appeared to the person.
View SolutionA man is walking due west with a velocity 3km/h and rain appears to be falling vertically with a velocity 4km/h. Find the velocity of rain with respect to the ground.
View SolutionRain droplets are falling in vertically downward direction with velocity 5m/s. A cyclist is moving in northward direction with velocity 10 m/s. The rain droplets will appear to the cyclist to be coming from
View SolutionA man standing on a inclined plain observes rain is falling vertically. When he starts moving down the inclined plain with velocity v=6m/s observes rain hitting him horizontally.

The velocity of rain with respect to the man when he is moving down is
View Solution