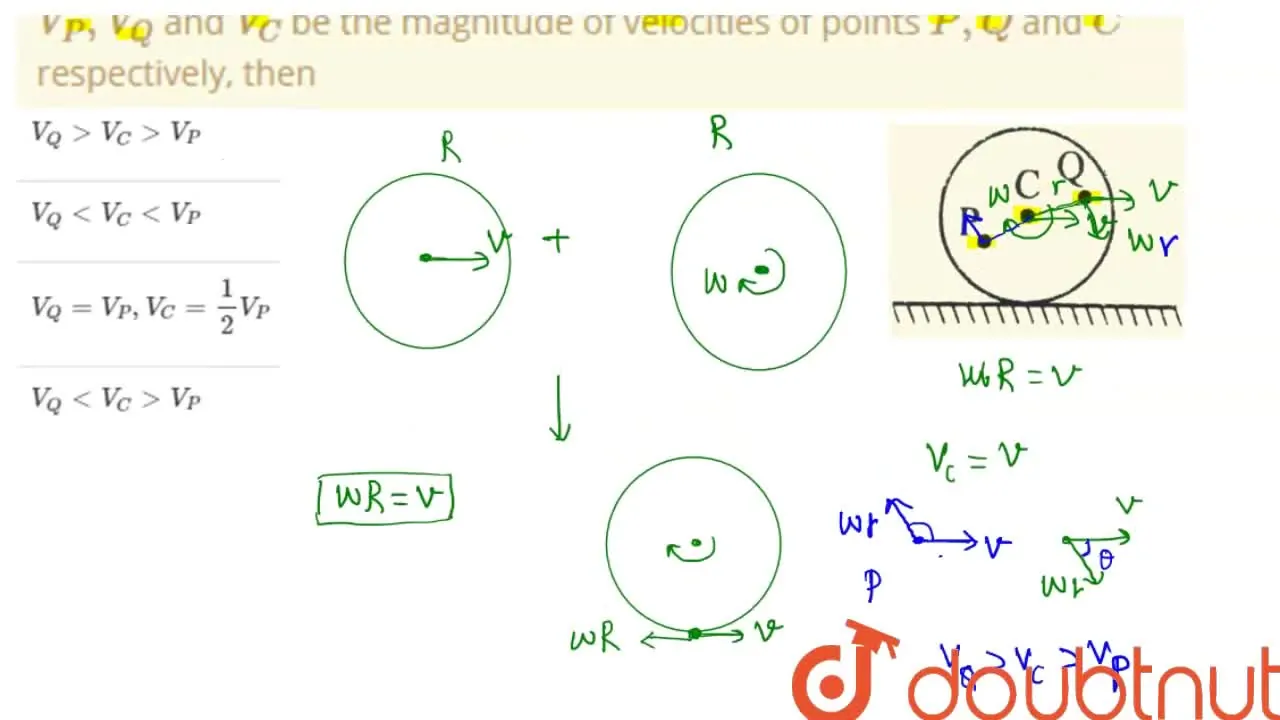
A disc is rolling (without slipping) on a horizontal surface. C is its center and Q and P are two points equidistant from C. Let VP,VQ and VC be the magnitude of velocities of points P,Q and C respectively, then
 .
.
vQ>vC>vP
vQ<vC<vP
vQ=vP, vC=vP
vQ<vC>vP
vC=v
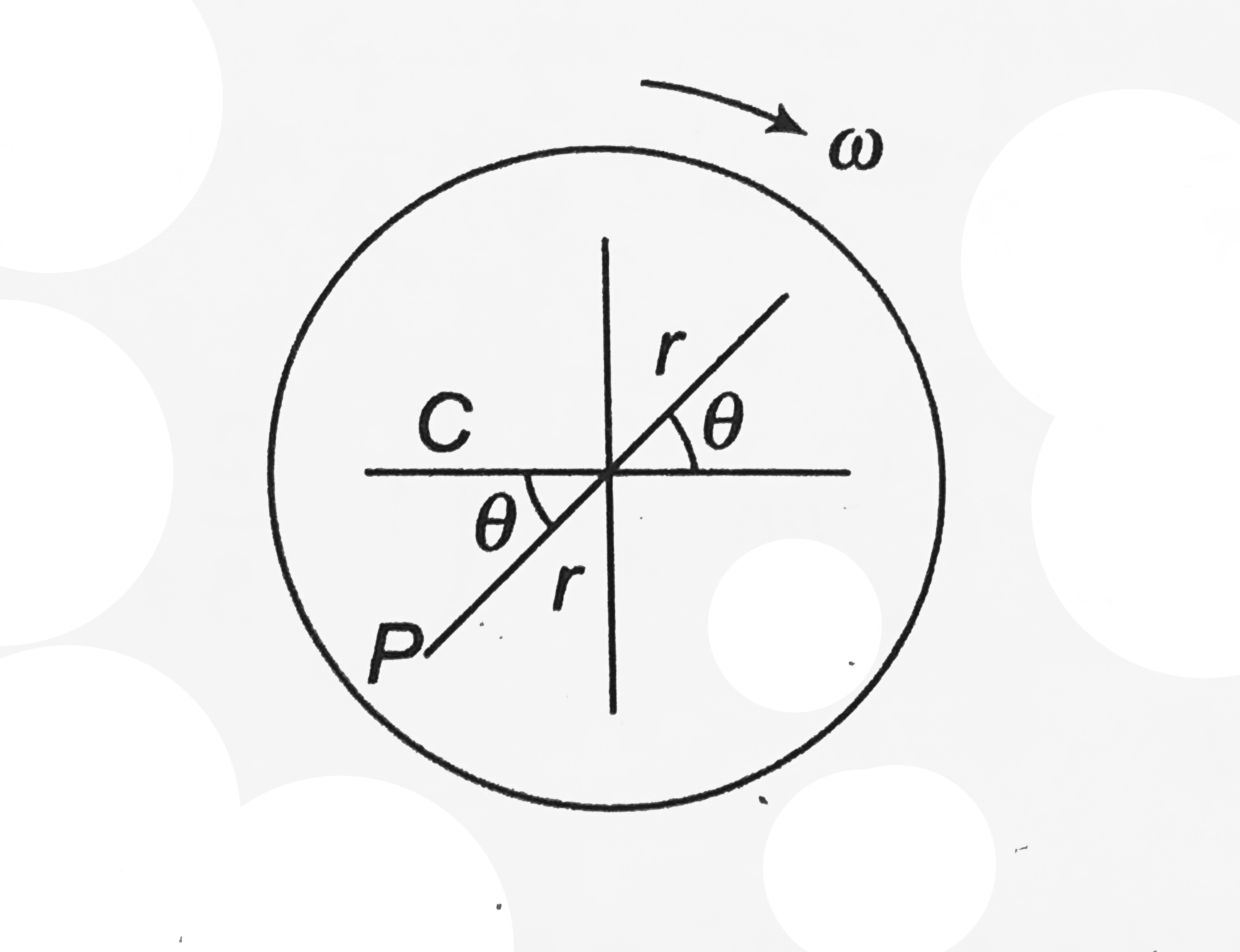
P: 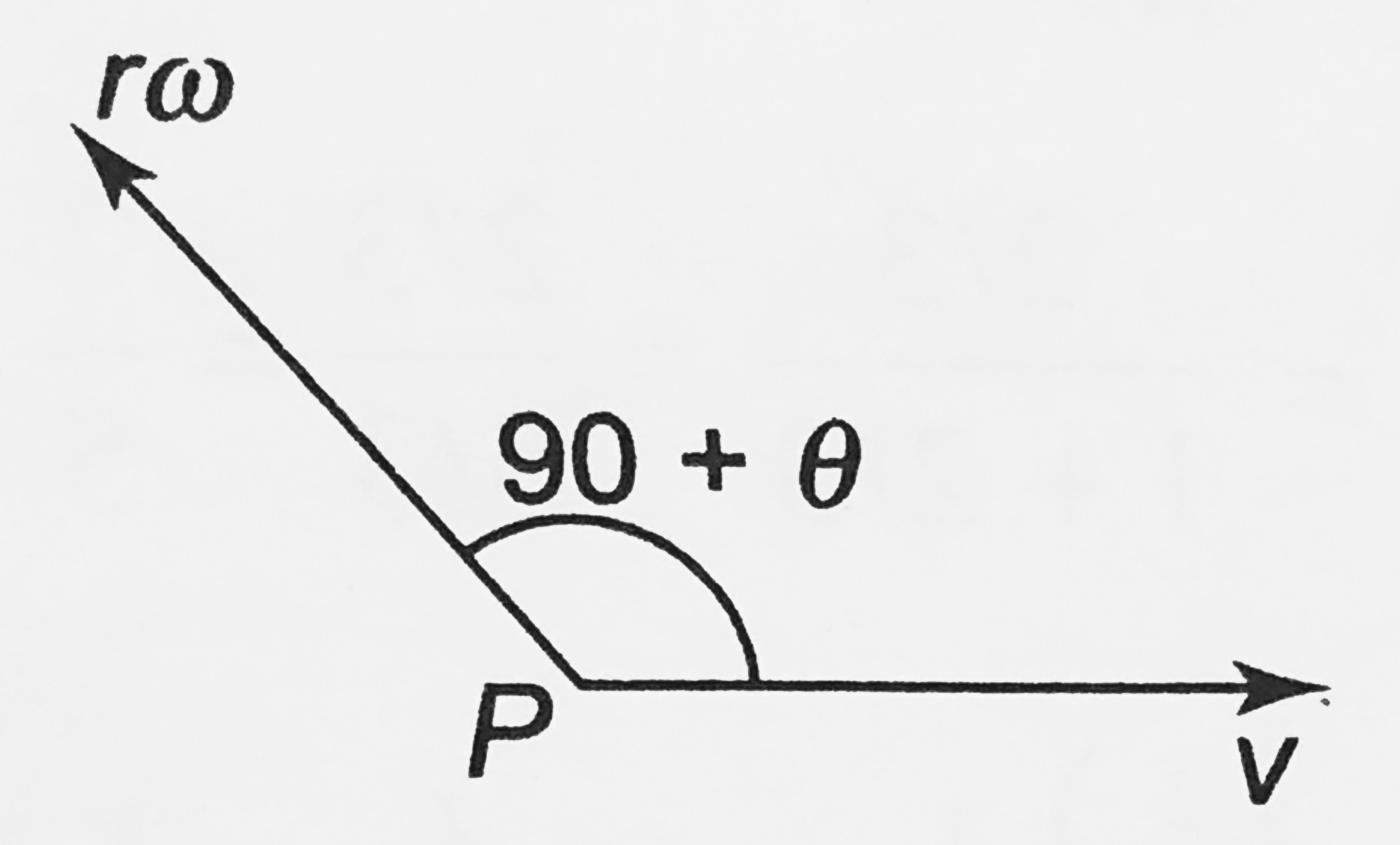
vP=√v2+(rω)2+2×v×rωcos(90+θ)
Q: 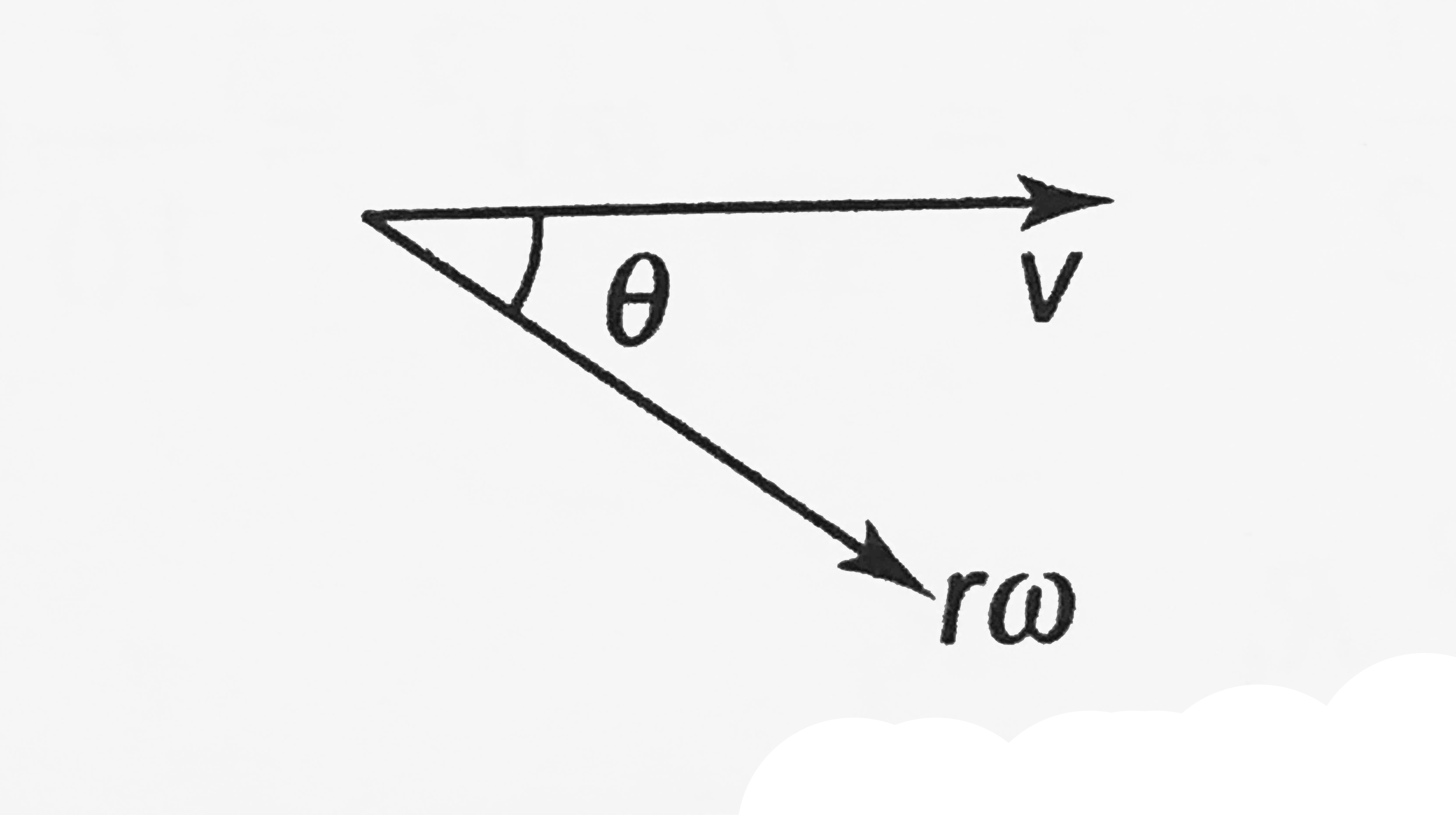
vQ=√v2+(rω)2+2×v×rωcosθ
vQ>vC>vP
OR
Assuming motion as the case of pure rotation about the point of contact 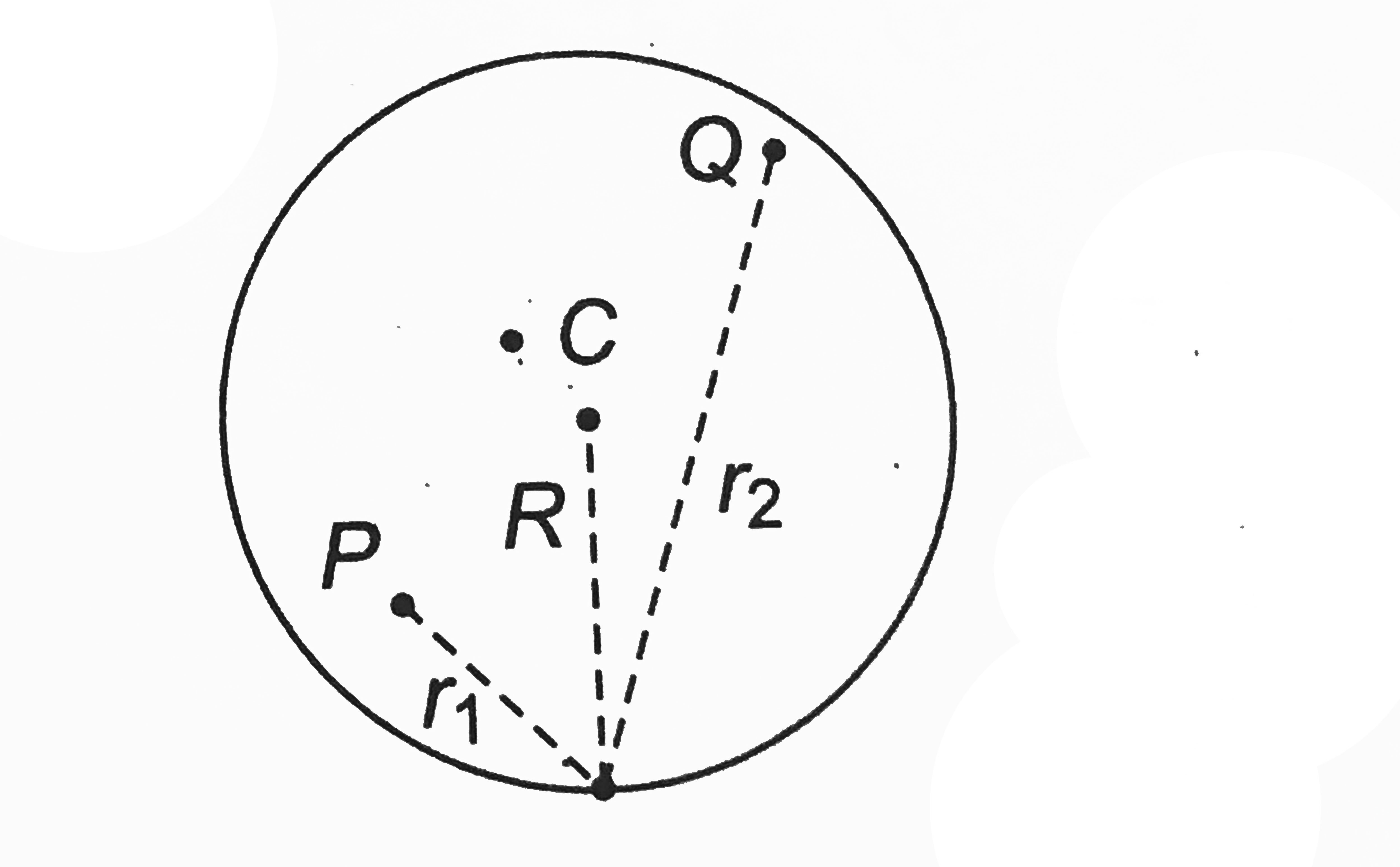
vC=Rω,vQ=r2ω,vP=r1ω
vQ>vC>vP
Related Playlists
Similar Questions
A disc is rolling without slipping on a horizontal surface with C, as its centre and Q and P the two points equidistant from C. Let vP,vQandvC be the magnitudes of velocities of point P,Q and C respectively, then
 View Solution
View Solution
A disc is rolling (without slipping) on a horizontal surface. C is its centre and Q and P are two point equidistanec from C. let υp,υQ and υC be the magnitude of velocities of points P, Q, and C repsectively,
(a). υQ>υC>υP
(b). υQ<υC<υP
(c). υQ=υP,υC=12υP
(d). υQ<υC>υPView SolutionA disc is rolling without slipping with angular velocity ω. P and Q are two points equidistant from the centre C. the order of magnitude fo velocity is
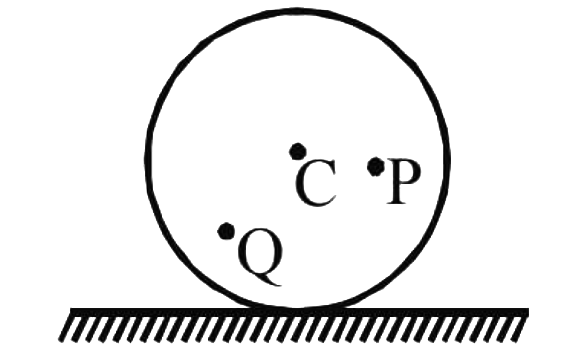 View Solution
View SolutionA disc rolls without slipping on a horizontal surface such that its velocity of center of the mass is v. Find the velocity of points A,B,C and D.
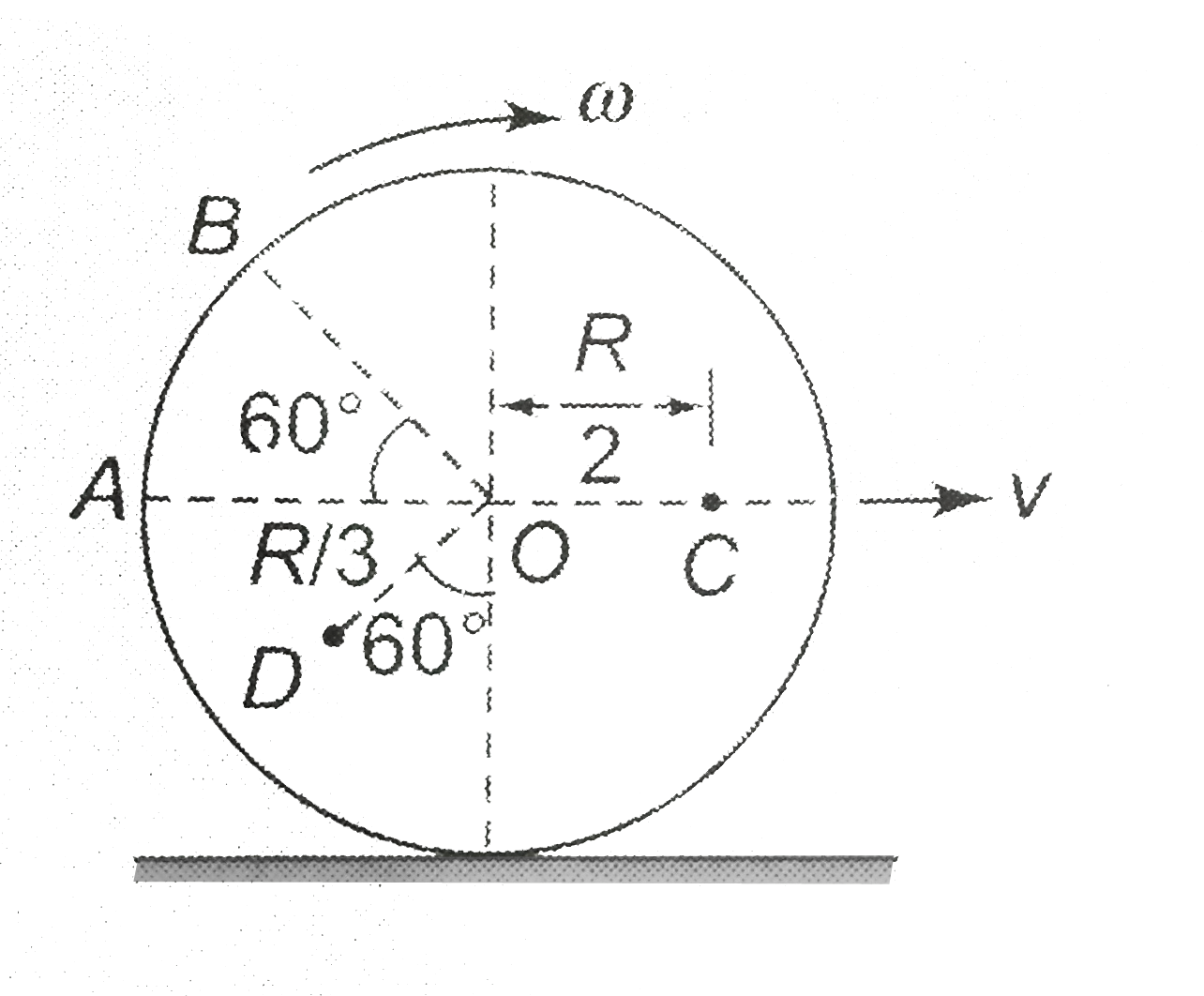 View Solution
View SolutionA disc is rolling without sliding on a horizontal surface. Velocity of the centre of the disc is V. Then the maximum relative sped of any point on the circumference of the disc with respect to point P is.
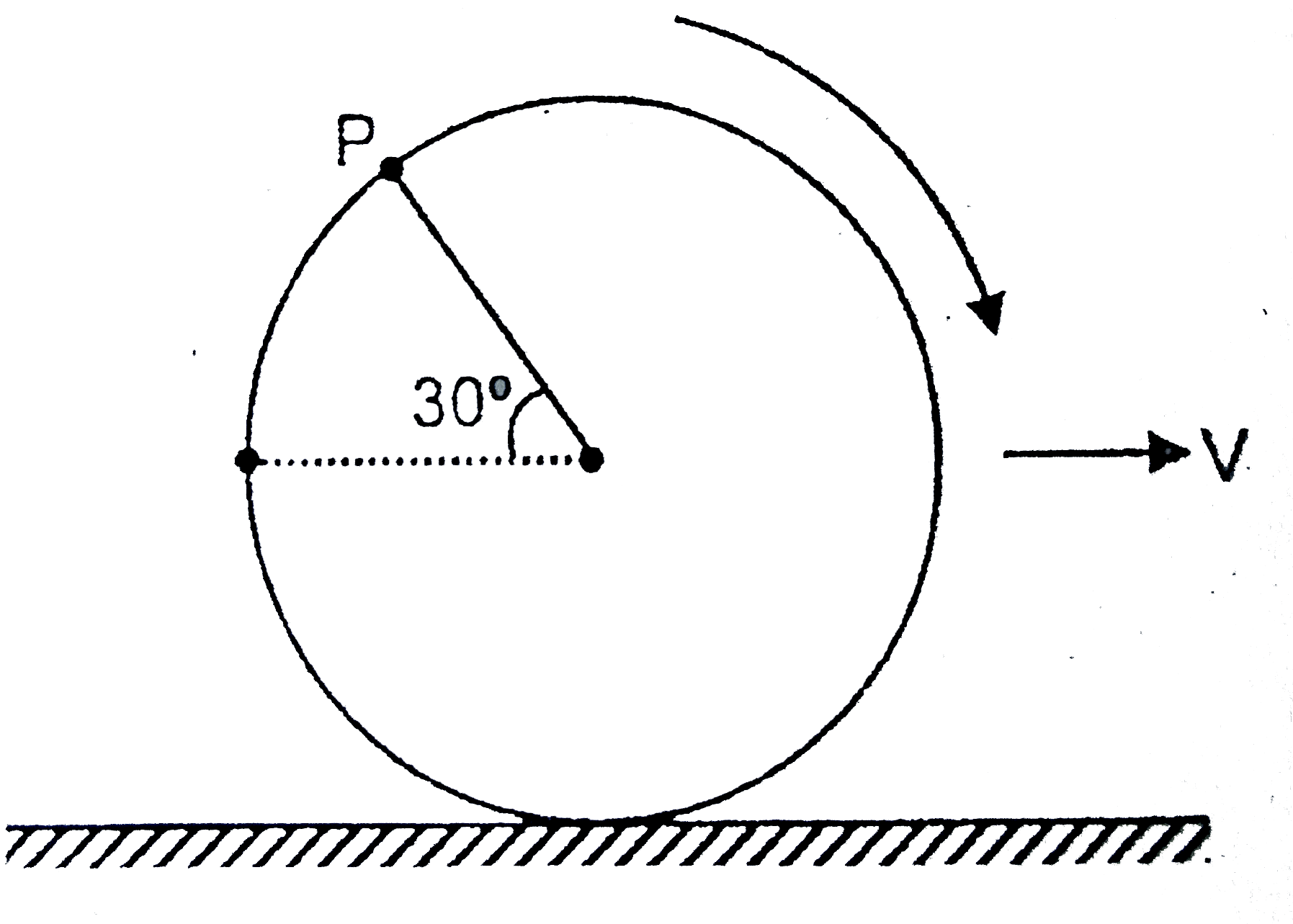 View Solution
View SolutionA hollow conducting sphere is placed in an electric field produced by a point charge placed at P as shown in figure.
Let VA,VB,VC be the potentials at points A,BandC respectively. Then
 View Solution
View SolutionA solid sphere of mass m and radius R rolls without slipping on a horizontal surface such that vc.m.=v0.
View SolutionA wheel rolls without slipping on a horizontal surface such that its velocity of center of mass is v. The velocity of a particle at the highest point of the rim is
View SolutionA solid disc rolls clockwise without slipping over a horizontal path with a constant speed v. Then the magnitude of the velocities of points A,B and C (see figure) with respect to a standing observer are, respectively,
 .View Solution
.View SolutionThe point P(x,y) is equidistant from the points Q(c+d,d−c)andR(c−d,c+d) then
View Solution