A heavy circular disc of mass M is revolving in a horizontal plane wit

A uniform disc of mass M and radius R is rotating about a horizontal axis passing through its centre with angular velocity $ω$ . A piece of mass m breaks from the disc and flies off vertically upwards. The angular speed of the disc will be
A $\frac{(M - 2 m) ω}{(M - m)}$
B $\frac{(M + 2 m) ω}{(M + m)}$
C $\frac{(M - 2 m) ω}{(M + m)}$
D $\frac{(M + 2 m) ω}{(M - m)}$
As shown in the figure, a disc of mass m is rolling without slipping with angular velocity $ω$ . When it crosses point B disc will be in

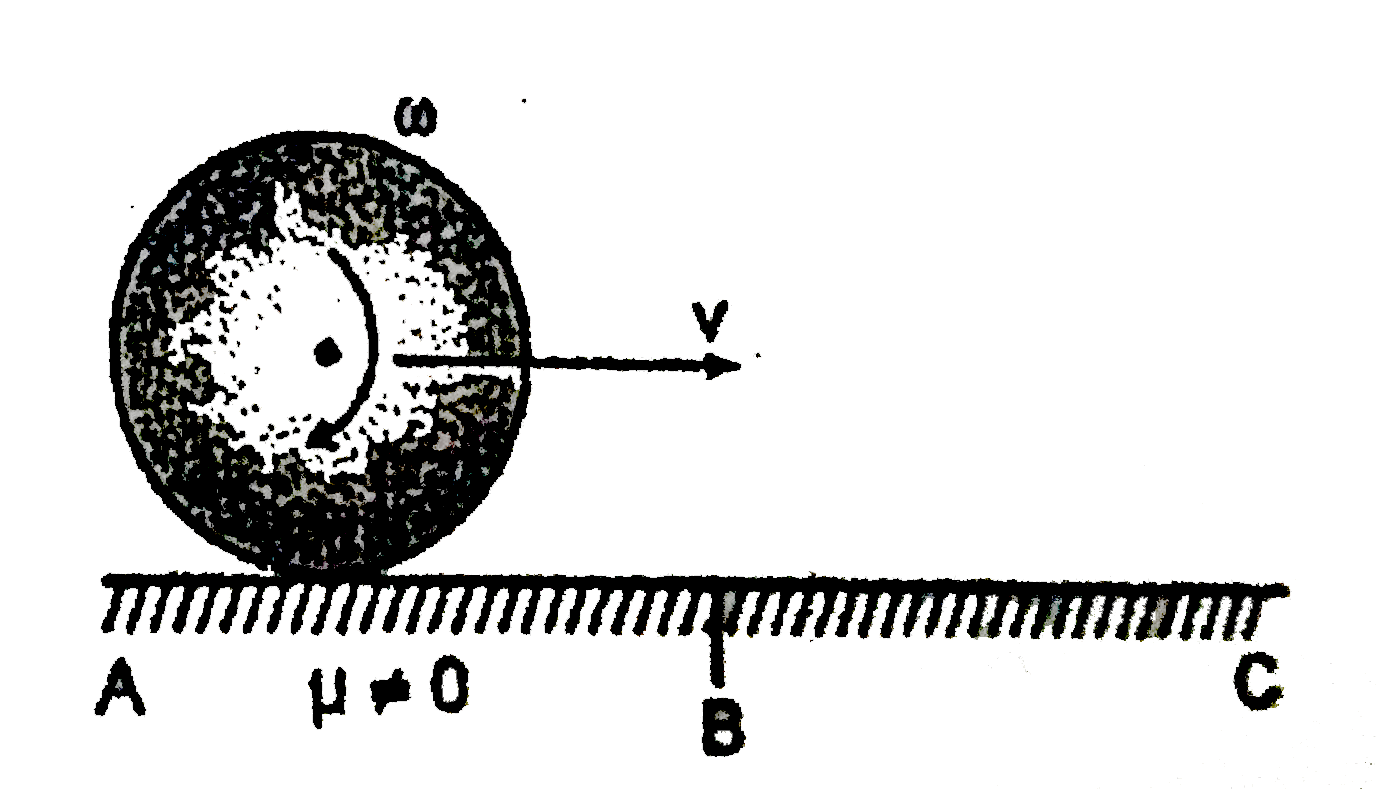Atranslational motion only
Bpure rolling motion
Crotational motion only
Dnone of these
A uniform disc of radius 'a' and mass 'm' is rotating freely with angular speed $ω$ in a horizontal plane about a smooth fixed vertical axis through its centre. A particle of mass 'm' is then suddenly attached to the rim of the disc and rotates with it. The new angular speed is
A $ω / \sqrt{3}$
B $ω / 3$
C $ω / \sqrt{4}$
D $ω / 5$

A heavy circular disc of mass $M$ is revolving in a horizontal plane with angular speed $ω_{0}$ about its center which is fixed. An insect of mass $m$ walks from the center to the edge and then files away. Find the final angular speed of disc.