From a uniform wire, two circular loops are made (i) P of radius r and (ii) Q of radius nr. If the moment of inertia of Q about an axis passing through its center and perpendicular to tis plane is 8 times that of P about a similar axis, the value of n is (diameter of the wire is very much smaller than r or nr)
8
6
4
2
To solve the problem, we need to find the value of n given that the moment of inertia of loop Q is 8 times that of loop P. Let's break this down step by step.
Step 1: Understand the Moment of Inertia Formula
The moment of inertia I of a circular loop about an axis passing through its center and perpendicular to its plane is given by the formula:
I=mr2
where m is the mass of the loop and r is its radius.
Step 2: Determine the Mass of Each Loop
Since the wire is uniform, the mass of each loop is proportional to its circumference.
- For loop P with radius r:
Circumference of P=2πr
Let the mass of loop P be mP. Thus:
m_P \propto 2\pi r \implies m_P = k \cdot 2\pi r \quad \text{(where k is a constant)}
- For loop Q with radius nr:
Circumference of Q=2π(nr)=2nπr
Let the mass of loop Q be mQ. Thus:
mQ∝2nπr⟹mQ=k⋅2nπr
Step 3: Calculate the Moments of Inertia
Now we can calculate the moments of inertia for both loops.
- For loop P:
IP=mPr2=(k⋅2πr)r2=2πkr3
- For loop Q:
IQ=mQ(nr)2=(k⋅2nπr)(nr)2=2nπkn2r3=2n3πkr3
Step 4: Set Up the Equation Based on the Given Condition
According to the problem, the moment of inertia of Q is 8 times that of P:
IQ=8IP
Substituting the expressions we derived:
2n3πkr3=8(2πkr3)
Step 5: Simplify the Equation
We can cancel out 2πkr3 from both sides (assuming k, r, and π are non-zero):
n3=8
Step 6: Solve for n
Taking the cube root of both sides:
n=√(3)8=2
Final Answer
Thus, the value of n is:
2
The moment of inertia I of a circular loop about an axis passing through its center and perpendicular to its plane is given by the formula:
I=mr2
where m is the mass of the loop and r is its radius.
Since the wire is uniform, the mass of each loop is proportional to its circumference.
Circumference of P=2πr
Let the mass of loop P be mP. Thus:
m_P \propto 2\pi r \implies m_P = k \cdot 2\pi r \quad \text{(where k is a constant)}
Circumference of Q=2π(nr)=2nπr
Let the mass of loop Q be mQ. Thus:
mQ∝2nπr⟹mQ=k⋅2nπr
Now we can calculate the moments of inertia for both loops.
IP=mPr2=(k⋅2πr)r2=2πkr3
IQ=mQ(nr)2=(k⋅2nπr)(nr)2=2nπkn2r3=2n3πkr3
According to the problem, the moment of inertia of Q is 8 times that of P:
IQ=8IP
Substituting the expressions we derived:
2n3πkr3=8(2πkr3)
We can cancel out 2πkr3 from both sides (assuming k, r, and π are non-zero):
n3=8
Taking the cube root of both sides:
n=√(3)8=2
Thus, the value of n is:
2
Topper's Solved these Questions
Similar Questions
The moment of inertia of a uniform ring about an axis passing through its centre and perpendicular to its plane is 100kgm2. What is the moment of inertia of the ring about its diameter ?
View SolutionCalculate the moment of inertia of a disc of radius R and mass M, about an axis passing through its centre and perpendicular to the plane.
View Solution
Knowledge Check
From a uniform wire, two circular loops are made (i) P of radius r and (ii) Q of radius nr. If the moment of inertia of Q about an axis passing through its center and perpendicular to tis plane is 8 times that of P about a similar axis, the value of n is (diameter of the wire is very much smaller than r or nr)
A8B6C4D2A disc of mass m and radius R has a concentric hole of radius r. Its moment of inertia about an axis through its center and perpendicular to its plane is
A12m(R−r)2B12m(R2−r2)C12m(R+r)2D12m(R2+r2)Moment of inertia of a ring of mass M and radius R about an axis passing through the centre and perpendicular to the plane is
A1/2MR2BMR2C1/4MR2D3/4MR2
The moment of inertia of a thin square plate ABCD of uniform thickness about an axis passing through its center and perpendicular to its plane will
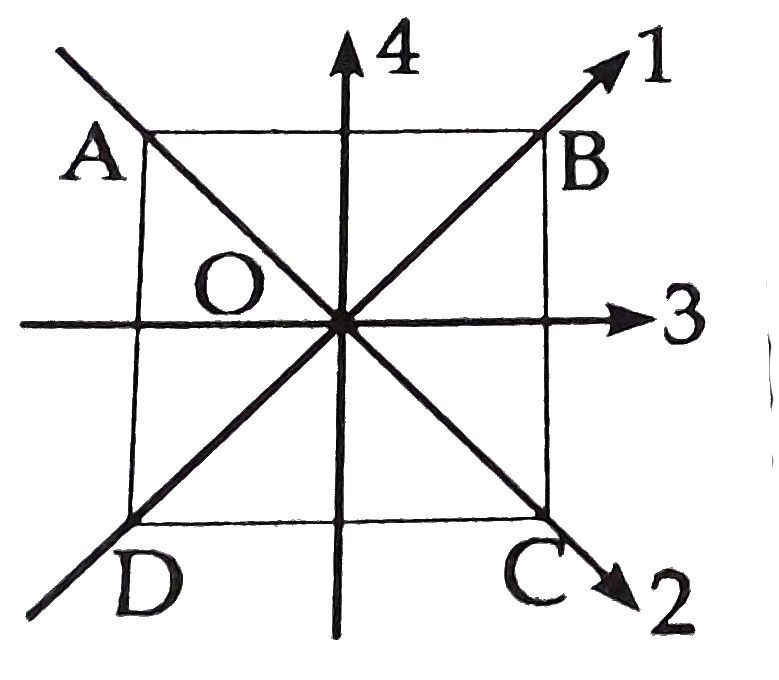
View SolutionRadius of gyration of a uniform circular disc about an axis passing through its centre of gravity and perpendicular to its plane is
View SolutionThe moment of inertia of a sphere of radius R about an axis passing through its centre is proportional to-
View SolutionMoment of inertia of a rectangular plate about an axis passing through P and perpendicular to the plate is I. Moment of PQR about an axis perpendicular to the plane of the plate :
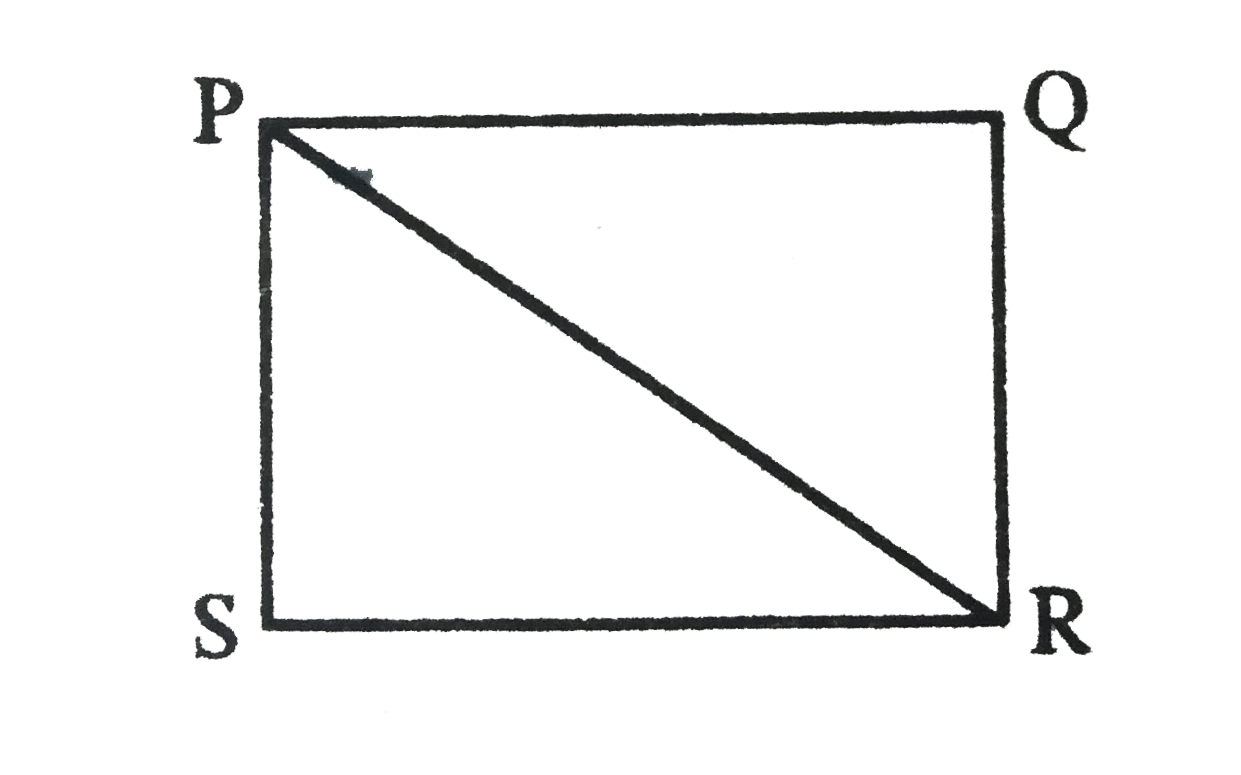 .
.
View SolutionThe moment of inertia of ring about an axis passing through its diameter is I. Then moment of inertia of that ring about an axis passing through its centre and perpendicular to its plane is
View Solution
