The work done 'w' by a body varies with displacement 'x' as w=Ax+B(C−x)2. The demensional formula for 'B' is
[ML2T−2]
[ML4T−2]
[MLT−2]
[ML2T−4]
The correct Answer is:B
w=Ax+B(C−x)2 (principle of homogenity)
Topper's Solved these Questions
- UNITS AND MEASUREMENTSBOOK - NARAYNACHAPTER - UNITS AND MEASUREMENTSEXERCISE - LEVEL-III
21 Videos
- UNITS AND MEASUREMENTSBOOK - NARAYNACHAPTER - UNITS AND MEASUREMENTSEXERCISE - Single Option Questions
12 Videos
- UNITS AND MEASUREMENTSBOOK - NARAYNACHAPTER - UNITS AND MEASUREMENTSEXERCISE - LEVEL-I (C.W)
42 Videos
- TRANSMISSION OF HEATBOOK - NARAYNACHAPTER - TRANSMISSION OF HEATEXERCISE - LEVEL-II(C.W)
27 Videos
- VECTORSBOOK - NARAYNACHAPTER - VECTORSEXERCISE - LEVEL-II (H.W)
14 Videos
Similar Questions
The work done 'w' by a body varies with displacement 'x' as w=Ax+(B)/(c-x)^(2) .The dimensional formula for B is
View SolutionThe potential energy of a particle varies with distance 'x' as U=(Ax^(1/2))/(x^(2)+B),where A and B are constants.The dimensional formula for A times B is [M^(a)L^(b)T^(c)] then a=
View Solution
Knowledge Check
The work done 'w' by a body varies with displacement 'x' as w=Ax+B(C−x)2. The demensional formula for 'B' is
A[ML2T−2]B[ML4T−2]C[MLT−2]4D[ML2T−4]Hydrostatic pressure 'P' varies with displacement 'x' as P=ABlog(Bx2+C) where A,B, and C are constants. The dimensional formula for 'A' is
A[M1L−1T−2]BMLT−2]C[ML−2T−2]DML−3T−2]if work is done by the system then W is
A(+)veB(-)veCZeroDDepends upon the thermodynamic process.
A force (F) acting on a particle varoes work done by a particle varies with the with the position x as shown in figure
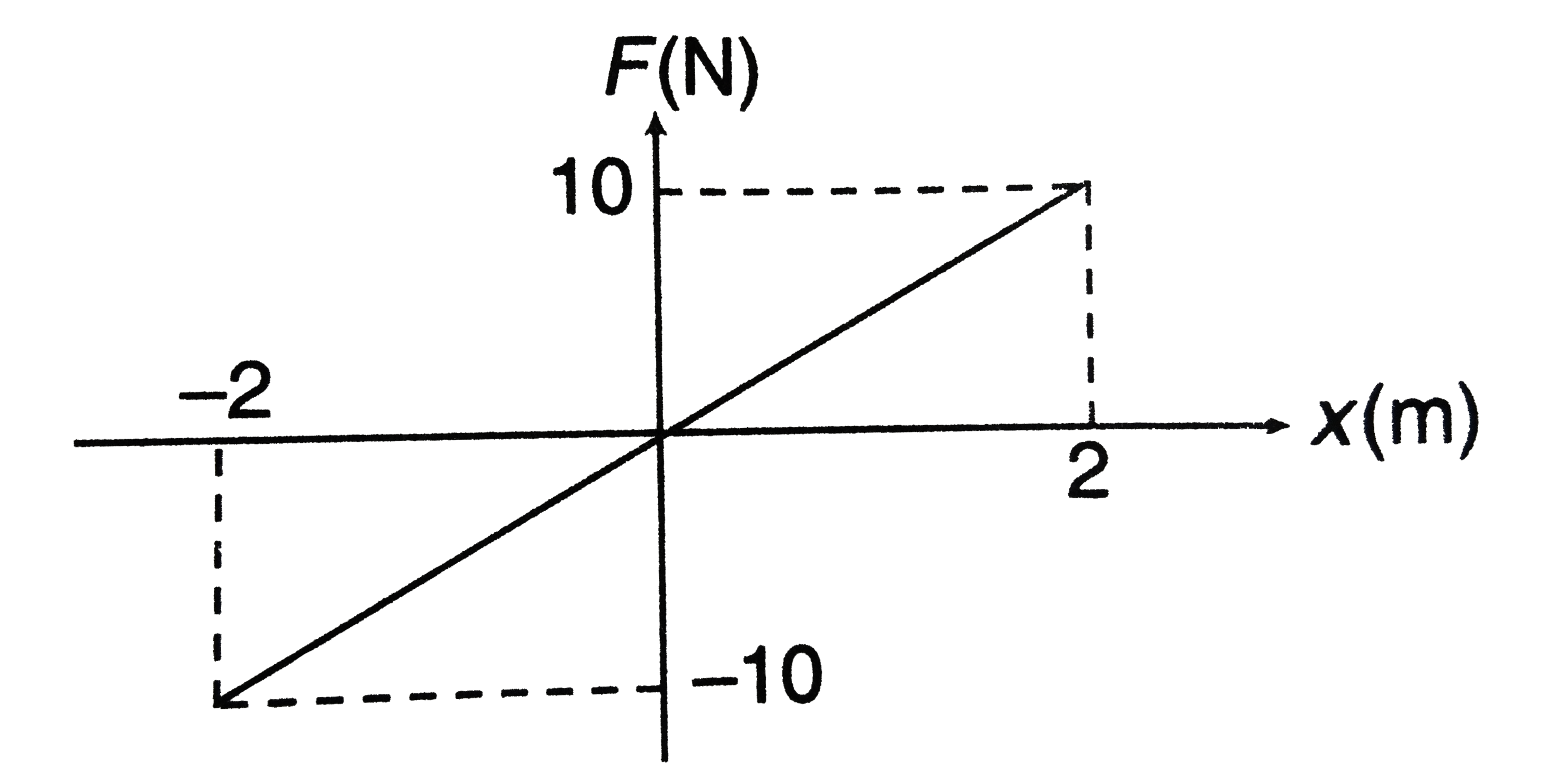 .
.
Find the work done by by force in displacing the particle from .
(a) x=−2m to x=0
(b) x=0 to x=2m..
View SolutionIf W=work done, T= Temperature, kB= boltzmann constant X = displacement &W=α(β2)e−x2αTkB then find the dimension of β
View SolutionIf W=work done, T= Temperature, kB= boltzmann constant X = discplacement & W=(α2)βe−x2βTkB then find dimension of β
View SolutionThe work done is blowing a soap bubble of volume V is W. The work done in blowing a soap bubble of volume 2V is
View SolutionIn a typical combustion engine the work done by a gas molecule is given W=α2βe−βx2kT where x is the displacement, k is the Boltzmann constant and T is the temperature. If αandβ are constants, dimensions of α will be:
View Solution
