A body is projected vertically upwards from the surface of the earth with a velocity equal to half of escape velocity of the earth. If R is radius of the earth, maximum height attained by the body from the surface of the earth is
To solve the problem of finding the maximum height attained by a body projected vertically upwards with a velocity equal to half of the escape velocity of the Earth, we can follow these steps:
Step 1: Understand the Given Data
- The escape velocity Ve from the surface of the Earth is given by the formula:
Ve=√2GMR
where G is the gravitational constant, M is the mass of the Earth, and R is the radius of the Earth.
- The initial velocity V of the body is half of the escape velocity:
V=12Ve=12√2GMR=√GM2R
Step 2: Apply Conservation of Energy
According to the law of conservation of energy:
Initial Total Energy=Final Total Energy
At the surface of the Earth (initial position):
- Kinetic Energy (KE) = 12mV2
- Potential Energy (PE) = −GMmR
At the maximum height h:
- Kinetic Energy (KE) = 0 (since the velocity is zero at maximum height)
- Potential Energy (PE) = −GMmR+h
Step 3: Set Up the Energy Equation
Setting the initial total energy equal to the final total energy:
12mV2−GMmR=−GMmR+h
Step 4: Substitute for Initial Velocity
Substituting V2:
12m(GM2R)−GMmR=−GMmR+h
This simplifies to:
GMm4R−GMmR=−GMmR+h
Step 5: Simplify the Equation
Combining terms:
GMm4R−4GMm4R=−GMmR+h
−3GMm4R=−GMmR+h
Dividing through by −GMm (assuming m≠0):
34R=1R+h
Step 6: Cross Multiply and Solve for h
Cross-multiplying gives:
3(R+h)=4R
Expanding and rearranging:
3h=4R−3R
3h=R
h=R3
Conclusion
The maximum height attained by the body from the surface of the Earth is:
R3
- The escape velocity Ve from the surface of the Earth is given by the formula:
Ve=√2GMR
where G is the gravitational constant, M is the mass of the Earth, and R is the radius of the Earth.
V=12Ve=12√2GMR=√GM2R
According to the law of conservation of energy:
Initial Total Energy=Final Total Energy
At the surface of the Earth (initial position):
- Kinetic Energy (KE) = 12mV2
- Potential Energy (PE) = −GMmR
- Kinetic Energy (KE) = 0 (since the velocity is zero at maximum height)
- Potential Energy (PE) = −GMmR+h
Setting the initial total energy equal to the final total energy:
12mV2−GMmR=−GMmR+h
Substituting V2:
12m(GM2R)−GMmR=−GMmR+h
This simplifies to:
GMm4R−GMmR=−GMmR+h
Combining terms:
GMm4R−4GMm4R=−GMmR+h
−3GMm4R=−GMmR+h
Dividing through by −GMm (assuming m≠0):
34R=1R+h
Cross-multiplying gives:
3(R+h)=4R
Expanding and rearranging:
3h=4R−3R
3h=R
h=R3
The maximum height attained by the body from the surface of the Earth is:
R3
Topper's Solved these Questions
- GRAVITATIONBOOK - NARAYNACHAPTER - GRAVITATIONEXERCISE - C.U.Q
220 Videos
- GRAVITATIONBOOK - NARAYNACHAPTER - GRAVITATIONEXERCISE - LEVEL I(C.W.)
51 Videos
- FRICTIONBOOK - NARAYNACHAPTER - FRICTIONEXERCISE - Passage type of questions I
6 Videos
- KINETIC THEORY OF GASESBOOK - NARAYNACHAPTER - KINETIC THEORY OF GASESEXERCISE - LEVEL-III(C.W)
52 Videos
Similar Questions
A body is projected vertically upwards from the surface of earth with a velocity equal to half the escape velocity. If R be the radius of earth, maximum height attained by the body from the surface of earth is Rn. Find the value of n.
View SolutionA body is projected vertically upward from. the surface of the earth.with a velocity equal to half its escape velocity. If R is radius of the earth, then the maximum height attained by the,body is (Rn). Find n.
View Solution
Knowledge Check
A body is projected vertically upward from the surface of the earth, then the velocity-time graph is:-
A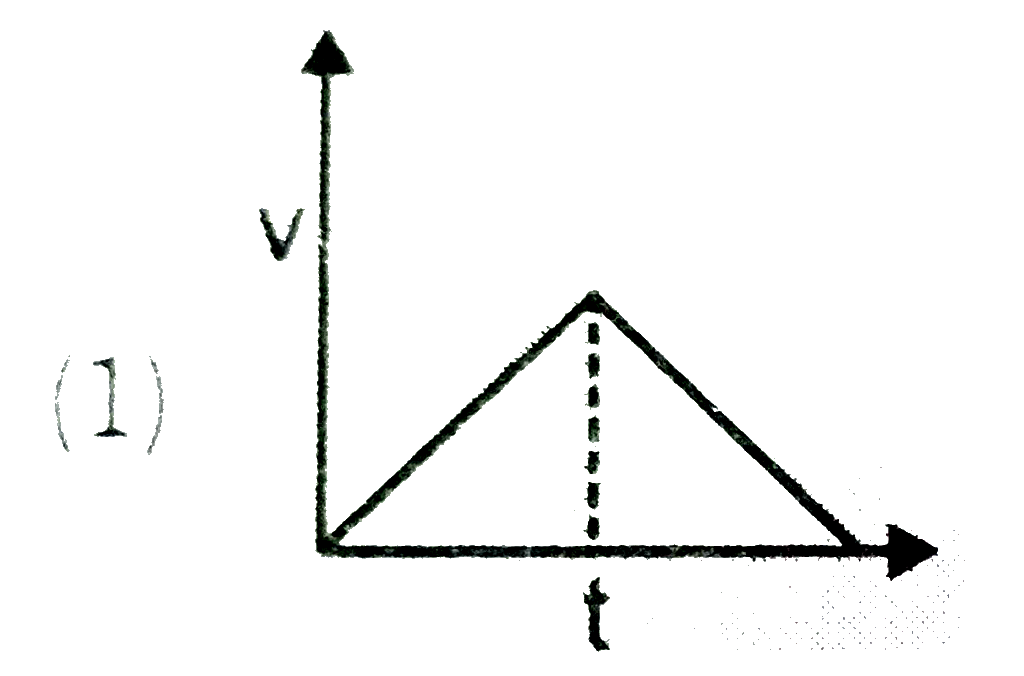 B
B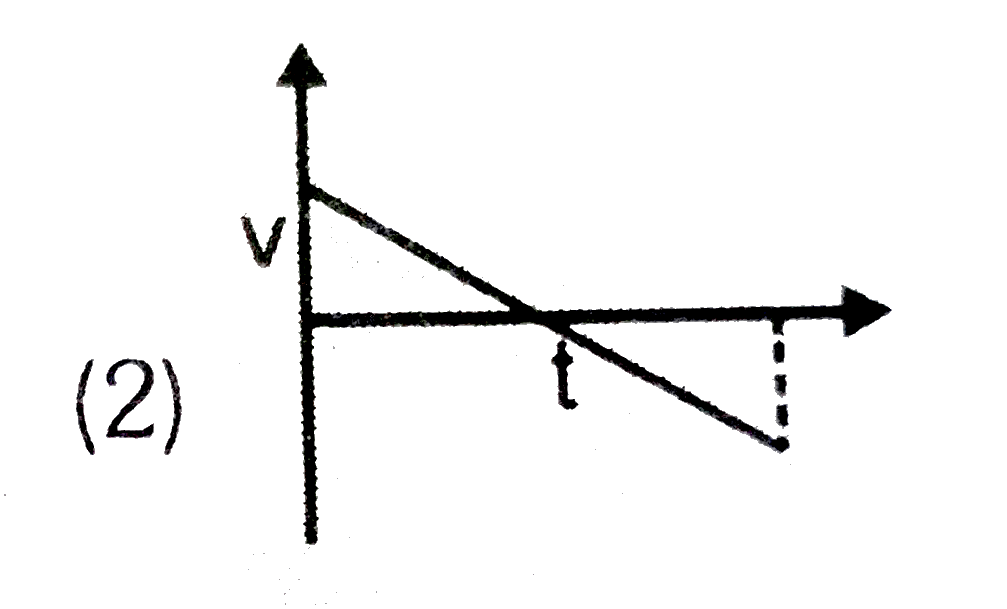 C
C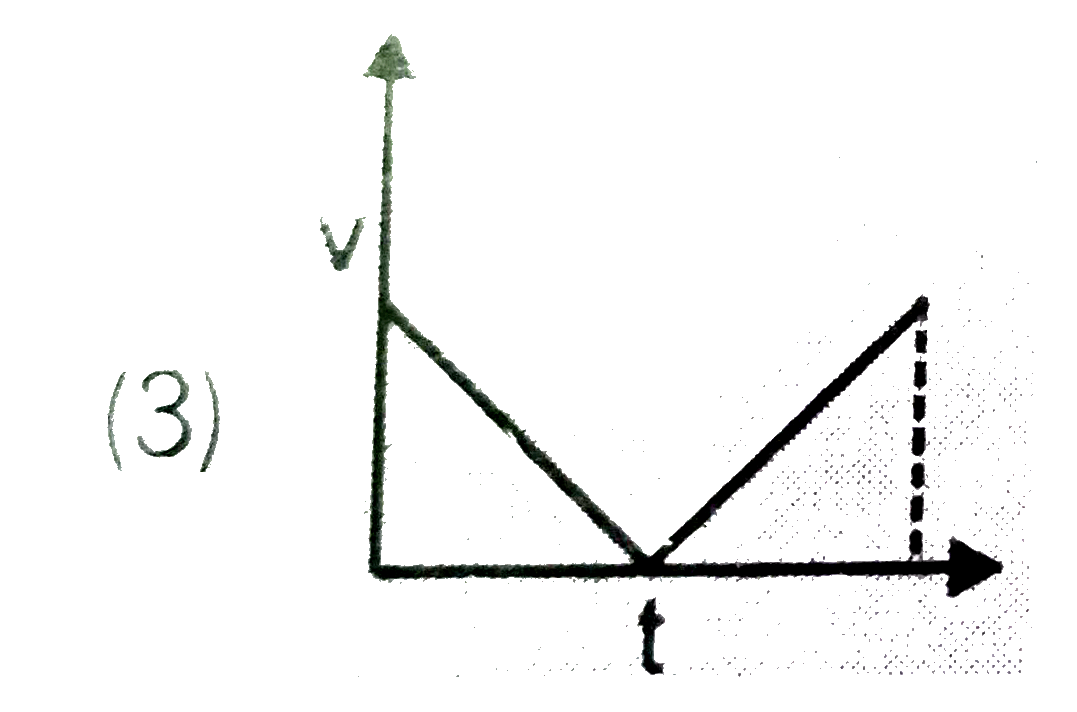 D
D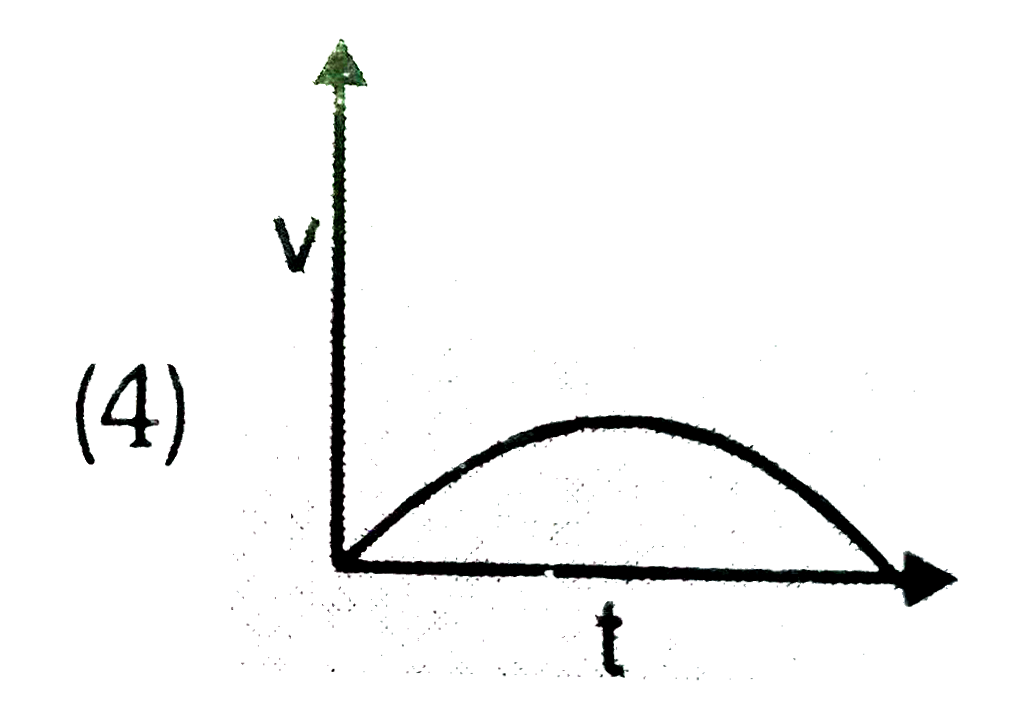
A body is projected vertically up from surface of the earth with a velocity half of escape velocity. The ratio of its maximum height of ascent and radius of earth is
A1:1B1:2C1:3D1:4A body is projected vertically upwards from the surface of a planet of radius R with a velocity equal to hall the escape velocity for that planet. The maximum height attained by the body is
AR/2BR/3CR/5DR/4
A particle is projected vertically upwards the surface of the earth (radius Re) with a speed equal to one fourth of escape velocity what is the maximum height attained by it from the surface of the earth?
View SolutionA body is projected vertically upwards from the surface of a planet of radius R with a velocity equal to 1/3rd the escape velocity for the planet. The maximum height attained by the body is
View SolutionA body is projected horizontally from the surface of the earth (radius =R) with a velocity equal to n times the escape velocity. Neglect rotational effect of the earth. The maximum height attained by the body from the earth s surface is R/2. Then, n must be
View SolutionA particle is prjected vertically upwards the surface of the earth (radius Re) with a speed equal to one fourth of escape velocity what is the maximum height attained by it from the surface of the earth?
View SolutionThe escape velocity from the surface of the Earth is
View Solution