A simple pendulam of length L carriers a bob of mass m. When the bob is at its lowest position, it is given the minimum horizontal speed necessary for it to move in a vertical circle about the point of suspension. When the string is horizontal the net force on the bob is
√10mg
√5mg
4 mg
1 mg
The correct Answer is:A
Step by step video, text & image solution for A simple pendulam of length L carriers a bob of mass m. When the bob is at its lowest position, it is given the minimum horizontal speed necessary for it to move in a vertical circle about the point of suspension. When the string is horizontal the net force on the bob is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CIRCULAR MOTIONBook:TARGET PUBLICATIONChapter:CIRCULAR MOTIONExercise:EVALUATION TEST
Explore 11 Videos
- CIRCULAR MOTIONBook:TARGET PUBLICATIONChapter:CIRCULAR MOTIONExercise:CRITICAL THINKING
Explore 93 Videos
- ATOMS, MOLECULES AND NUCLEI Book:TARGET PUBLICATIONChapter:ATOMS, MOLECULES AND NUCLEI Exercise:Evaluation test
Explore 19 Videos
- CURRENT ELECTRICITY Book:TARGET PUBLICATIONChapter:CURRENT ELECTRICITY Exercise:Evaluation Test
Explore 6 Videos
Similar Questions
A simple pendulm has a length L and a bob of mass M. The bob is vibrating with amplitude a .What is the maximum tension in the string?
View SolutionA simple pendulum of length L having a bob of mass m is deflected from its rest position by an angle θ and released figure. The string hits a peg which is fixed at distance x below the point of suspension and the bob starts going in a circle centred at the peg. a. Assuming that initially the bob has a height less thasn the peg, show that the maximum height reached by the bob equals its initialheight. b. If the pendulum is released with θ=900 and m=L2 find the maximum height reached by the bob above its lowest positon before the string becomes slack. c. Find the minimum value of x/L for which the bob goes in a complete circle about the pet when the pendulum is released from θ=900.
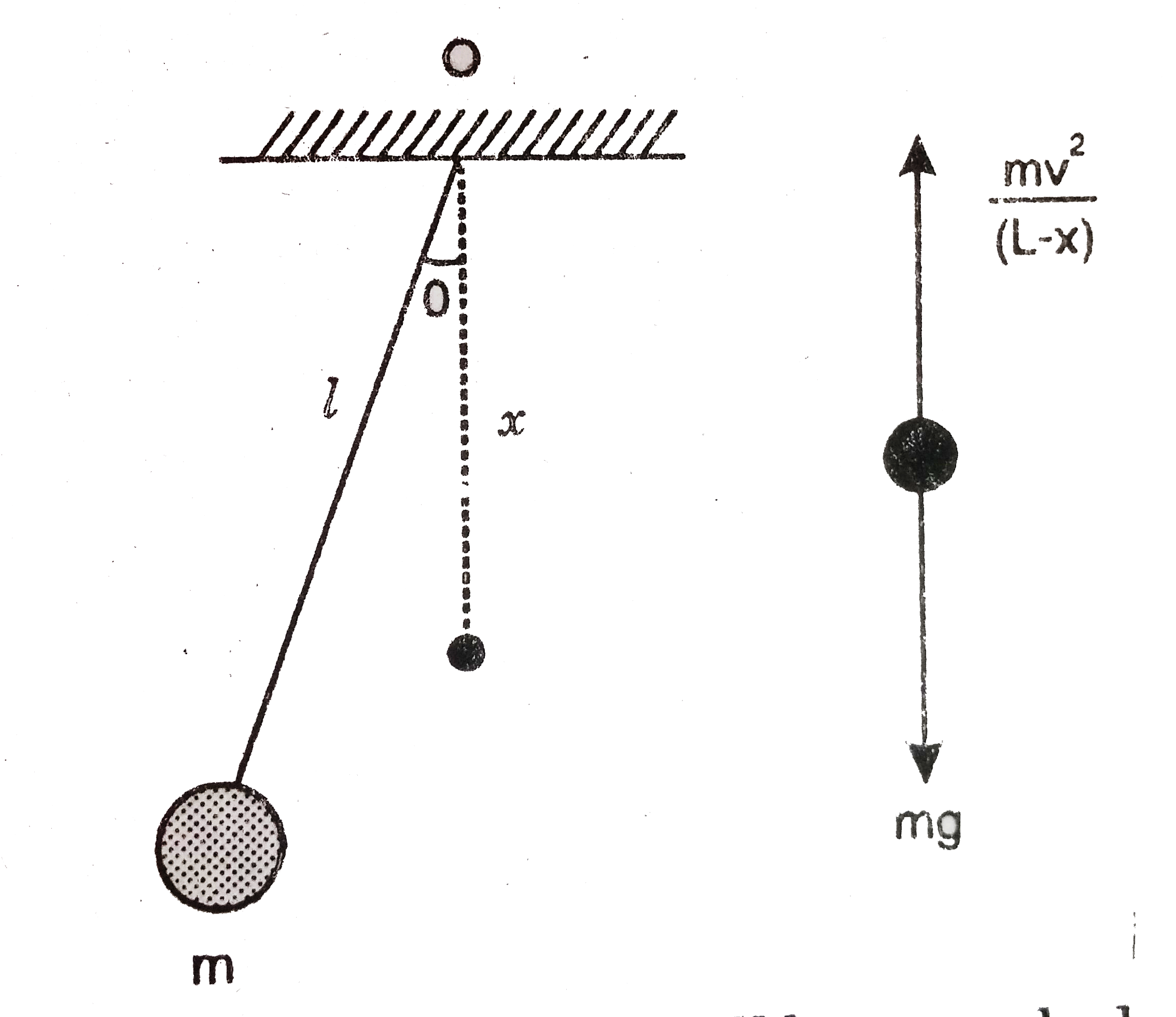
View Solution
Knowledge Check
In the previous question, when the string is horizontal, the net force on the bob is
AmgB3mgC√10mgD4mgA simple pendulum has a string of length l and bob of mass m. When the bob is at its lowest position , it is given the minimum horizontal speed necessary for it to move in a circular path about the point point of suspension. The tension in the string at the lowest position if the bob is
A3mgB4mgC5mgD6mgA simple pendulum of length l and mass m is initially at its lowest position. It is given the minimum horizontal speed necessary after to move in a circular path about the point of suspension. The tension in the string at the lowest positive of the bob is
A3mgB4mgC5mgD6mg
A simple pendulum has a bob of mass m and string of length R. The bob is projected from lowest position giving it a horizontal velocity just enough for it to complete the vertical circle. Let the angular displacement of the pendulum from its initial vertical position be represented by θ. Plot the variation of kinetic energy (kE) of the bob and the tension (T) in the string with θ. Plot the graph for one complete rotation of the pendulum.
View SolutionA simple pendulum is constructed by attaching a bob of mas m to a string of length L fixed at its upper end. The bob oscillates in a vertical circle. It is found that the speed of the bob is v when the string makes an angle θ with the vertical. Find the tension in the string at this instant.
View SolutionA simple pendilum with bob of mass m and length l is held in position at an angle θ with t he verticle. Find its speed when it passes the lowest position.
View SolutionThe string of a pendulum is horizontal. The mass of the bob is m. Now the string is released. The tension in the string in the lowest position is -
View SolutionA simple pendulum of mass m and length L is held in horizontal position. If it is released from this position, then find the angular momentum of the bob about the point of suspension when it is vertically below the point of suspension.
View Solution
