A simple pendulum is constructed by attaching a bob of mas m to a string of length L fixed at its upper end. The bob oscillates in a vertical circle. It is found that the speed of the bob is v when the string makes an angle θ with the vertical. Find the tension in the string at this instant.
T=m(v2L+gcosα),
F=m√g2sin2α+v4L2
T=m(v2L+gcosα),
F=m√g2cos2α+v4L2
T=m(v2L+gcosα),
F=m√g2sin2α−v4L2
T=m(v2L+gcosα),
F=m√g2cos2α−v4L2
The correct Answer is:A
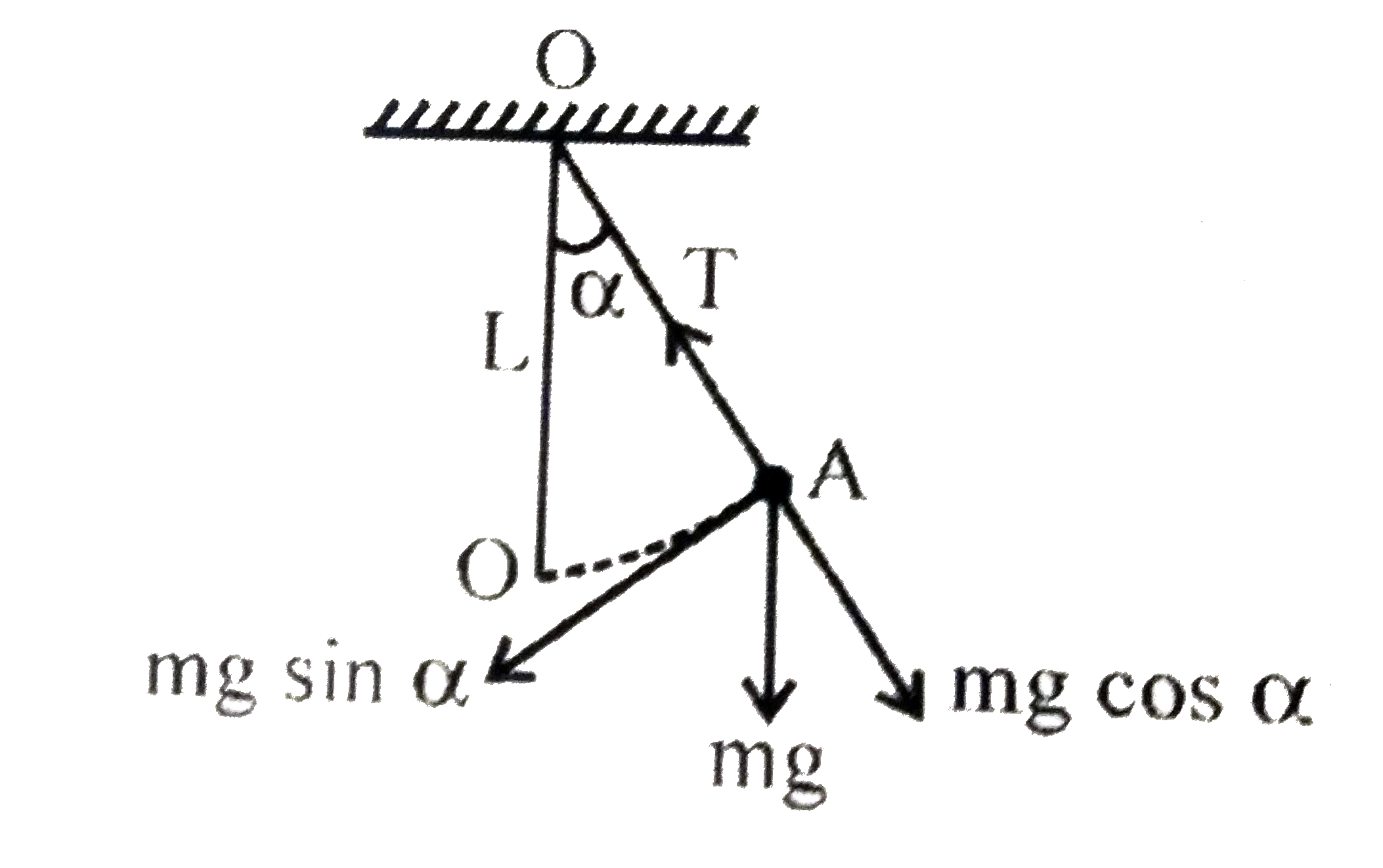
The bob moves in a circle of radius L. When it is at A, the centripetal force of magnitude mv2L is provided by T−mgcosα
∴T−mgcosα=mv2L
∴T=mv2L+mgcosα=m(v2L+gcosα)
and the net force,
F=√(mgsinα)2+(mv2L)2
=m√g2sin2α+v4L2
Related Playlists
Knowledge Check
- Question 1 - Select One
A pendulum bob of mass m charge q is at rest with its string making an angle θ with the vertical in a uniform horizontal electric field E. The tension in the string in
AmgsinθBmgCqEsinθDqEcosθ - Question 1 - Select One
A simple pendulum of length l and mass (bob) m is suspended vertically. The string makes an angle θ with the vertical. The restoring force acting on the pendulum is
AmgtanθB−mgsinθCmgsinθD−mgcosθ - Question 1 - Select One
A simple pendulum of length I and mass (bob) m is suspended vertically. The string makes an angle θ with the vertical. The restoring force acting on the pendulum is
AmgtanθB−mgsinθCmgsinθD−mgcosθ - Question 1 - Select One
A simple pendulum is released from rest with the string in horizontal position. The vertical component of the velocity of the bob becomes maximum, when the string makes an angle θ with the vertical. The angle θ is equal to
Aπ4Bcos−1(1√3)Csin−1(1√3)Dπ3 - Question 1 - Select One
A pendulum bob of mass m hangs from one end of a horizontal rod which is rotating about vertical axis with an unknown angular speed ω such that the light inextensible string of length l of the pedulum makes a constant angle θ with vertical. Find the
Q. tension in the string
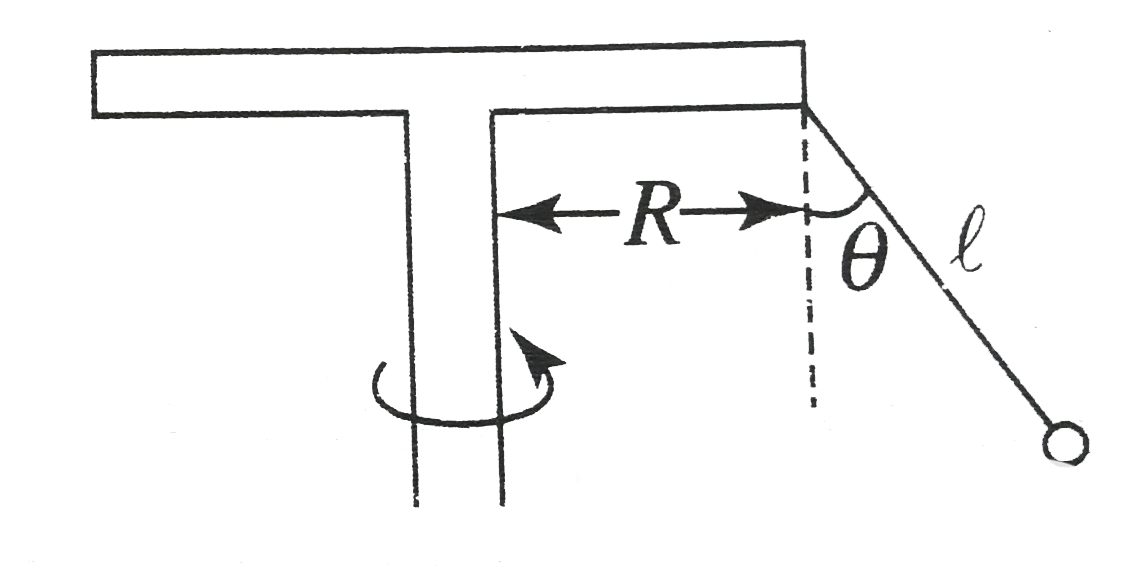 AT=m>anθBT=mgsecθCT=mgsinθcosθDT=mgcotθ
AT=m>anθBT=mgsecθCT=mgsinθcosθDT=mgcotθ - Question 1 - Select One
In a conical pendulam, when the bob moves in a horizontal circle of radius r with uniform speed v, the string of length L describes a cone of semivertical angle θ. The tension in the string is given by
AT=mgL√L2−r2BT=(L2−r2)1/2mgLCT=mgL(L2−r2)DT=mgL(L2−r2)2 - Question 1 - Select One
In a constant , when the bob moves in a horizontal circle of radius r, with uniform speed v, the string of length L describes a cone of semivertical angle θ . The tension in the string is given by
AT=mgL(L2−r2)B(L2−r2)1/2mgLCT=mgL√L2−r2DT=mgL(L2−r2)2
Similar Questions
A bob of mass m attached to an inextensible string of length l, is suspended from a vertical support. Bob rotates in a horizontal circle with angular speed ω and string makes an angle of θ with the vertical. Find the angular momentum of bob about axis of rotation
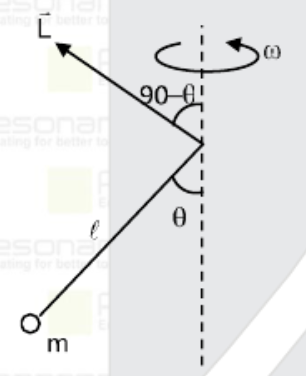 View Solution
View SolutionA small ball of mass m is attached to a string of lengh R and moves in a vertical circle with a speed v m/s about a fixed point O as illustrated in fig. Find the tension in the string, when it makes an angle θ with the vertical, in terms of v,θ and g.
View SolutionA coical pendulum consists of a string of length L whose upper end is fixed and another end is tied to a bob. The bob is moving in horizontal circle with constant angular speed ω such that the string makes a constant angle θ with the verticle. calculate time period T0 of revolution of bob in terms of L,g and θ.
View Solution

