∫px+q√x2+r2dxSolution in Bengali
The correct Answer is:p√x2+r2+qlog∣∣x+√x2+r2∣∣+c
Step by step video, text & image solution for int (px+q)/(sqrt(x^(2)+r^(2)))dx by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- METHOD OF SUBSTITUTIONBOOK - CHHAYA PUBLICATIONCHAPTER - METHOD OF SUBSTITUTIONEXERCISE - Sample Questions for Competitive Examination (Multiple Correct Answers Type)
5 Videos
- METHOD OF SUBSTITUTIONBOOK - CHHAYA PUBLICATIONCHAPTER - METHOD OF SUBSTITUTIONEXERCISE - Sample Questions for Competitive Examination (Integer Answer Type)
5 Videos
- METHOD OF SUBSTITUTIONBOOK - CHHAYA PUBLICATIONCHAPTER - METHOD OF SUBSTITUTIONEXERCISE - EXERCISE 6B (Short Answer Type Questions)
28 Videos
- MEASURMENT OF TRIGONOMETRICAL ANGLESBOOK - CHHAYA PUBLICATIONCHAPTER - MEASURMENT OF TRIGONOMETRICAL ANGLESEXERCISE - Sample Questions for Competitive Exams (Assertion -Reason Type)
2 Videos
- MISCELLANEOUS EXAMPLESBOOK - CHHAYA PUBLICATIONCHAPTER - MISCELLANEOUS EXAMPLESEXERCISE - WBJEE 2026
23 Videos
Similar Questions
∫√1+cosecxdx equals-
View SolutionIf ∫dxx12+x13=k3√x−k2x13+kx16−klog∣∣∣x16+1∣∣∣+c, then the value of k is-
View Solution∫dx√x2−ax
View SolutionStatement -I : The radian measurement of the angle of a regular octagon in radian is (3π4)c
Statement -II: Angle of an n sided regular polygon=(2n−4)n×90∘ .
View Solution72n+16n−1(n∈N) is divisble by
View SolutionIntegral of the form ∫(px+q)√ax2+bx+cdx
View Solution∫x2 √x+2 dx (ii) ∫x2√x−1 dx
View SolutionConsider
∫x3+3x2+2x+1√x2+x+1dx
=(ax2+bx+c)√x2+x+1+λ∫dx√x2+x+1
Now, match the following lists and then choose the correct code.
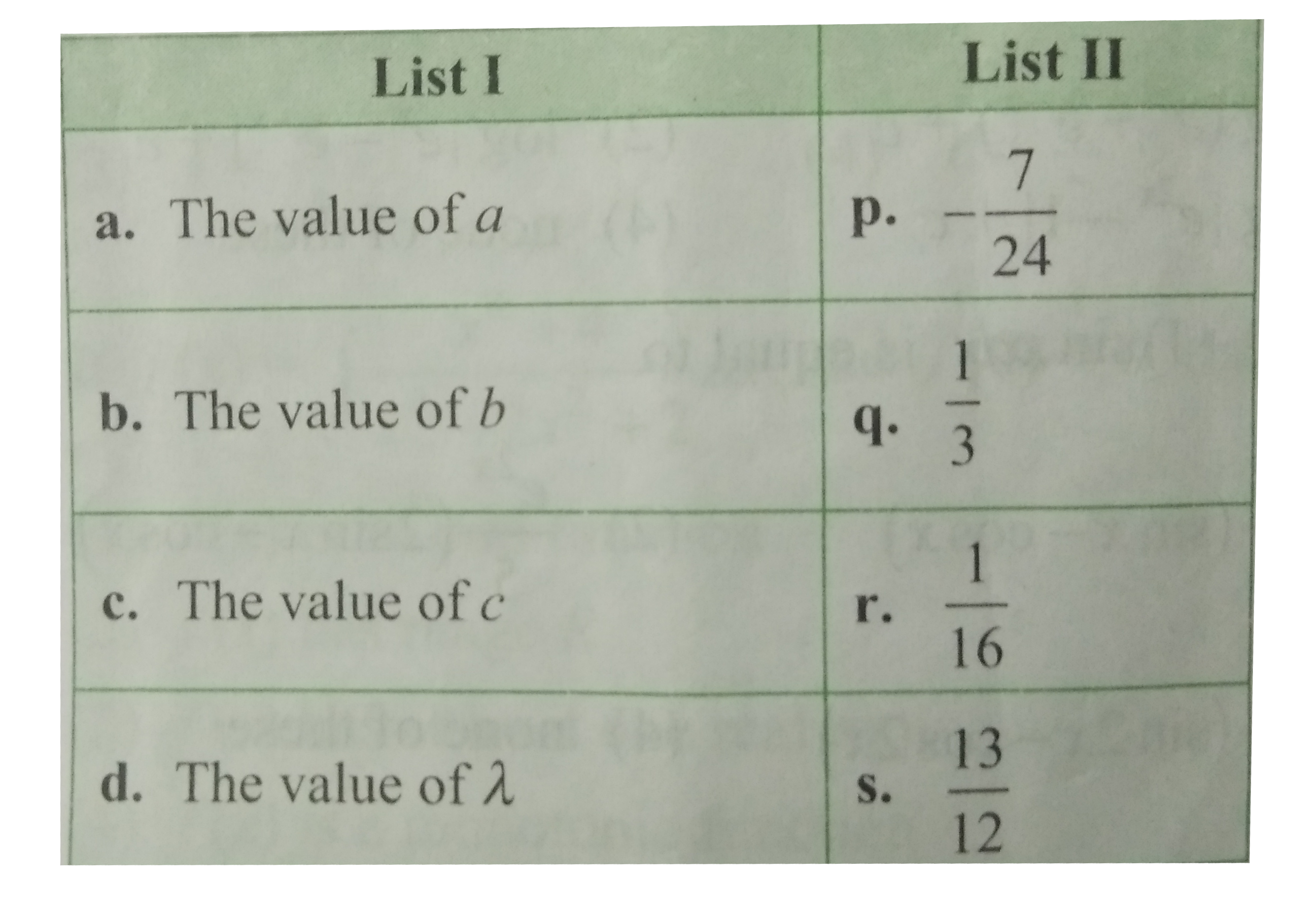
Codes:
abcd(1)qpsr(2)spqr(3)rqps(4)qspr
View SolutionIf ∫8x+73x−2dx=px−q.log(3x−2)+r, then (p,q)≡
View SolutionIf p<0, then the intergral of the form ∫√px2+qx+rdx can be obtained using the formula-
View SolutionThe integral of the form ∫dx(px+q)√ax2+bx+c can be obtained by the substitution-
View SolutionIf ∫(2x+3)x(x+1)(x+2)(x+3)+1dx=−1px2+qx+r+c then 3p−qr=
View Solutionif ∫(2x+3)x(x+1)(x+2)(x+3)+1dx=−1px2+qx+r+c , than 3p−qr
View Solutionযে প্রতিস্থাপন দ্বারা∫dx(px+q)√ax2+bx+c আকারের সমাকল নির্ণয় করা যায়, তা হবে―
View Solutionসমাকলন করো:∫px+q√x2+r2dx
View Solution
