A thin converging lens with focal length projects the image of an object on a screen removed from the lens by a distance . Then the screen was draws closer to the lens by a distance . By what distance should the object be shifted for its image to become sharp again ?
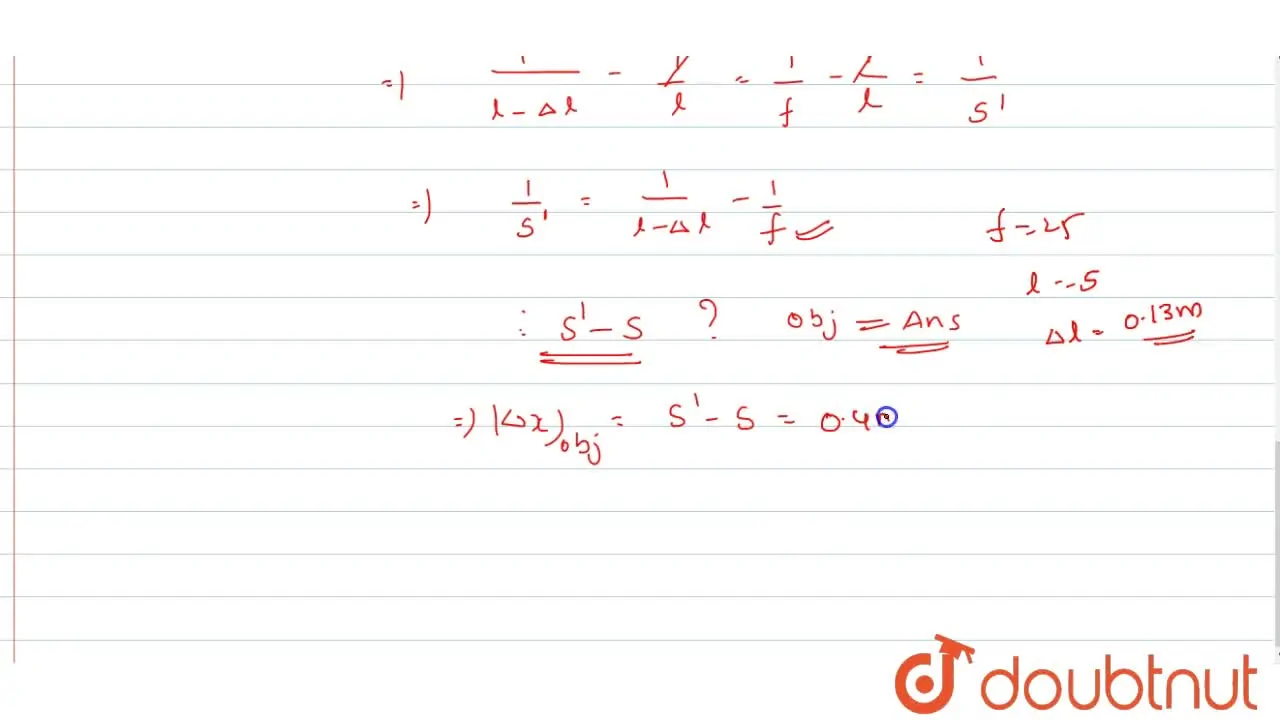
Since the image is formed on the screen, it is real, so for a conbersing lens object is in the incident side.
Let and be the magnitudes of the object distance in the first and second case respectively.
We have the lens formula
(1)
In the first case from Eqn. (1)
or, .
Similarly from Eqn. (1) in the second case
or, .
Thus the sought distance
Related Playlists
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Interference Of Light33videos
OPTICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Diffraction Of Light60videos
OPTICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - MECHANICSEXERCISE - Mechanics Problems92videos
MECHANICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electromagnetic Waves, Radiation36videos
OSCILLATIONS AND WAVES
Similar Questions
A thin converging lens of focal length f = 25 cm forms the image of an object on a screen placed at a distance of 75 cm from the lens. Now the screen in moved closer to the lens by a distance of 25 cm. The distance through which the object has to be shifted so that its image on the screen is sharp again is
View SolutionA thin converging lens of focal length f=25.0 cm forms the image of an object,on the screen,at a distance 5m from the lens. The screen is then drawn closer by a distance of 18cm. By what distance should the object be shifted so that its image on the screen is sharp again?
View SolutionA thin converging lens of focal length 25.0cmforms the image of an object, on the screen, at a distance 5m from the lens. The screen is then drawn closer by a distance of 18cm. By what distance should the object be shifted so that its image on the screen is sharp again?
View SolutionA thin biconvex lens of focal length f=25cm forms a real image of a real object an a screen. The object is separated by 5cm from the lens. The screen is drawn closer to the lens by a distance 18cm. Through what distance (approximately) must the object be shifted so that its image is again formed on the screen ?
View SolutionA convex lens of focal length f produces a real image m times the size of an object, then the distance of the object from the lens is
View SolutionA converging lens has a focal length of 0.12 m. To get an image of unit magnification the object should be placed at what distance from the converging lens ?
View SolutionA convex lens of focal length 16 cm forms a real image double the size of the object. The image distance of the object from the lens is
View SolutionA converging lens of focal length 5 cm is placed at a distance of 20 cm from a screen. How far from the lens should an object be placed so as to form its real image on the screen?
View SolutionA convex lens is used to obtain a magnified image of an object on a screen . The object is at a distance 10 m from the lens . If the magnification is 19. the focal length of the lens is
View SolutionA convex lens forms an image of an object on a screen. The height of the image is 9 cm . The lens is now displaced until an image is again obtained on the screen. Then height of this image is 4 cm . The distance between the object and the screen is 90 cm.
View Solution