A ring is made of a wire having a resistance R0=12Ω. Find the point A and B, as shown in the figure, at which a current carrying conductor should be connected so that the resistance R of the subcircuit between these points is equal to 83Ω
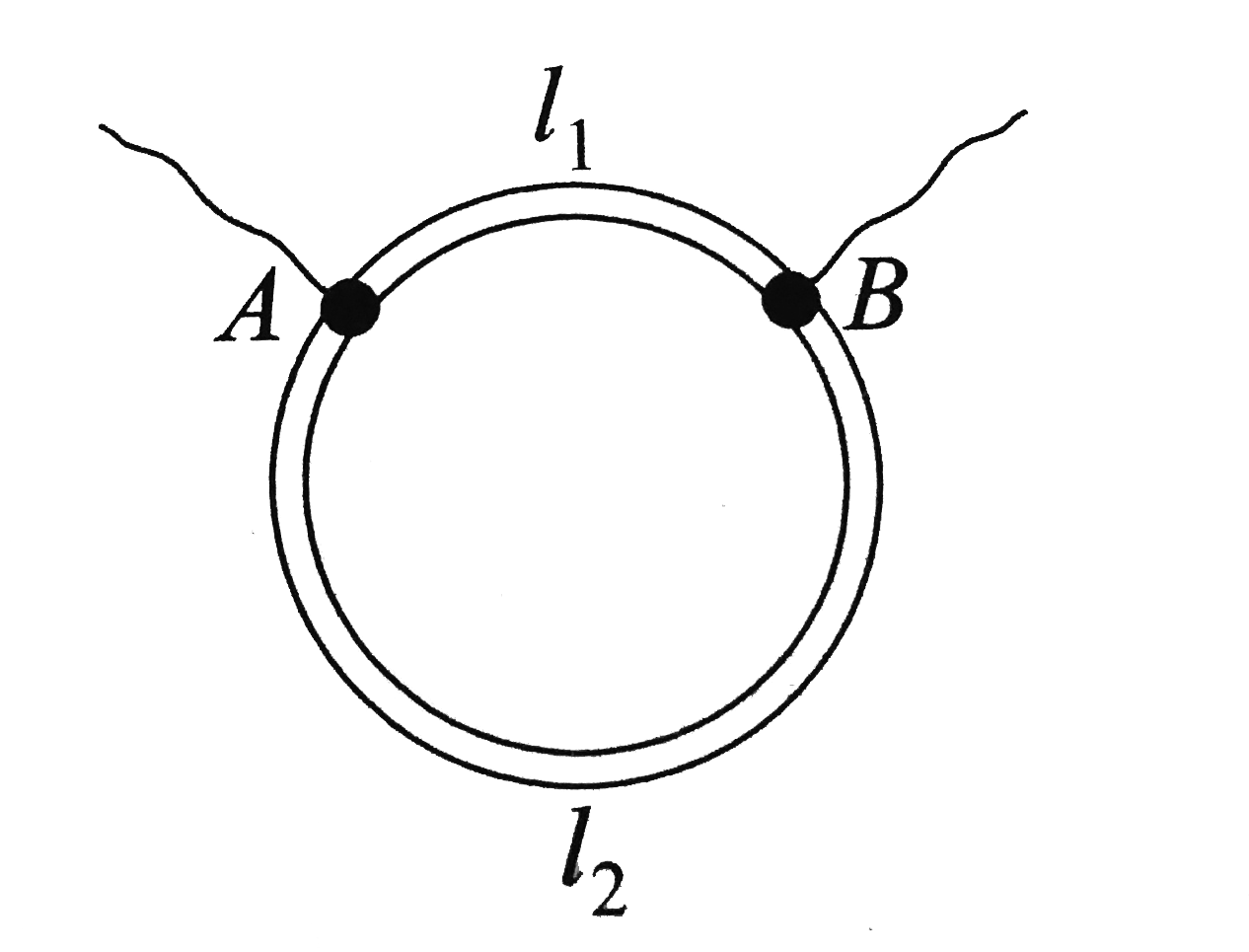
l1l2=56
l1l2=13
l1l2=38
l1l2=12
The correct Answer is:d

Let R1,R2 be the resistances of a wire ring of length l1 and l2 respectively As per question
R1+R2=12orR1=12−R2…(i)
and R1R2R1+R2=83
or R1+R2=83(R1+R2)=83×12=32.....(ii)
Solving (i) and (ii) we get,
R1=4ΩandR2=8Ω
as R∝l, so R1R2=l1l2=48=12
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - PRADEEPCHAPTER - CURRENT ELECTRICITYEXERCISE - Jee (main and advance)
4 Videos
- CURRENT ELECTRICITYBOOK - PRADEEPCHAPTER - CURRENT ELECTRICITYEXERCISE - Interger Type
2 Videos
- CURRENT ELECTRICITYBOOK - PRADEEPCHAPTER - CURRENT ELECTRICITYEXERCISE - Value based
2 Videos
- COMMUNICATION SYSTEMSBOOK - PRADEEPCHAPTER - COMMUNICATION SYSTEMSEXERCISE - MODEL TEST PAPER-2
9 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - PRADEEPCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - Exercise
191 Videos
Similar Questions
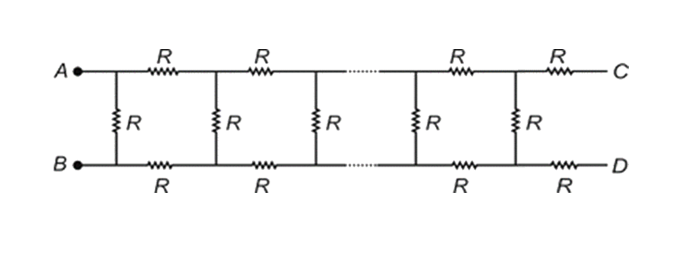
In the circuit shown, the resistance of each of resistor is R=100Ω. Find the nearest integer, which is to be connected points C and D so that the resistance of entire ciruit between A and B does not depend on the number of elementary cells.
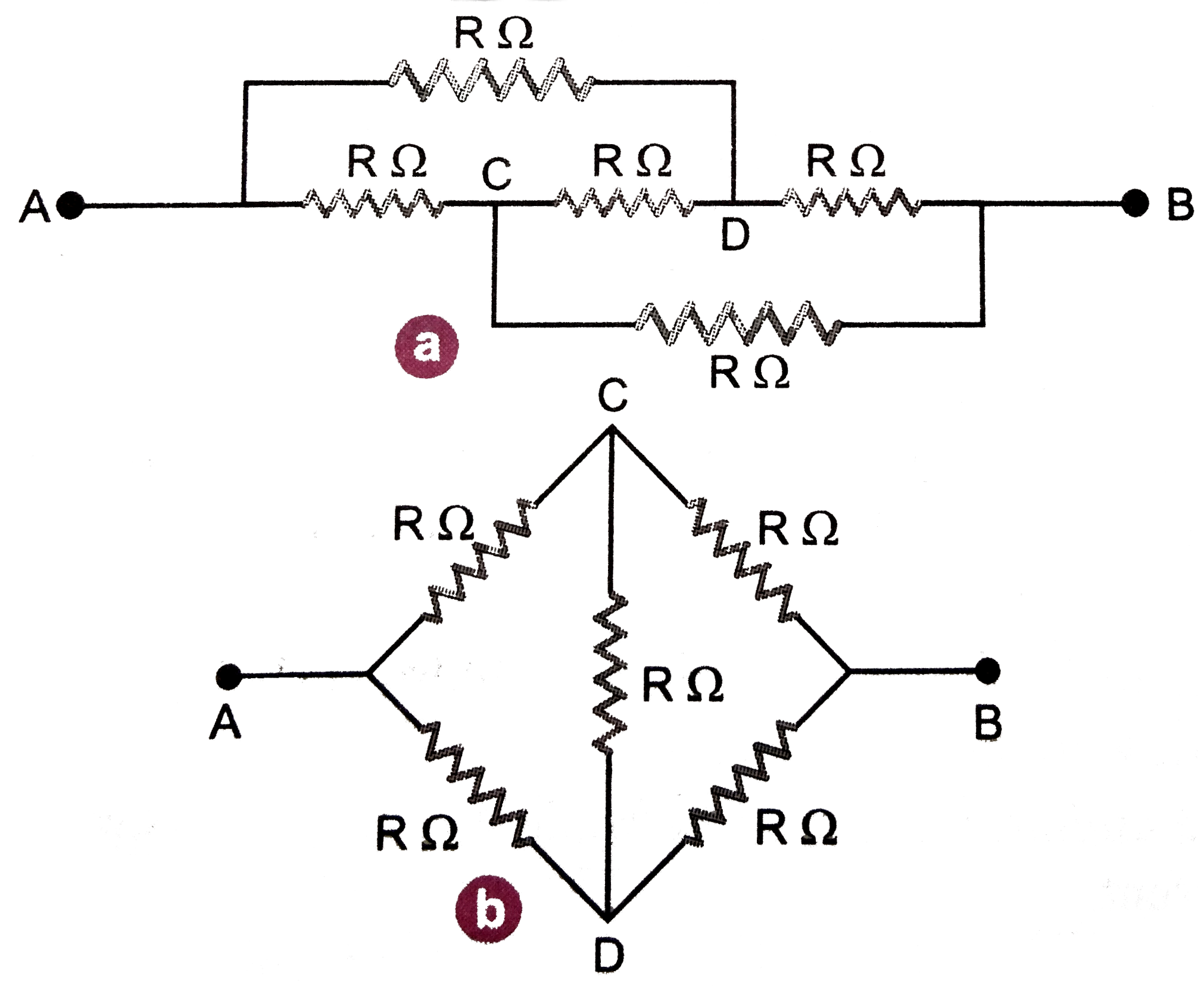
View SolutionFive equal resistors each of RΩ are connected in a network. Calculate the equivalent resistance between the points A and B.
View Solution
Knowledge Check
Five equal resistances of 10Ω are connected between A and B as shown in figure. The resultant resistance
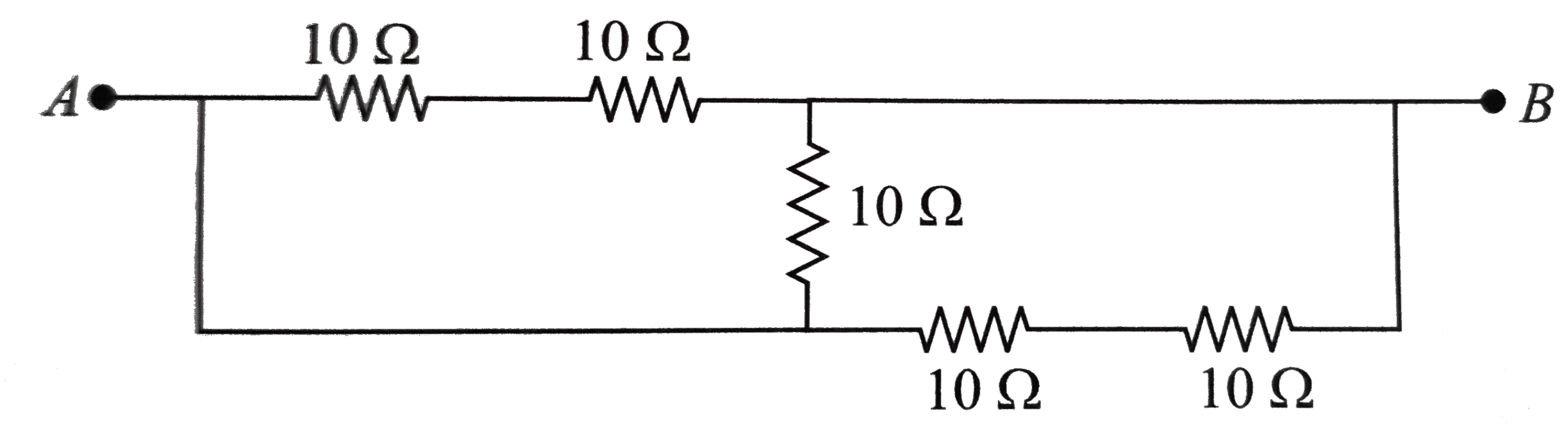 A10ΩB5ΩC15ΩD6Ω
A10ΩB5ΩC15ΩD6ΩA wire has linear resistance ρ (inΩm−1). Find the resistance R between points A and B if the side of the big square is d.
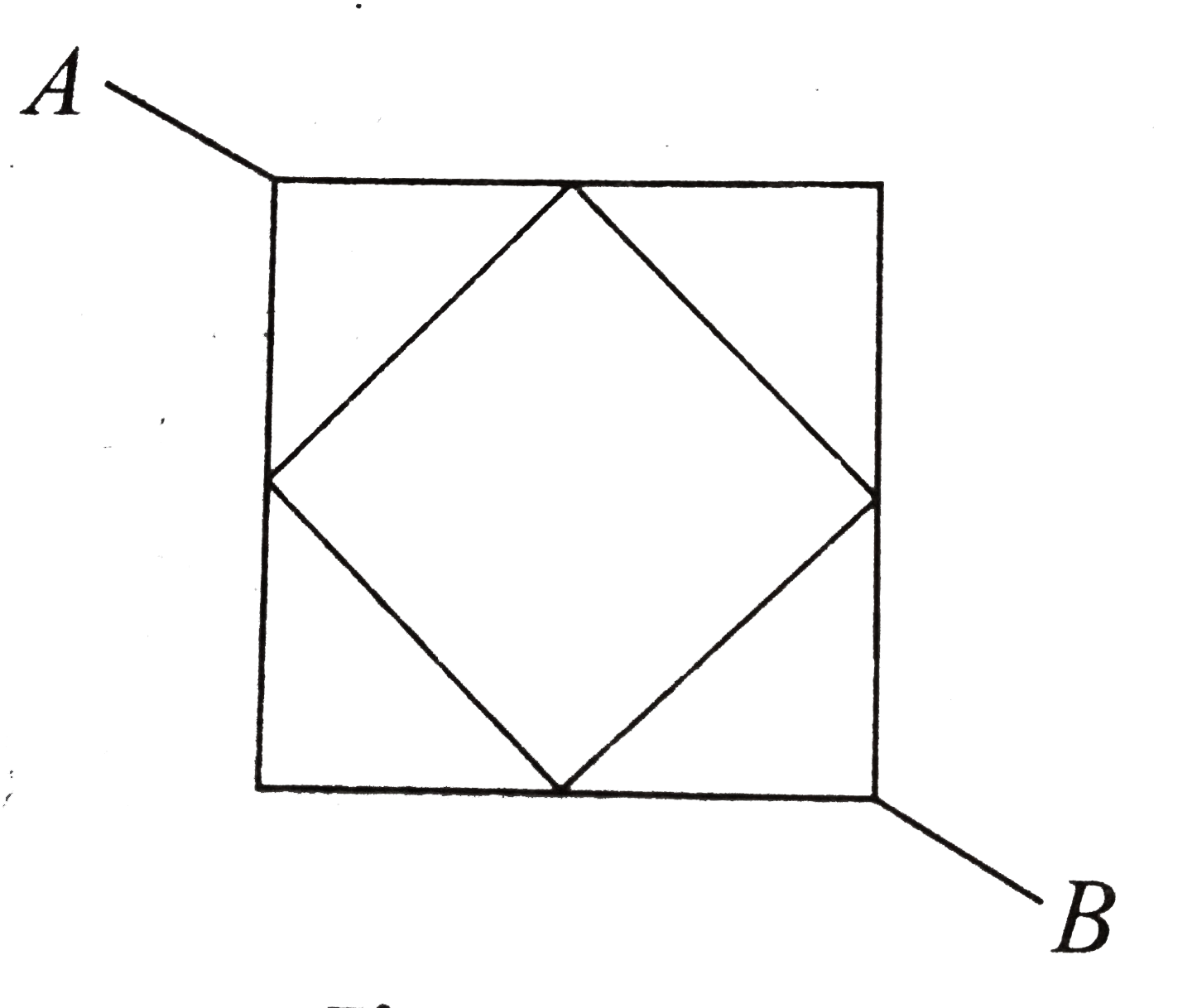 Aρd√2B(√2)ρdC2ρdDnone of these
Aρd√2B(√2)ρdC2ρdDnone of theseIn the network shown in the figure, each of the resistance is equal to 2Ω. The resistance between the points A and B is
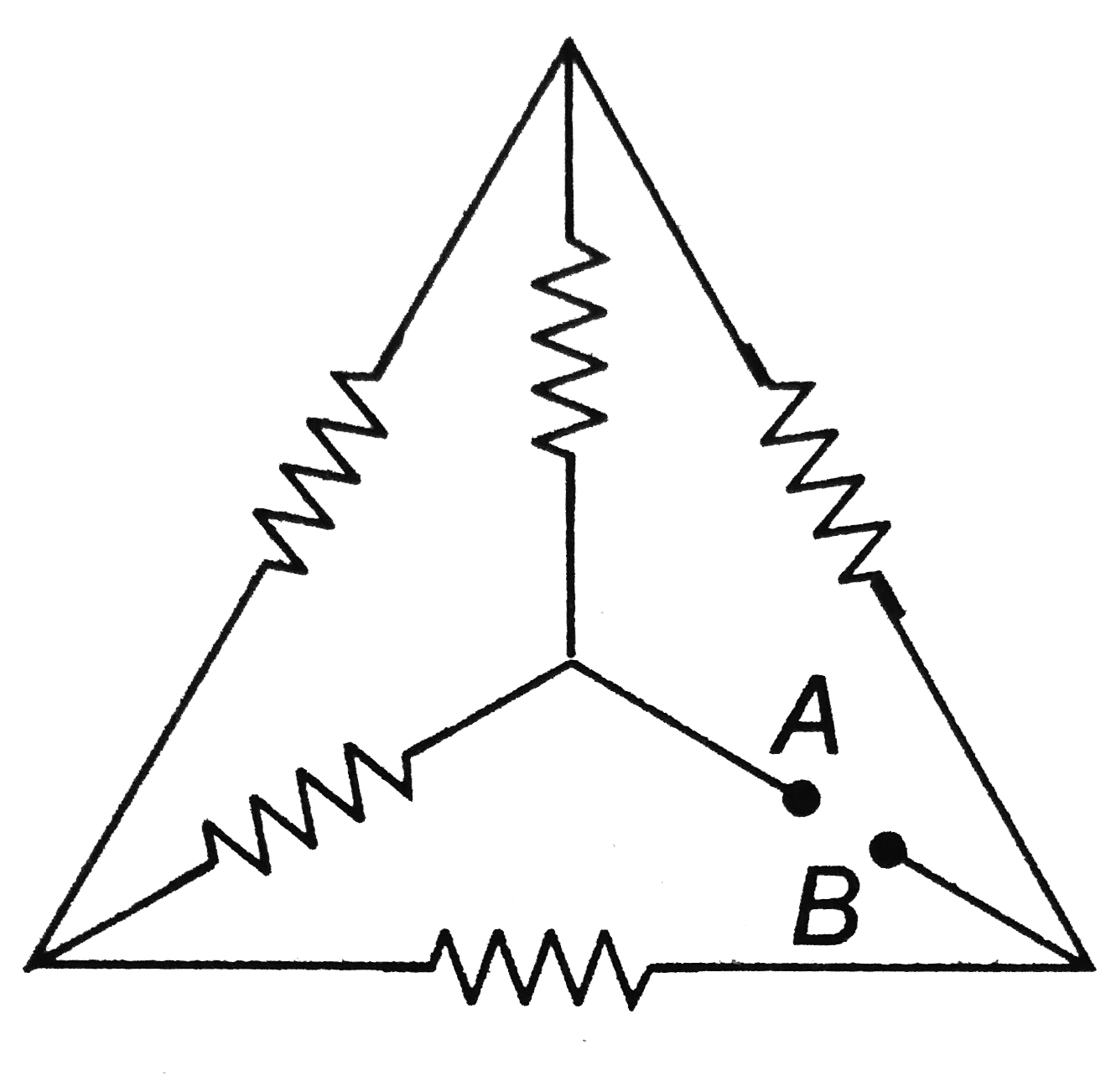 A1ΩB4ΩC3ΩD2Ω
A1ΩB4ΩC3ΩD2Ω
In the network shown in the figure, each of the resistance is equal to 2Ω . The resistance between the point A and B is
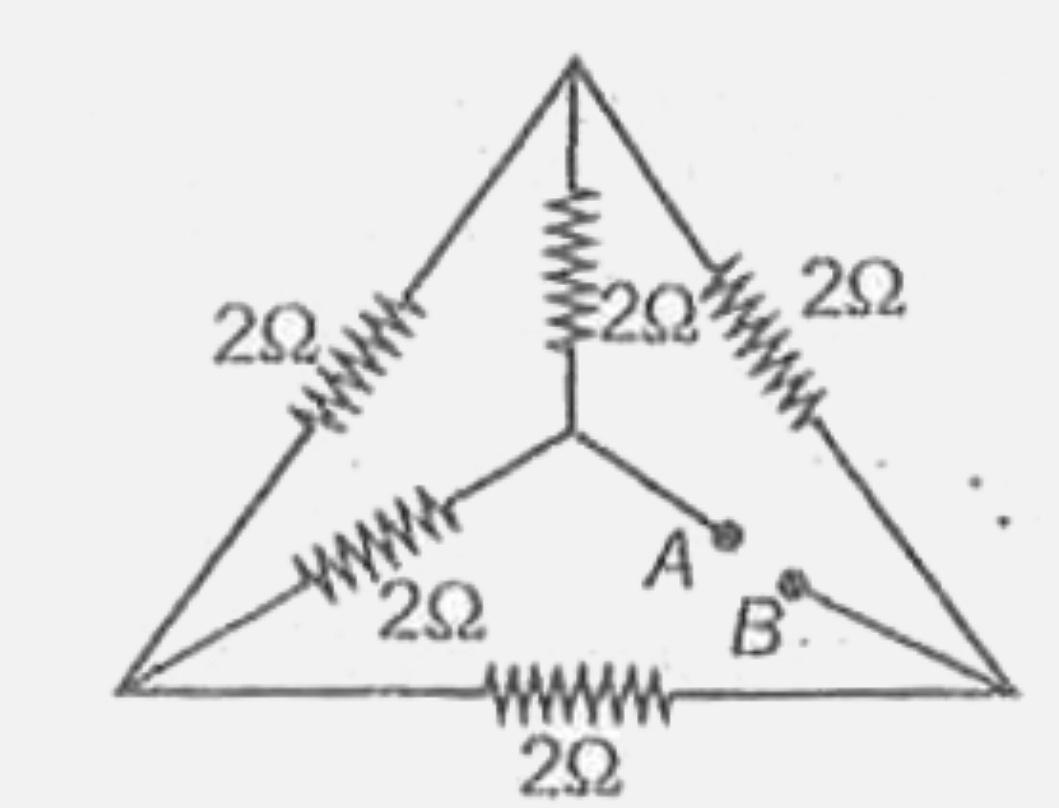
View Solution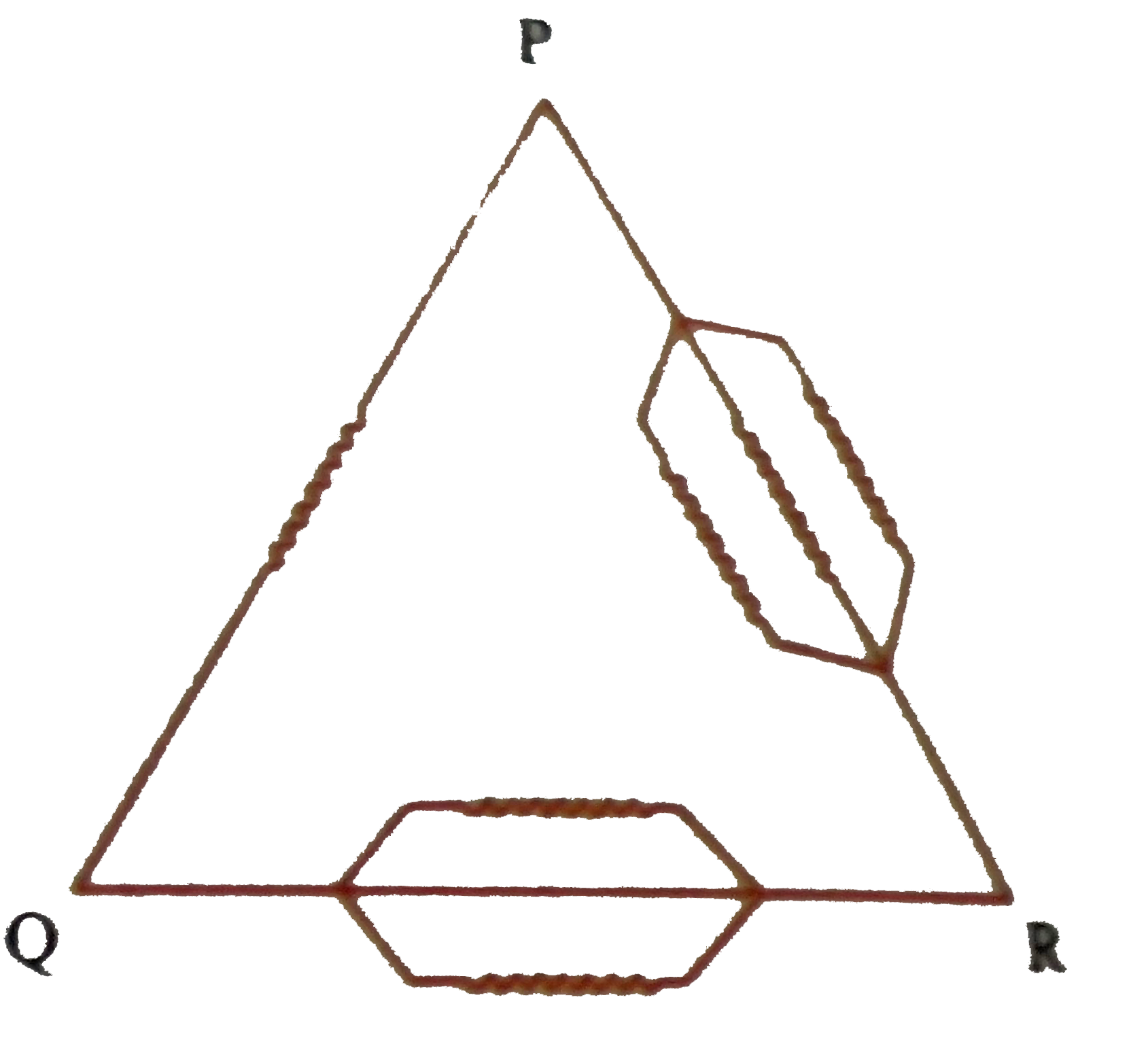
Six equal resistances are connected between points P, Q and R as shown in the figure. Then the net resistance will be maximum between
View SolutionThe effective resistance (in Ω) between P and R of letter A containing resistances as shown in the figure is:
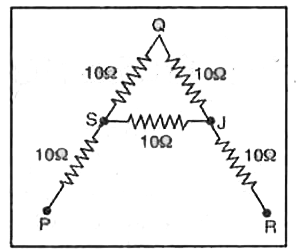
View SolutionA battery of internal resistance 4Ω is connected to the network of resistance as shown . In order that the maximum power can be delivered to the network, the value of R in Ω should be
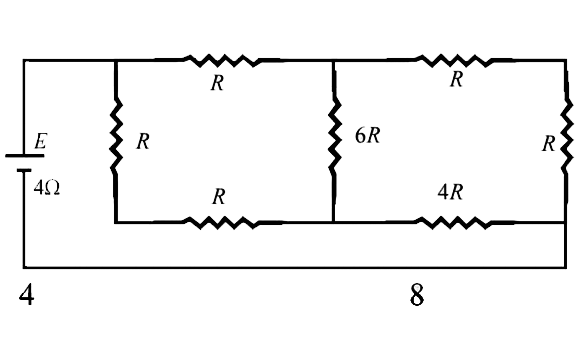 .
.
View SolutionThe network shown in figure is an arrangement of nine identical resistors. The resistance of the network between points A and B is 1.5Ω. The resistance r is
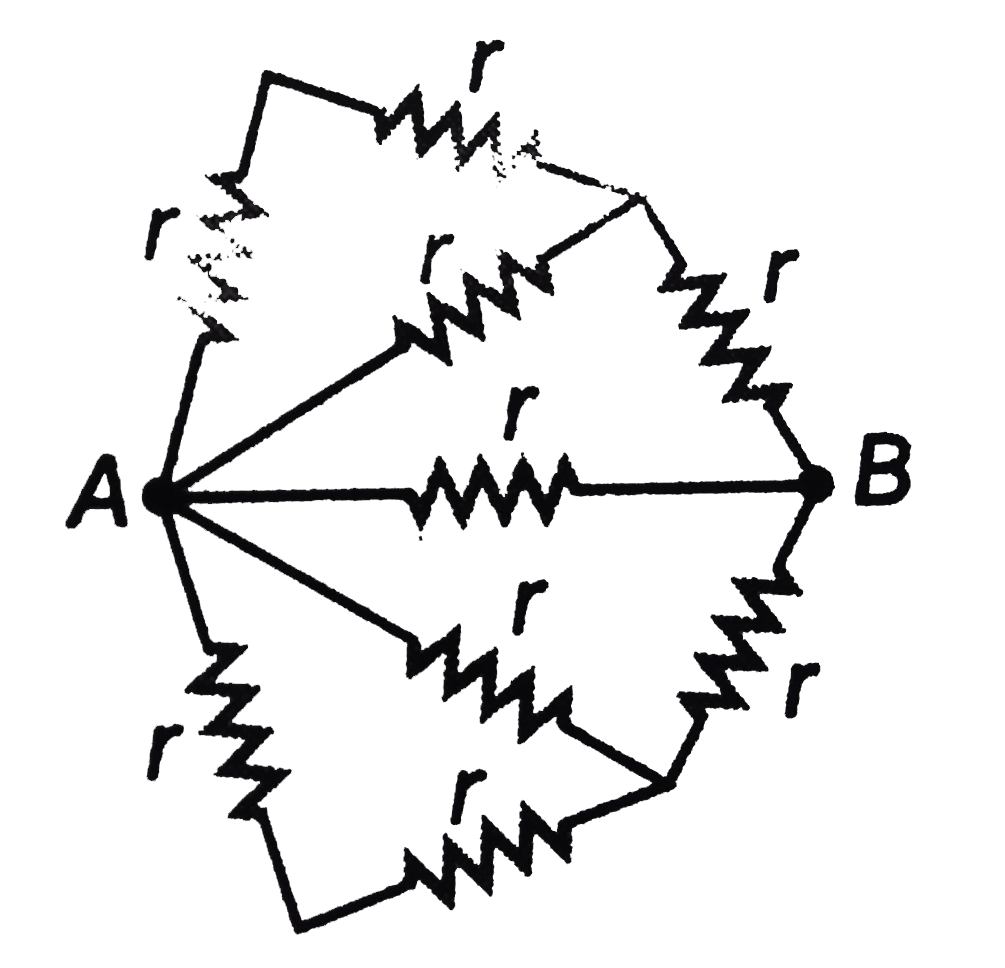
View Solution
