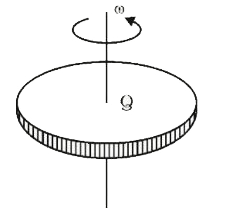
A non-conducting ring of radius r has charge q distributed unevenly over it. What will be the equivalent current if it rotates with an angular velocity $Ω$ ?

Video Solution

Text Solution

Generated By DoubtnutGPT

To find the equivalent current produced by a non-conducting ring of radius $r$ with charge $q$ distributed unevenly over it, rotating with an angular velocity $Ω$ , we can follow these steps:
Step 1: Understand the concept of current
Current ( $I$ ) is defined as the amount of charge ( $Q$ ) passing through a point in a circuit per unit time ( $T$ ). Mathematically, it can be expressed as:
$I = \frac{Q}{T}$
Step 2: Determine the time period of rotation
The time period ( $T$ ) for one complete rotation of the ring can be calculated using the angular velocity ( $Ω$ ). The relationship between angular velocity and time period is given by:
$T = \frac{2 π}{Ω}$
Step 3: Substitute the time period into the current formula
Now, substituting the expression for $T$ into the current formula, we have:
$I = \frac{q}{T} = \frac{q}{\frac{2 π}{Ω}} = \frac{q \cdot Ω}{2 π}$
Step 4: Final expression for equivalent current
Thus, the equivalent current $I$ for the rotating non-conducting ring is:
$I = \frac{q \cdot Ω}{2 π}$
Summary
The equivalent current produced by the rotating non-conducting ring is given by:
$I = \frac{q \cdot Ω}{2 π}$

Updated on:7/8/2024

A conducting ring of radius $R$ has charge $Q$ distributed unevenly over it. If it rotates with an angular velocity $ω$ , the equivalent current will be
Azero
B $Q ω$
C $Q \frac{ω}{2 π}$
D $Q \frac{ω}{2 π R}$
A thin semi-circular ring of radius r has a positive charge q distributed uniformly over it. The net field $\to E$ at the centre O is

A(a) $\frac{q}{4 π^{2} ε_{0} r^{2}} ˆ j$
B(b) $- \frac{q}{4 π^{2} ε_{0} r^{2}} ˆ j$
C(c) $- \frac{q}{2 π^{2} ε_{0} r^{20} ˆ j}$
D(d) $\frac{q}{2 π^{2} ε_{0} r^{2}} ˆ j$
A non conducting disc of radius $R$ , charge $q$ is rotating about an axis passing through its centre and perpendicular to its plane with an angular velocity $ω$ charge $q$ is uniformly distributed over its surface. The magnetic moment of the disc is
A $\frac{1}{4} q ω R^{2}$
B $\frac{1}{2} q ω R$
C $q ω R$
D $\frac{1}{2} q ω R^{2}$