Three identical spheres, each having a charge q and radius R. are kept in such a way that each touches the other two. The magnitude of the electric force on any sphere due to the other two is

A

$\frac{1}{4 π \in_{0}} {(\frac{q}{R})}^{2}$

B

$\frac{1}{4 π \in_{0}} \frac{3}{4} {(\frac{q}{R})}^{2}$

C

$\frac{1}{4 π \in_{0}} \frac{\sqrt{3}}{4} {(\frac{q}{R})}^{2}$

D

$\frac{1}{4 π \in_{0}} \frac{3}{2} {(\frac{q}{R})}^{2}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

Force one sphere $C$ due to $A, F_{A C}$ = Force on sphere
$C$ due to $B, F_{B C} = \frac{1}{4 π \in_{0}} \frac{q^{2}}{{(2 R)}^{2}}$

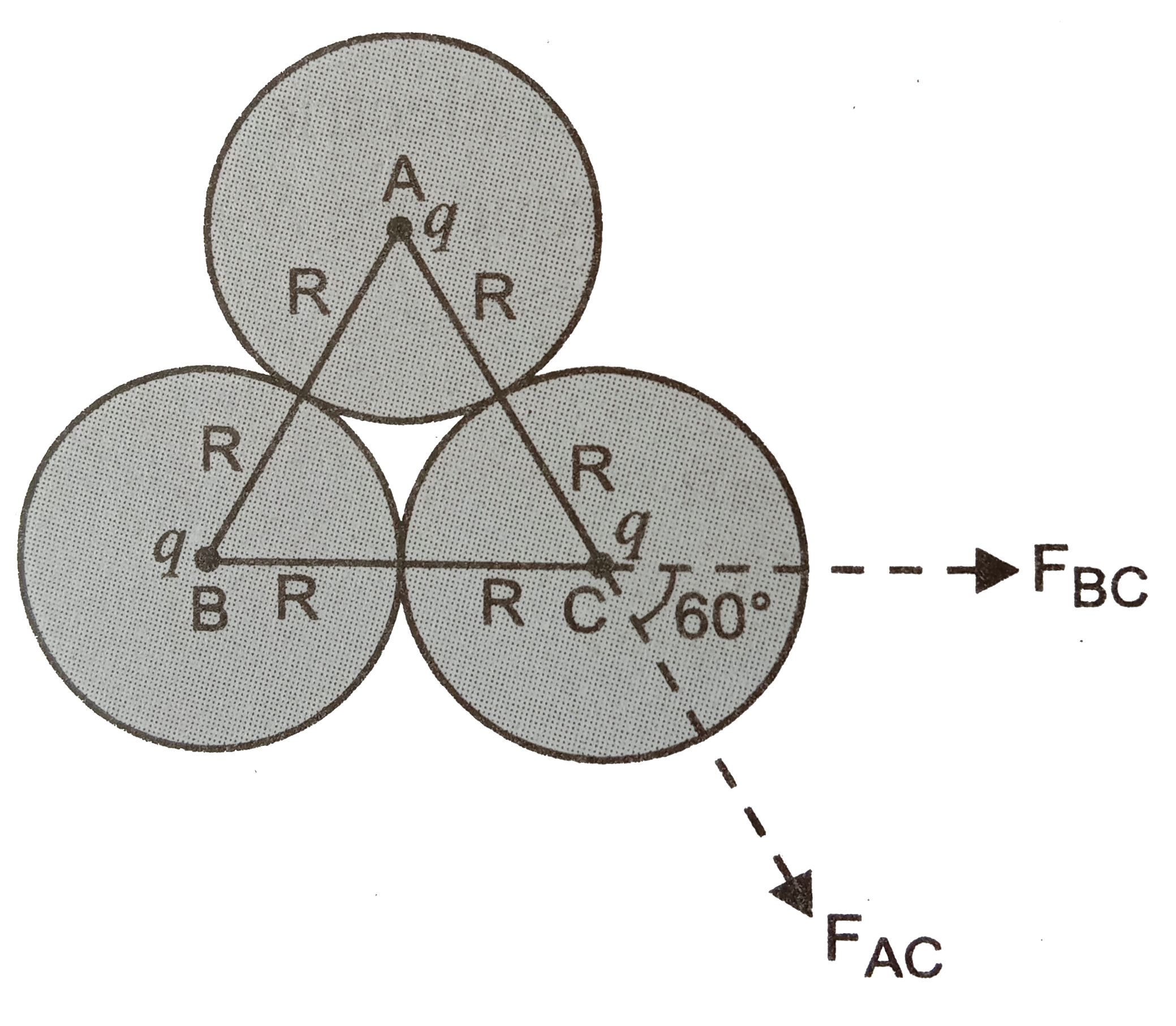
Now $F_{A C}$ and $F_{B C}$ are inclined at $60^{\circ}$ to each other, therefore resultant force on $C$ is
$F_{R} = \sqrt{F_{A C}^{2} + F_{B C}^{2} + 2 F_{A C} F_{B C} {cos 60}^{\circ}}$
$= \sqrt{F^{2} + F^{2} + 2 F^{2} {cos 60}^{\circ}}$
$= \sqrt{3} F$ [Taking $F_{A C} = F_{B C} = F$ ]
$= \frac{1}{4 π \in_{0}} \frac{\sqrt{3}}{4} {(\frac{q}{R})}^{2}$

|

Updated on:21/07/2023

Class 12 PHYSICS ELECTRIC CHARGES AND FIELDS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Three uniform spheres each of mass $m$ and diameter $D$ are kept in such a way that each touches the other two, then magnitudes of the gravitational force on any one sphere due to the other two is
A $\frac{3 G m^{2}}{D^{2}}$
B $\frac{2 \sqrt{3} G m^{2}}{D^{2}}$
C $\frac{\sqrt{3 G m^{2}}}{4 D^{2}}$
D $\frac{\sqrt{3} G m^{2}}{D^{2}}$
Question 2 - Select One
Three uniform spheres, A, B and C each of mass M and radius R are kept in such a way that each sphere touches the other two. What is the magnitude of the gravitational force acting on A due to B and C ?
A $\frac{\sqrt{3} G M^{2}}{4 R^{2}}$
B $\frac{\sqrt{3} G M^{2}}{R^{2}}$
C $\frac{3 G M^{2}}{4 R^{2}}$
D $\frac{\sqrt{3} G M^{2}}{2 R}$
Question 3 - Select One
Three circles of radius 3.5 cm each are placed in such a way that each touches the other two. The area of the portion enclosed by the circles is
A $1.975 c m^{2}$
B $1.967 c m^{2}$
C $19.67 c m^{2}$
D $21.21 c m^{2}$

PRADEEP-ELECTROSTATICS-Exercise

Home
Ask Doubt
Profile