On a smooth table two particles of mass of each, travelling with a velocity v0 in opposite directions, strike the ends of a rigid massless rod length l, kept perpendicular to their velocity. The particles stick to the rod after the collision. Find the tension in rod during subsequent motion.
 .
.
The correct Answer is:2mv20l
As no external torque is acting
:. Its angular momentum will remain conserve
mv0×l2×2=Iω
mv0l=[m(l2)2×2]ω⇒ω=2v0l

Tensiom in the rod will be due to provide centripetal force
T=mω2l2⇒T=m×(2v0l)2×l2
T=2mv2l.
Similar Questions

A uniform rod of mass 3 m and length l is lying on a smooth horizontal surface. Two particle of mass m and 2 m moving with speed 2v0 and v0 in the opposite directly hit the rod perpendicularly at a distance l3 and l6 from the midpoint O of the rod ad stick to it after collision. if the loss in kinetic energy of the system due to collision is n5mv20 find the value of n.
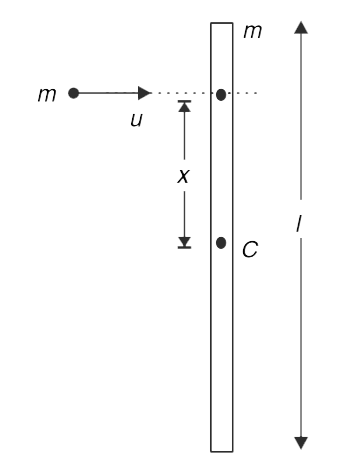
View SolutionA uniform rod of mass m and length l has been placed on a smooth table A particle of mass m, travelling perpendicular to the rod, hits it at a distance x=l√6 from the centre C of the rod. Collision is elastic.
(a) Find the speed of the centre of the rod and the particle after the collision.
(b) Do you think there is a chance of second Collision? If yes, how is the system of particle and stick moving after the second collision?
View Solution
Knowledge Check
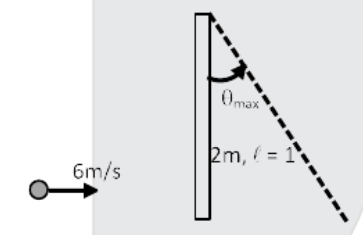
Find maximum angular displacement of rod. if particle sticks after collision.A63∘B69∘C53∘D59∘A rod of mass m and length l in placed on a smooth table. An another particle of same mass m strikes the rod with velocity v0 in a direction perpendicular to the rod at distance x(<l/2) from its centre . Particle sticks to the end. Let ω be the angular speed of system after collision , then
Find the maximum possible value of impulse (by varying x) that can be imparted to the particle during collision. Particle still sticks to the rod.Amv02B2mv03C3mv04D4mv05A rod of mass m and length l in placed on a smooth table. An another particle of same mass m strikes the rod with velocity v0 in a direction perpendicular to the rod at distance x(<l/2) from its centre . Particle sticks to the end. Let ω be the angular speed of system after collision , then
As x is increased from 0 to l/2 , the angular speed ω.Awill continuously increaseBwill continuously decreaseCwill first increase and then decreaseDwill first increase and then decrease
Two particles A and B of mass m each hit the ends of a rigid bar of mass M and length l in-elastically from opposite sides the speeds v and perpendicular to the rod. The bar is kept on a smooth horizontal plane (as shown). Find the linear and angular speed of the system (bar+particle).

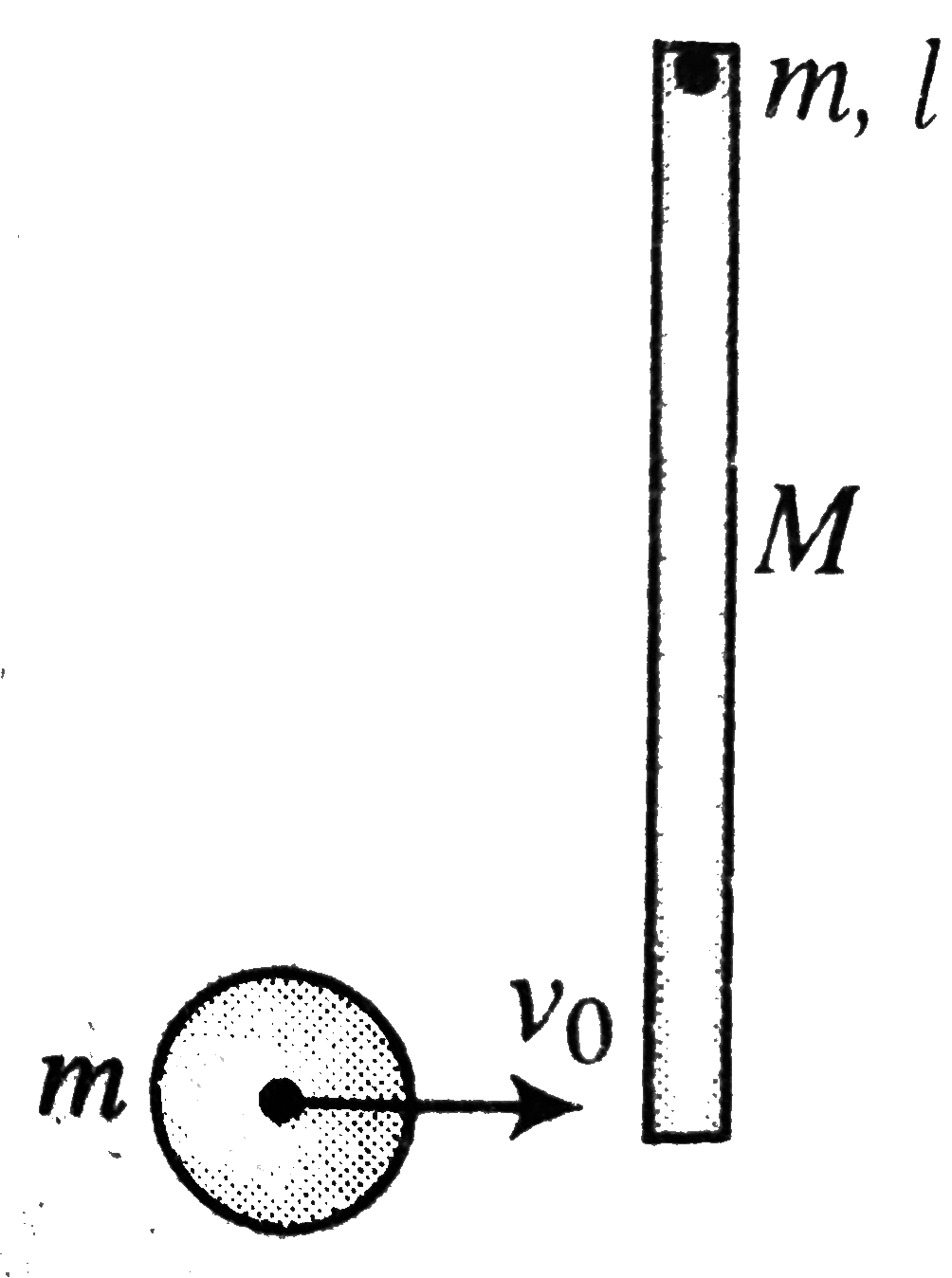
View SolutionA uniform rod of mass M and length l is placed on a smooth- horizontal surface with its one end pivoted to the surface. A small ball of mass m moving along the surface with a velocity v0, perpendicular to the rod, collides elastically with the free end of the rod. Find:
a. the angular velocity of the rod after collision.
b. the impulse applied by the pivot on the rod during collision. (Take M/m=2)
View SolutionSuppose the particle of the previous problem has a mass m and a speed v before the collision and it sticks to the rod after the collision. The rod has a mass M. a. Find the velocity of the particle with respect to C of the system consituting the rod plus the particle. b. Find the velociyt of the particle with respect to C before the collision. c. Find the velocity of the rod with respect to C before the colision. e. find the moment of inertia of the system about the vertical axis through the centre of mass C after the collision. f. Find the velociyt of the centre of mass C and the angular velocity of the system about the centre of mass after the collision.
View SolutionA uniform rod of length l and mass 2m rest on a smooth horizontal table. A points mass m moving horizontally at right angles to the rod with an initial velocity v collides with one end of the rod and sticks to it.
Determine
(a) the angular velocity of the system after collision,
(b) the position of the point on the rod which remains stationary immediately after collision,
(c) the chabge in kinetic energy of the system as a result of the collision.
View SolutionTwo particles, each of mass M and speed v, move as shown. They simultaneously strike the ends of a uniform rod of mass M and length d which is pivoted at its centre. The particles stick to the ends of the rod. Find the ratio of total initial kinetic energy of the two particles to the total loss in kinetic energy in the collision of the two particles with the rod?
View Solution