A pulley systemis attached to a massless board as shown below. The board pivots only at the pivot point. A 10g mass M sits exaxtly in the middle of the board. If the angle θ is 60∘, what is the force F(inN) necessary to hold the board in the position shown.
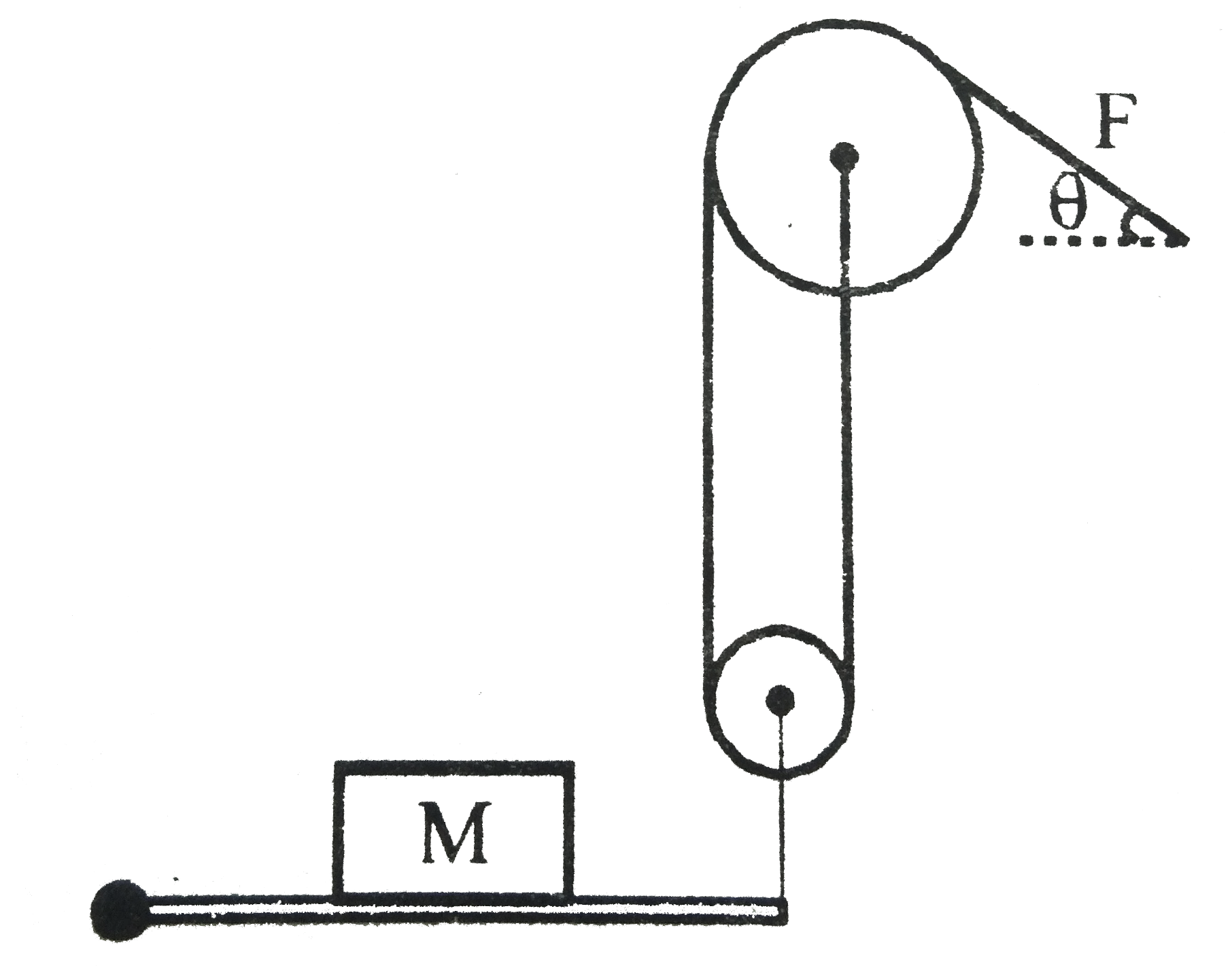 .
.
The correct Answer is:25N
Balancing the torque about pivot
2Fl=Mg(l)2
F=Mg4⇒F=25N.
Similar Questions
A pulley system is attached to a massless board as shown below. The board pivots only at the pivot point. A 10kg mass M sits exactly in the middle of the board.
'(##CEN_KSR_PHY_JEE_C09_E01_023_Q12##)'
View SolutionBoard A is placed on board B as shown. Both boards slide, without moving with respect to each other, along a frictionless horizontal surface at a speed 6 m/ s. Board B hits a resulting board C "head·on". After the collision, board B and C stick together and board A slides on top of board C and stops its motion relative to C in the position shown on the diagram. What is the length (in m) of each board? All three boards have the same mass, size and shape. The coefficient of kinetic friction between boards A and C and between board A & B is 0.3
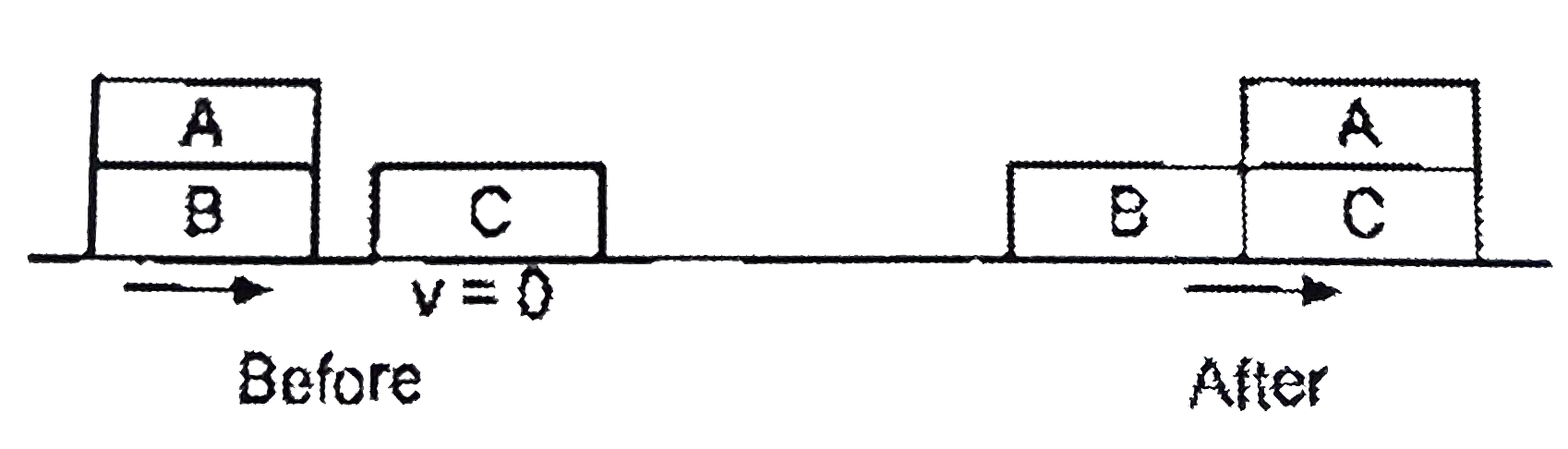
View Solution
Knowledge Check
A mass m is attached to a rigid rod of negligible mass as shown in figure. The system is pivoted at point O and rotates about the indicated z-axis with angular velocity →ω, maintaining a fixed angle θ with the axis.
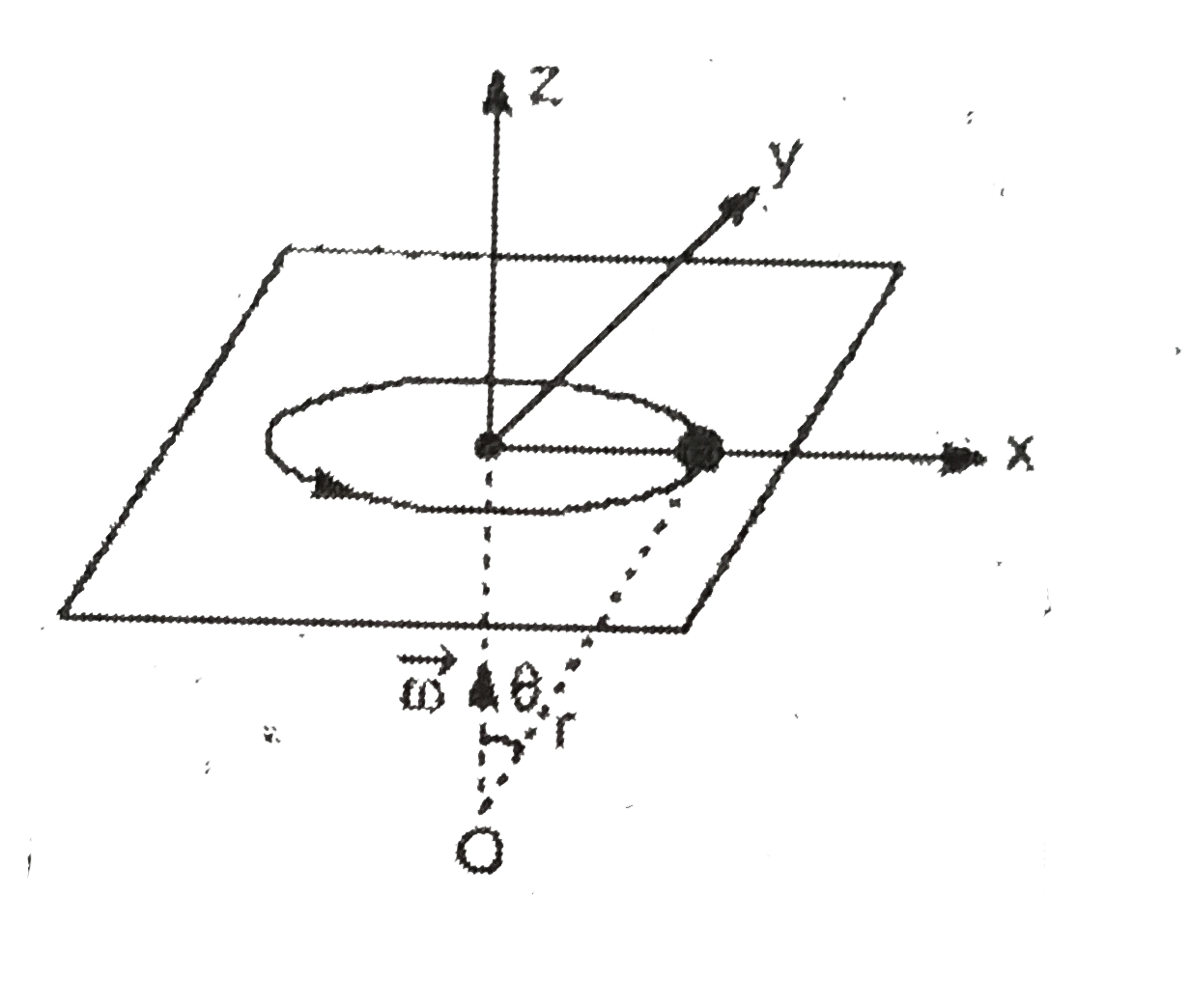 AAngular momentum →L of mass m about pivot is parallel to vector →ωBAngular momentum →L of mass m about pivot is never parallel to →ωC→L0=−mr2ωsinθcosθˆi+mr2ωsin2θˆkDAngular momentum of particle about P is parallel
AAngular momentum →L of mass m about pivot is parallel to vector →ωBAngular momentum →L of mass m about pivot is never parallel to →ωC→L0=−mr2ωsinθcosθˆi+mr2ωsin2θˆkDAngular momentum of particle about P is parallelA uniform bar of mass m is supported by a pivot at its top about which the bar can swing like a pendulum. If a force F is applied perpendicular to the lower end of the bar as shown in figure, what is the value of F in order to hold the bar in equilibrium at an angle (θ) from the vertical
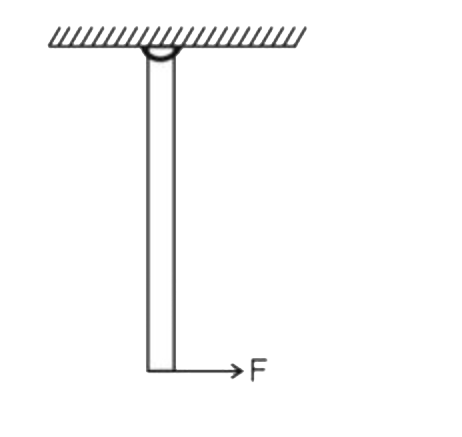 A2mgsinθBmgsinθCmg2sinθDmg2cosθ
A2mgsinθBmgsinθCmg2sinθDmg2cosθTwo painters are working from a wooden hoard 5m long suspended from the top of a building by two ropes attached to the ends of the plank. Either rope can withstand a maximum tension of 1040N. Painter A of mass 80kg is working at a distance of 1m from one end. Painter B of mass 60kg is working at a distance of x in from the centre of mass of the board on the other side. Take mass of the board as 20kg and g=10ms−2. The range of x so that both the painters can work safely is
A13<x<116B0<x<116C0<x<2D13<x<2
One end of a string of length 1.5 m is tied to a stone of mass 0.4 kg and the other end to a small pivot on a smooth vertical board. What is the minimum speed of the stone required at its lower most point so that the string does not slack at any point in its motion along the vertical circle ?
View SolutionIn figure the tension in the diagram string is 60N
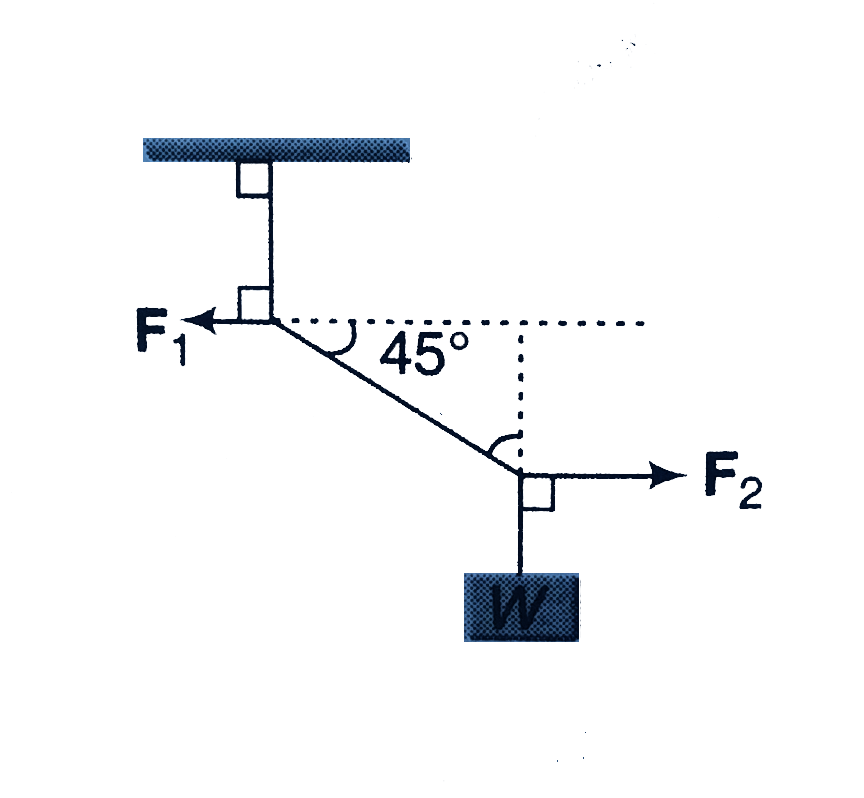
(a) Find the magnitude of the horizontal force F1 and F2 that must be applied to hold the system In the position shown.
(b) What is the weight of the suspended block?
View SolutionA capillary tube with very thin walls is attached to the beam of a balance which is then equalized. The lower end of the capillry is brought in contact with the surface of water after which an additional load of P=0.135gm force is needed to regain equilibrium. If the radius of the capillary is λ10cm then find λ The surface tension of water is 70dyne/cm. (g=9.8m/s2)
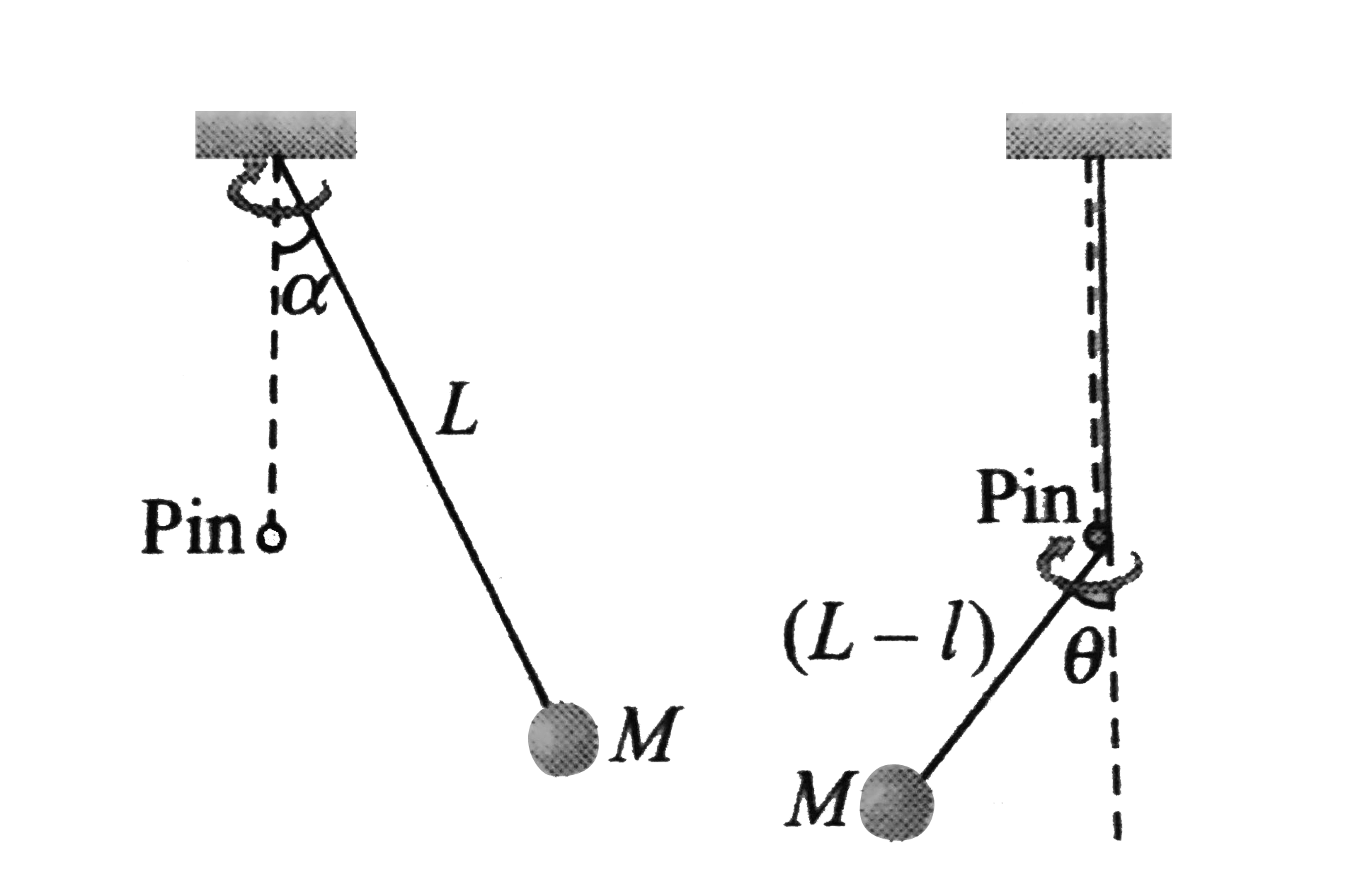
View SolutionA simple pendulum consisting of a mass M attached to a string of length L is released from rest at an angle α. A pin is located at a distance l below the pivot point. When the pendulum swings down, the string hits the pin as shown in figure. The maximum angle θ which the string makes with the vertical after hitting the pin is
View SolutionSTATEMENT-1: One end of a string of length r is tied to a stone of mass m and the other end to a small pivot on a frictionless vertical board. The stone is whirled in a vertical circle with the pivot as the centre. The minimum speed the stone must have, when it is at the topmost point on the circle, so that the string does not slack is √gR.
because
STATEMENT-2: At the topmost point on the circle, the centripetal force is provided partly by tension in the string and partly by the weight of the stone.
View Solution
