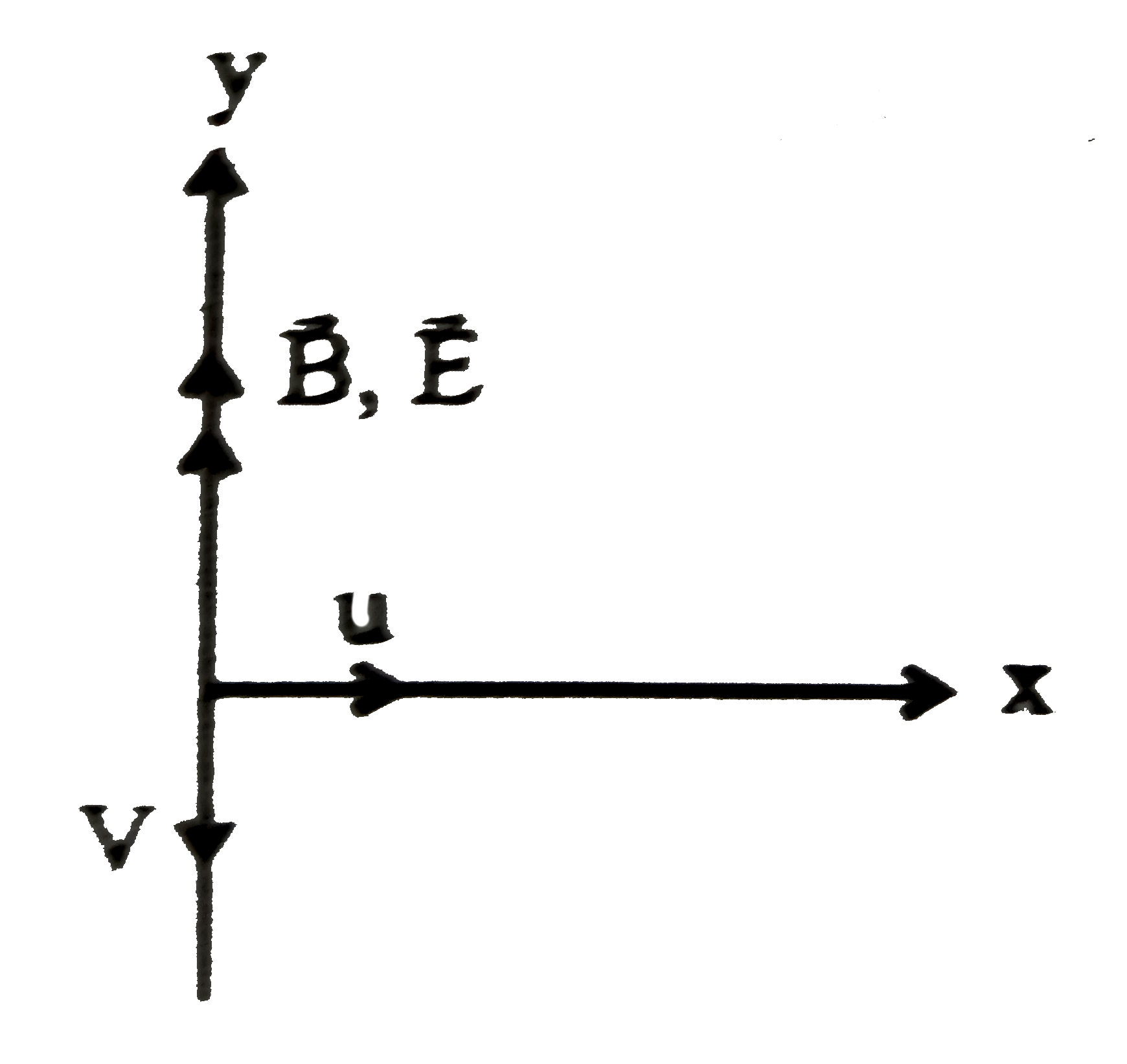
A particle of specific charge (q/m) is projected from the origin of coordinate with initial velocity [ui−vj] unifrom electric magnetic fields exist in the region along the +ydirection, of magnitude E and B The particle will definitely return to the origin once if .
[vB/2πE] is an integer
(u2+v2)1/2[B/πE] is an integer
[vB/πE] in an integer
[uB/πE] is an integer .
The correct Answer is:C
Taking motion along y axis (con by electric field)
α=y0+uyt+12qyt2
0=0−vt+12qEmt2
t=2mvqE
In this time charge must complex one oe more revolution in x -z plane due to magnetic field
T=2πmqB=−2⇒t=nt...(2)
2mv)qE=n×2πmqB⇒vBπE .
Similar Questions
A particle of specific charge α is projected from origin with velocity v=v0ˆi−v0ˆk in a uniform magnetic field B=−B0ˆk. Find time dependence of velocity and position of the particle.
View SolutionA charged particle of specific charge (Charge/mass) α is released from origin at time t=0 with a velocity given as
→v=v0(ˆi+ˆj)
The magnetic field in region is uniform with magnetic induction →B=B0ˆi. Coordinates of the particle at time t=πB0α are:
View Solution
Knowledge Check
A particle of specific charge qm=πC/kg is projected from the origin towards positive x-axis with a velocity of 10m/s in a uniform magnetic field →B=−2ˆkT. The velocity →v of particle after time t=112s will be (in m/s)
A5[ˆi+√3ˆj]B5[√3ˆi+ˆj]C5[√3ˆi−ˆj]D5[ˆi+ˆj]A particle of specific charge q/m=(π)C/kg is projected from the origin towards positive x-axis with a velocity of 10m/s in a uniform magnetic field →B=−2ˆK Tesla. The velocity →V of the particle after time t=1/6 s will be
A(5ˆi+5√3ˆj)m/sB10ˆjm/sC(5√3ˆi+5ˆj)m/sD−10ˆjm/sA particle of charge 'q' and mass 'm' is projected from the origin with velocity (u0ˆi+v0ˆj) in a gravity free region where uniform electric field −E0ˆi and uniform magnetic field −B0ˆi exist. Find the condition so that the particle would return to origin at least for once .
Aμ0B02πE0 is an integerB√u20+v20B0πE0 is an integerCv0B0πB0 is an integerDμ0B0πE0 is an integer
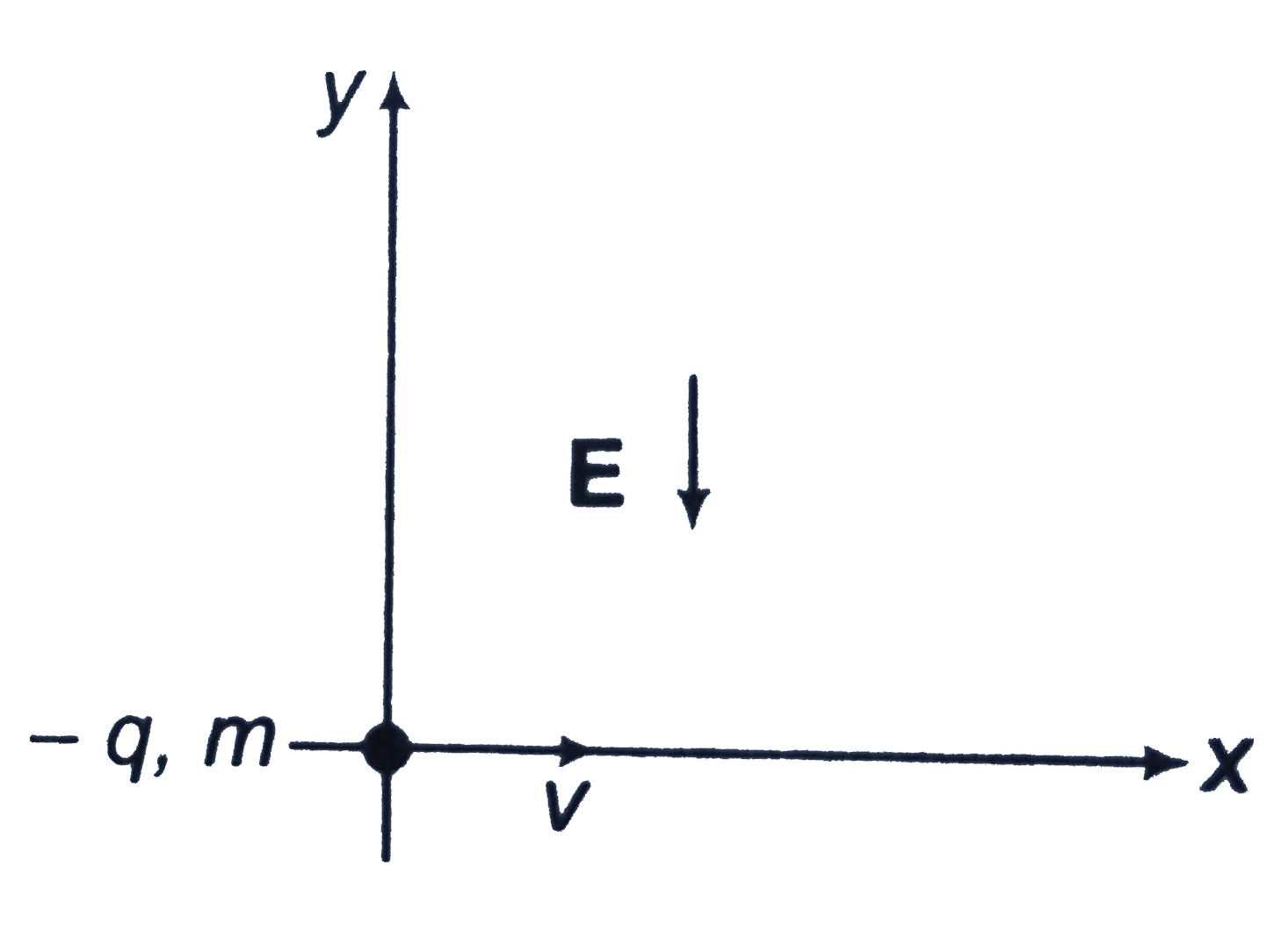
A particle of mass m and charge −q is projected from the origin with a horizontal speed v into an electric field of intensity E directed downward. Choose the wrong statement. Neglect gravity
View SolutionA particle of charge q0 and of mass m0 is projected along the y-axis at t=0 from origin with a velocity V0. If a uniform electric field E0 also exist along the x-axis, then the time at which debroglie wavelength of the particle becomes half of the initial value is:
View SolutionA particle is released from the origin with a velocity vˆe(i). The electric field in the region is Eˆe(i) and magnetic field is Bˆk. Then
View SolutionTwo particles of charges +Q and −Q are projected from the same point with a velocity v in a region of unifrom magnetic filed B such that the velocity vector makes an angle 0 with the magnetic filed Their masses are M and 2M respectively Then, they will meet again for the first time at a point whose distane from the point of projection is .
View SolutionA charged particle of specific charge (charge/mass) α released from origin at time t=0 with velocity →v=v0(ˆi+ˆj) in uniform magnetic field →B=B0ˆi. Coordinates of the particle at time t=π/(B0α) are
View Solution
