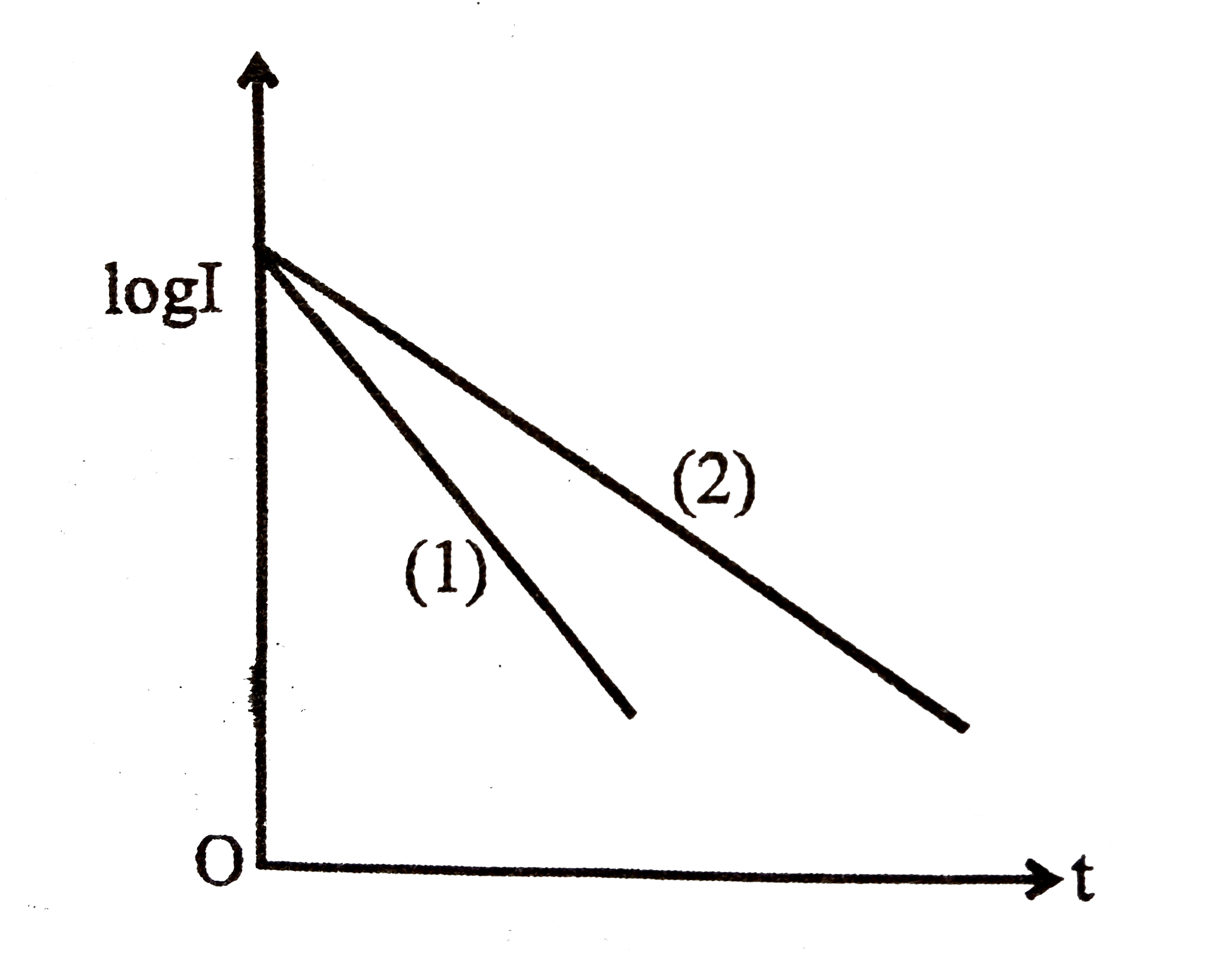
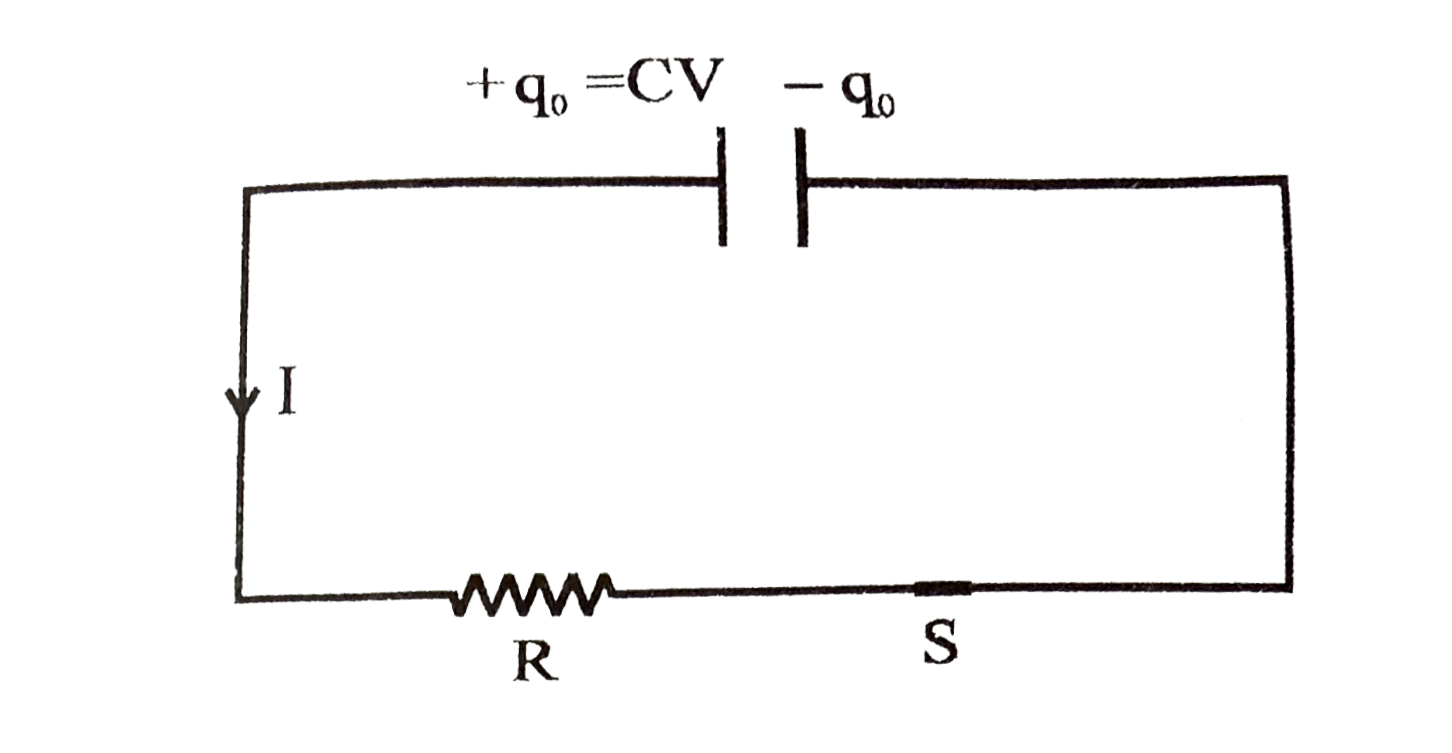
A capacitor of capacity C is charged to a steady potential difference V and connected in series with an opne key and a pure resistor 'R'. At time t=0, the key si closed. If I= current at time t, a plot of logI against 't' is as shown in (1) in the graph. Later one of the parametrs i.e. V,R or C is charged keeping the other two constant, and graph (2) is recored. then-
C is reduced
C is increased
R is reduced
R is increased
The correct Answer is:B
I=VRe−t/RC
logI=−tRC+log(V)R
Since intercept is same so R a V is not charged slope decreases so C increases.
Similar Questions
In the circuit shown, a capacitor charged to a potential difference V0 is connected to an uncharged capacitor through a resistor at t=0, by closing the switch. Find current in the circuit as function of time.
 .
.
View SolutionA capacitor of capacity 2μF is charged to a potential difference of 12V. It is then connected across an inductor of inductance 6μH. What is the current (in A) in the circuit at a time when the potential difference across the capacitor is 6.0V?
View Solution
Knowledge Check
A capacitor of capacitance C1 is charged to a potential difference V and then connected with an uncharged capacitor of capacitance C2 a resistance R. The switch is closed at t = 0. Choose the correct option(s):
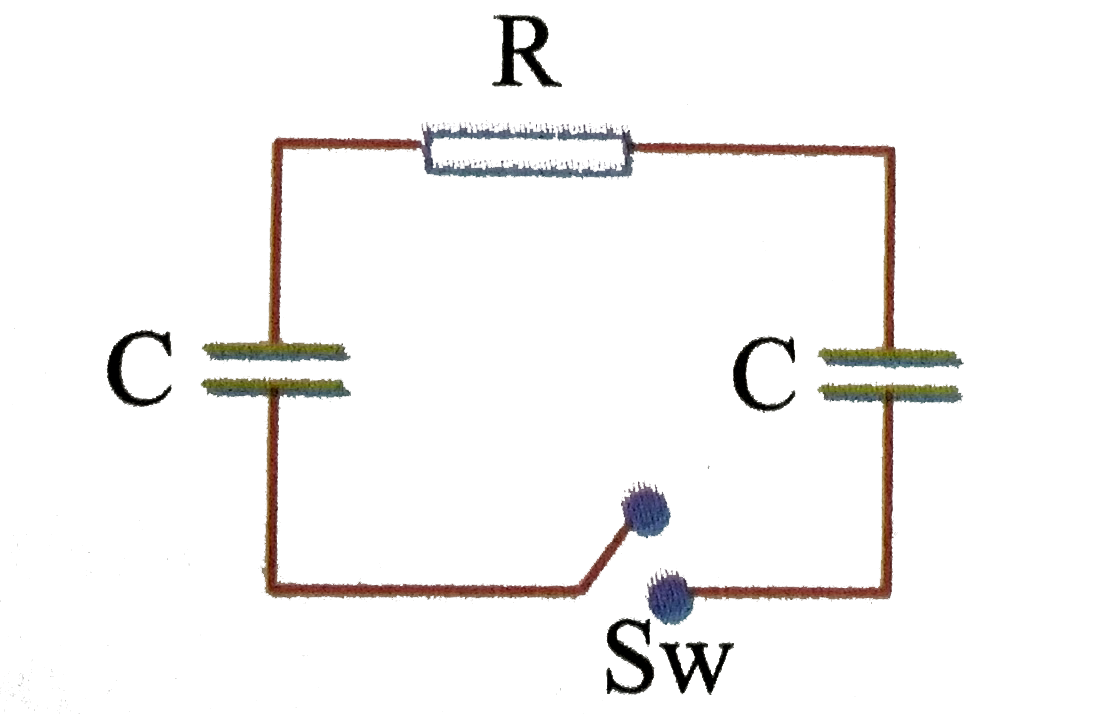
AThe initial current through the resistance is VRBThe current through the resistance as a function of time is i(t)=VRe−tτ where τ=R(C1C2C1+C2)CThe charge on capacitor C1 as a function of time is q1(t)=(C1VC1+C2)(C1+C2e−tτ)DAfter a long time, the potential difference across the capacitor C1is(C1C1+C2)VA capacitor with capacitance C is charged to Q0 and then connected in the circuit shown. When key is closed, what is charge on this capacitor in steady state?
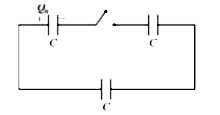 AQ03B2Q03CQ02D3Q02
AQ03B2Q03CQ02D3Q02Two identical capacitors eachof capacitance C are charged to the same potential V and are connected in two circuits (i) and (ii) at t=0 as shown. The charges on the capacitor at t=CR are
 ACVe,CVeBCV, CVCVCe,VCDVC,VCe
ACVe,CVeBCV, CVCVCe,VCDVC,VCe
A capacitor of capacitance C is charged to a voltage V0 and subsequently discharged through a combination of a resistor R and another identical capacitor C in series. Find the current at time t and heat generated in the same time.
View SolutionA capacitor of capacitance as C is given a charge Q. At t=0,it is connected to an ideal battery of emf (ε)through a resistance R. Find the charge on the capacitor at time t.
View SolutionTwo identical capacitors each of capacitance C are charged to the same potential V and are connected in two circuits (i) and (ii) at t=0 as shown. The charged on the capacitor at t=CR are
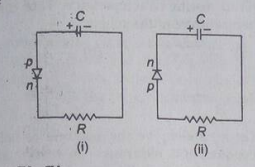
View SolutionTwo identical capacitors each of capacitance C are charged to the same potential V and are connected in two circuits (i) and (ii) at t=0 as shown. The charged on the capacitor at t=CR are

View SolutionA capacitor of capacity 6μF and initial charge 160μC is connected with a key s and resistance as shown in figure, Point M is earthed. If key is closed at t=0, then the current through resistances R1,andR2, at t=16μs are
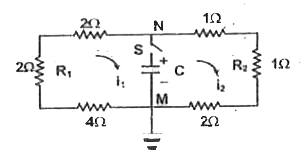
View Solution
