A binary star system consists of two stars of masses M1 and M2 revolving in circular orbits of radii R1 and R2 respectively. If their respective time periods are T1 and T2, then
T1>T2 if R1>R2
T1>T2 if M1>M2
T1=T2
T1T2=(R1R2)3/2
The correct Answer is:C
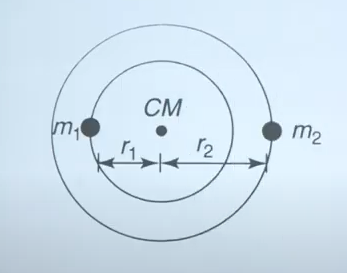
In a binary star system, there are two stars moving under their mutual gravitational force of attraction. Their angular velocities are equal and hence their periodic times are also equal. [∵ω=2πT]
∴T1=T2
Topper's Solved these Questions
- GRAVITATION Book:MARVEL PUBLICATIONChapter:GRAVITATION Exercise:TEST YOUR GRASP -2
Explore 20 Videos
- ELECTROSTATICSBook:MARVEL PUBLICATIONChapter:ELECTROSTATICSExercise:TEST YOUR GRASP
Explore 20 Videos
- INTERFERENCE AND DIFFRACTIONBook:MARVEL PUBLICATIONChapter:INTERFERENCE AND DIFFRACTIONExercise:TEST YOUR GRASP
Explore 20 Videos
Similar Questions
Two stars of masses m1 and m2 form a binary system,revolving around each other in circular orbits of radii r1 and r2 respectively.Time period of revolution for this system is
View SolutionA planet of mass M, has two natural satellites with masses m1 and m2. The radii of their circular orbits are R1 and R2 respectively. Ignore the gravitational force between the satellites. Define v1,L1,K1 and T1 to be, respectively, the orbital speed, angular momentum, kinetic energy and time period of revolution of satellite 1 , and v2,L2,K2 and T2 to be he corresponding quantities of satellite 2. Given m1/m2=2 and R1/R2=1/4, match the ratios in List-I to the numbers in List-II.
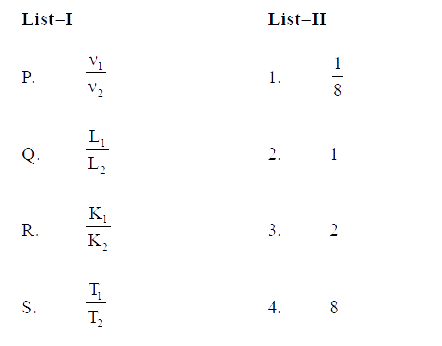
View Solution
Knowledge Check
A system of binary stars of mass mA and mB are moving in circular orbits of radii rA and rB respectively. If TA and TB are at the time periods of masses mA and mB respectively then
ATATB=(rArB)32BTA>TB (if rA>rB)CTA>TB (if mA>mB)DTA=TBTwo satellites A and B of masses m1 and m2(m1=2m2) are moving in circular orbits of radii r1 and r2(r1=4r2), respectively, around the earth. If their periods are TA and TB, then the ratio TA/TB is
A4B16C2D8Two bodies of masses 8 kg and 4 kg are moving in concentric circular orbits of radii r1andr2 respectively . If their time periods are same , the ration of their centripetal accelerations is
Ar1:r2B2r1:r2Cr1:2r2Dr1:r2
Two satellites of masses 3 m and m orbit the earth in circular orbits of radii r and 3 r respectively. The ratio of the their speeds is
View SolutionTwo particles of equal masses are revolving in circular paths of radii r1 and r2 respectively with the same speed. The ratio of their centripetal force is
View SolutionTwo satellite fo masses m and 4m orbit the earth in circular orbits of radii 4r and r respectively. The ratio of their orbital speed is
View SolutionTwo particles of equal masses are revolving in circular paths of radii r1 and r2 respectively with the same speed. The ratio of their centripetal force is,
View SolutionTwo racing cars having masse m1andm2 move in concentric circle of radii r1andr2 respectively. If their angular speed are same , then ratio of their linear speed is
View Solution