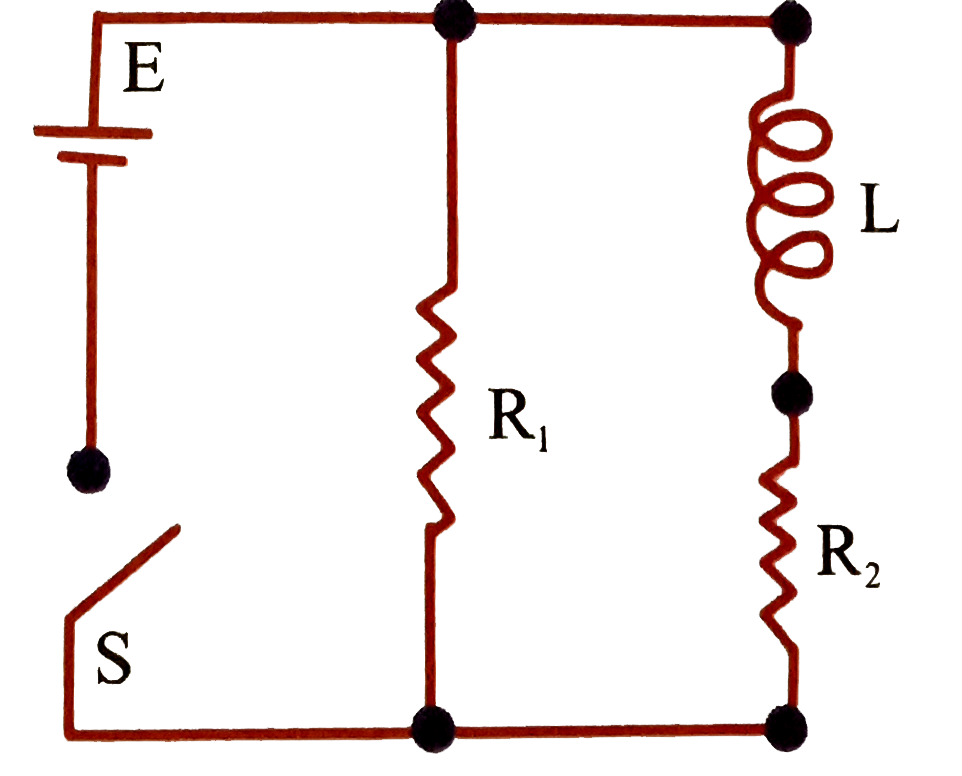
An inductor $(L = 20 m H)$ , a resistor $(R = 100 Ω)$ and a battery $(ɛ = 10 V)$ are connected in series. Find (a) the time constant, (b) the maximum current and (c ) the time elapsed before the current reaches 99% of the maximum value.

Video Solution

Text Solution

Verified by Experts

Here, $L = 20 m H = 20 \times 10^{- 3} H, R = 100 o h m$ .
$E = 10 V$
Time constant, $τ = \frac{L}{R} = \frac{20 \times 10^{- 3}}{100} = 2 \times 10^{- 4} s$
Maximum current, $I_{0} = \frac{E}{R} = \frac{10}{100} = 10^{- 1} A$
Using $I = I_{0} (1 - e^{- t / τ})$
$0.99 I_{0} = I_{0} (1 - e^{- t / τ})$
$∴ e^{- t / τ} = 0.01$ .
Solve to get $t = 0.92 \times 10^{- 3} s$

|

Updated on:21/07/2023

Class 12 PHYSICS ELECTROMAGNETIC INDUCTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
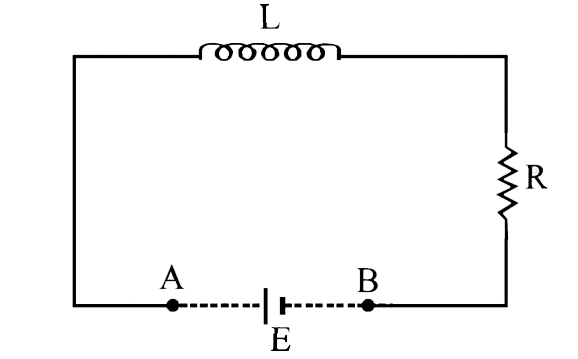
An inductor $(L = 100 m H)$ , a resistor $(R = 100 Ω)$ , and a battery $(W = 100 V)$ are initially connected in series as shown by the figure. After a long time the battery is disconnected after short circuiting the points $A$ and $B$ . The currents in the circuit $1 m m$ after the short circuit is

A $0.1 A$
B $1 A$
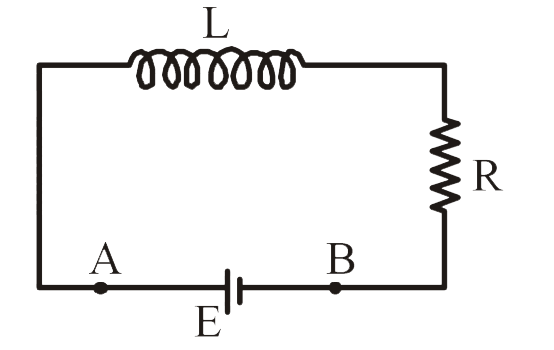
C $\frac{1}{e} A$
D $e A$
Question 2 - Select One
An inductor $(L = 100 m H)$ , a resistor $(R = 100 (Ω))$ and a battery $(E = 100 V)$ are initially connected in series as shown in the figure. After a long time the battery is disconnected after short circuiting the point A and B. The current in the circuit 1 ms after the short circuit is

A $\frac{1}{e} A$
BeA
C0.1A
D $1 A$
Question 3 - Select One
An inductor $20 m H$ , a capacitor $100 μ F$ and a resistor $50 Ω$ are connected in series across a source of emf, $V = 10 sin 314 t$ . The power loss in the circuit is
A $2.74 W$
B $1.13 W$
C $0.43 W$
D $0.79 W$

PRADEEP-ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENT-Exercise

Home
Ask Doubt
Profile