Question

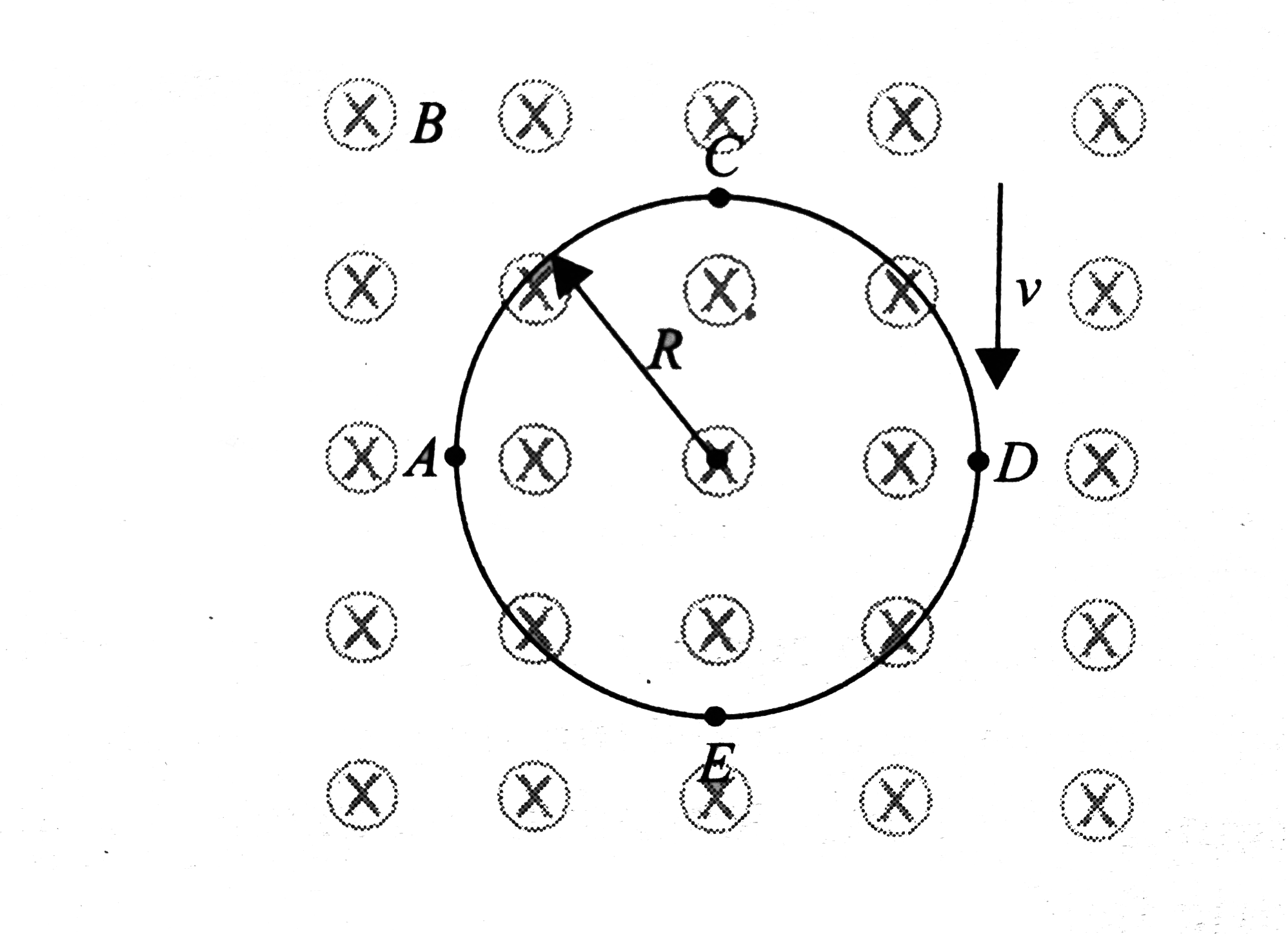
A metallic ring of field. If z is the radius l (ring being horizontal is falling under gravity in a region haivng a magnetic field. If z is the vertical direction, the z-component of magnetic field is $B_z=B_0(1+\lambda z)$. If R the resistance of the ring and if the ring falls with a velocity $\upsilon$, find the energy lost in the resistance If the ring has reached a constant velocity, use the conservation of energy to determine $\upsilon$ in terms of m, B, $\lambda$ and acceleration due to gravity g.

Explanation

To solve the problem step by step, we will analyze the situation involving a metallic ring falling under the influence of gravity in a magnetic field that varies with height. The magnetic field is given by $$B_z=B_0(1+\lambda z)$$. We will find the energy lost in the resistance of the ring and determine the constant velocity $$\upsilon$$ in terms of mass $$m$$, magnetic field $$B$$, $$\lambda$$, and gravitational acceleration $$g$$.

Step 1: Determine the Induced EMF
The induced electromotive force (EMF) in the ring can be calculated using Faraday's law of electromagnetic induction. The EMF \( \mathcal{E} \) is given by:

\( \mathcal{E} = -\frac{d\Phi}{dt} \)

where \( \Phi \) is the magnetic flux through the ring. The magnetic flux \( \Phi \) is given by:

\( \Phi = B_z \cdot A \)

where \( A \) is the area of the ring. For a ring of radius \( l \):

\( A = \pi l^2 \)

Thus, the magnetic flux becomes:

\( \Phi = B_z \cdot \pi l^2 = B_0 (1 + \lambda z) \cdot \pi l^2 \)

Step 2: Calculate the Change in Flux
As the ring falls, \( z \) changes with time. Therefore, we can express the change in flux with respect to time:

\( \frac{d\Phi}{dt} = \pi l^2 \frac{d}{dt}(B_0 (1 + \lambda z)) = \pi l^2 B_0 \lambda \frac{dz}{dt} \)

Since \( \frac{dz}{dt} = v \) (the velocity of the ring), we have:

\( \frac{d\Phi}{dt} = \pi l^2 B_0 \lambda v \)

Thus, the induced EMF becomes:

\( \mathcal{E} = -\frac{d\Phi}{dt} = -\pi l^2 B_0 \lambda v \)

Step 3: Calculate the Induced Current
Using Ohm's law, the induced current \( I \) in the ring can be expressed as:

\( I = \frac{\mathcal{E}}{R} = \frac{-\pi l^2 B_0 \lambda v}{R} \)

Step 4: Determine the Energy Lost as Heat
The energy lost in the resistance \( R \) due to the current \( I \) can be calculated using the formula for power:

\( P = I^2 R \)

Substituting for \( I \):

\( P = \left(\frac{-\pi l^2 B_0 \lambda v}{R}\right)^2 R = \frac{\pi^2 l^4 B_0^2 \lambda^2 v^2}{R} \)

Step 5: Apply Conservation of Energy
At constant velocity, the energy lost in the resistance equals the gravitational potential energy lost by the ring. The gravitational force acting on the ring is \( mg \), and the power due to gravitational potential energy loss is:

\( P_{gravity} = mg v \)

Setting the power lost in the resistance equal to the power due to gravity:

\( \frac{\pi^2 l^4 B_0^2 \lambda^2 v^2}{R} = mg v \)

Step 6: Solve for Velocity \( v \)
We can simplify this equation by dividing both sides by \( v \) (assuming \( v \neq 0 \)):

\( \frac{\pi^2 l^4 B_0^2 \lambda^2 v}{R} = mg \)

Rearranging gives:

\( v = \frac{mg R}{\pi^2 l^4 B_0^2 \lambda^2} \)

Final Result
The constant velocity \( \upsilon \) of the ring in terms of \( m \), \( B_0 \), \( \lambda \), and \( g \) is:

\( \upsilon = \frac{mg R}{\pi^2 l^4 B_0^2 \lambda^2} \)