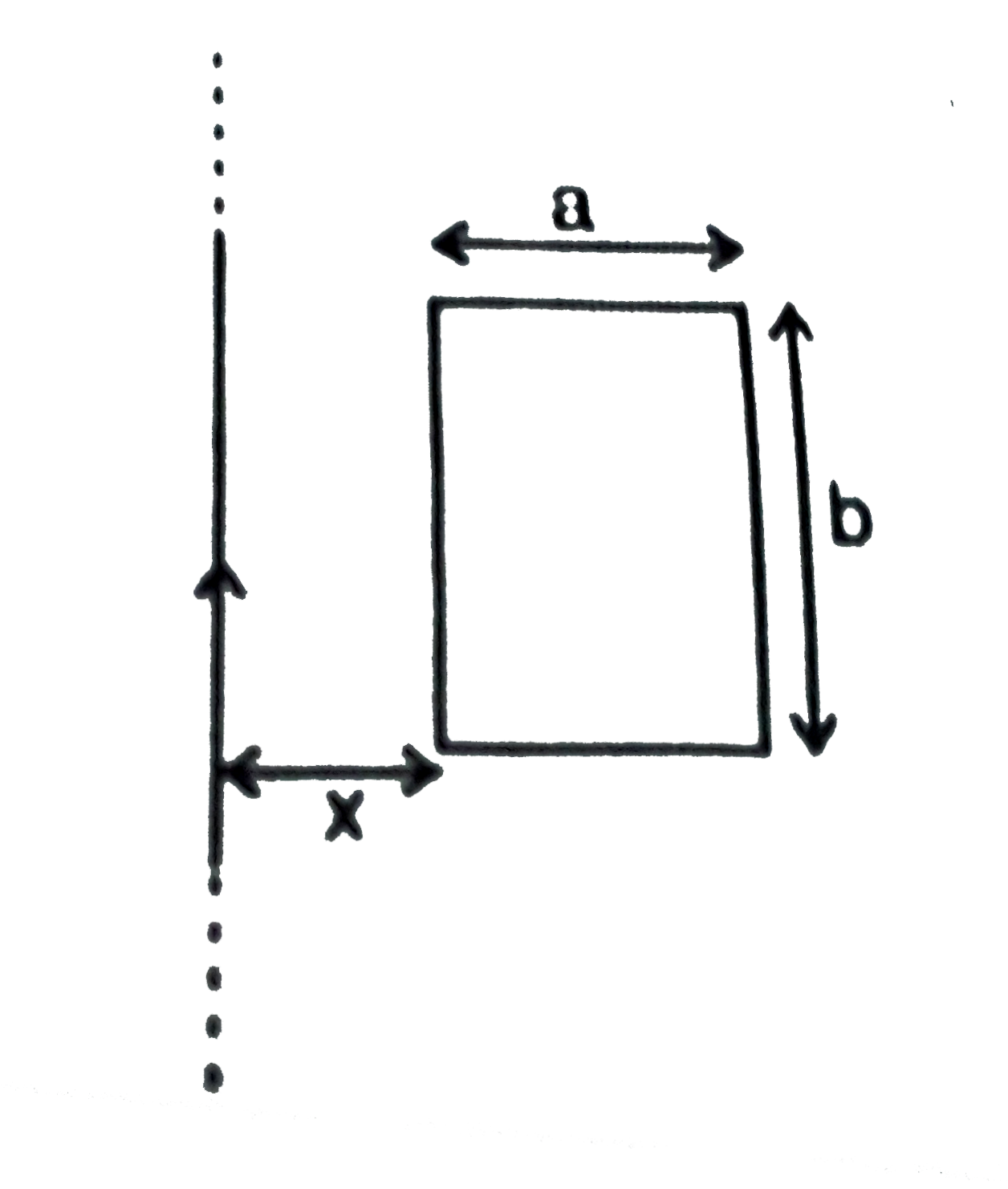
(a) Obtain an expression for the mutual inductance between a long straight wire and a square loop of side a as shown in Fig.
(b) Evaluate the induced emf in loop if the wire carries a current of 50 A and the loop has an instantaneous velocity υ=10ms−1 at the location x = 0.2 m, as shown. Take a = 0.1m and assume that the loop has a large resistance.
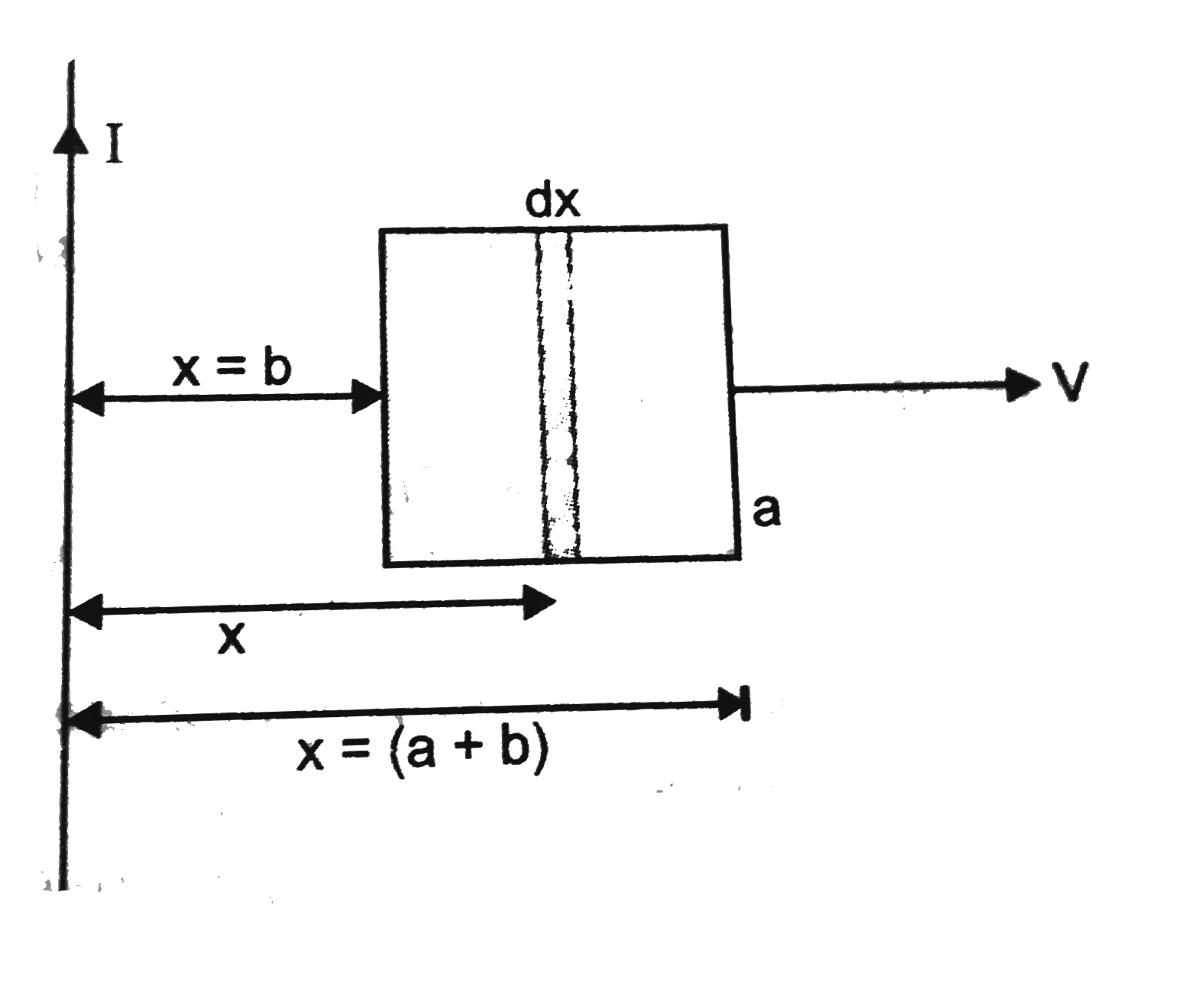
(a) Consider a strip of width dx (of the square loop) at a distance x form the wire carrying current.
Magnetic field due to current carrying wire at a distance x from the wire is B=m0I2πx
Small amount of magnetic flux associated with the strip dϕ=BdA=μ0I2πx(adx)
Magnetic flux linked with the square loop
ϕ=μ0Ia2π∫x=a+bx=bdxx=μ0Ia2π[logex]x=a+bx=b=μ0Ia2πloge(a+bb)=μ0Ia2πloge(ab+1)
As ϕ=MI∴MI=μ0Ia2πloge(ab+1)=μ0a2πloge(ab+1)
(b) Induced emf in the loop
e=Blυ=(μ0I2πx)lυ=4π×10−7×502π×0.2×0.1×10=5×10−5 volt
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTBOOK - PRADEEPCHAPTER - ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTEXERCISE - Solved Examples (b)
1 Videos
- ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTBOOK - PRADEEPCHAPTER - ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTEXERCISE - Short Answer Qusetions
2 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - PRADEEPCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - Exercise
191 Videos
- ELECTROMAGNETIC WAVESBOOK - PRADEEPCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - II Focus multiple choice question
5 Videos
Similar Questions
Find the mutual inductance between the straight wire and the square loop of figure.
View Solution(a) Obtain an expression for the mutual inductance between a long straight wire and a square loop of side a as shown in Fig. 6.06.
(b) Now assume that the straight wire carries a current of 50 A and the loop is moved to the right with a constant velocity, v = 10 m/s. Calculate the induced emf in the loop at the instant when x = 0.2 m. Take a = 0.1 m and assume that the loop has a large resistance.
View Solution
Knowledge Check
The mutual inductance between the rectangular loop and the long straight wire as shown in figure is M.
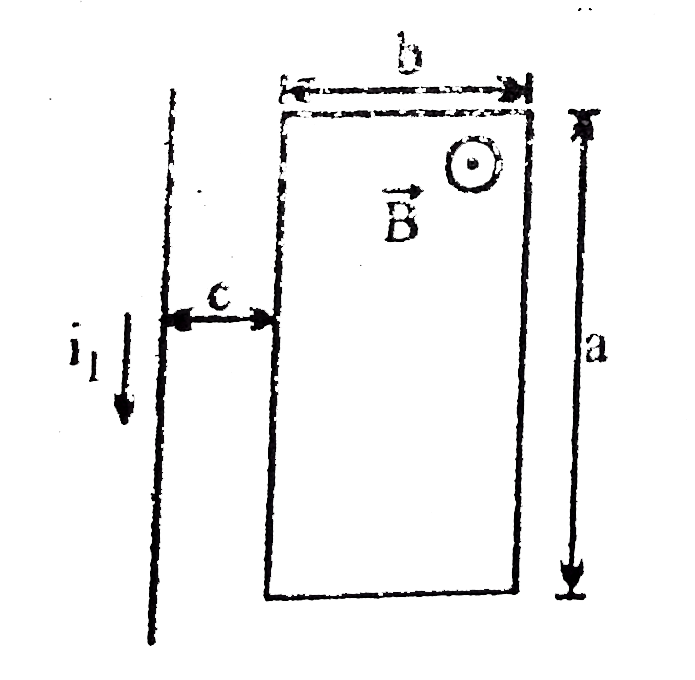 AM=ZeroBM=μ0a2πIn(1+cb)CM=μ0b2πIn(a+cb)DM=μ0a2πIn(1+bc)
AM=ZeroBM=μ0a2πIn(1+cb)CM=μ0b2πIn(a+cb)DM=μ0a2πIn(1+bc)The mutual inductance between the rectangular loop and the long straight wire as shown in figure is M.
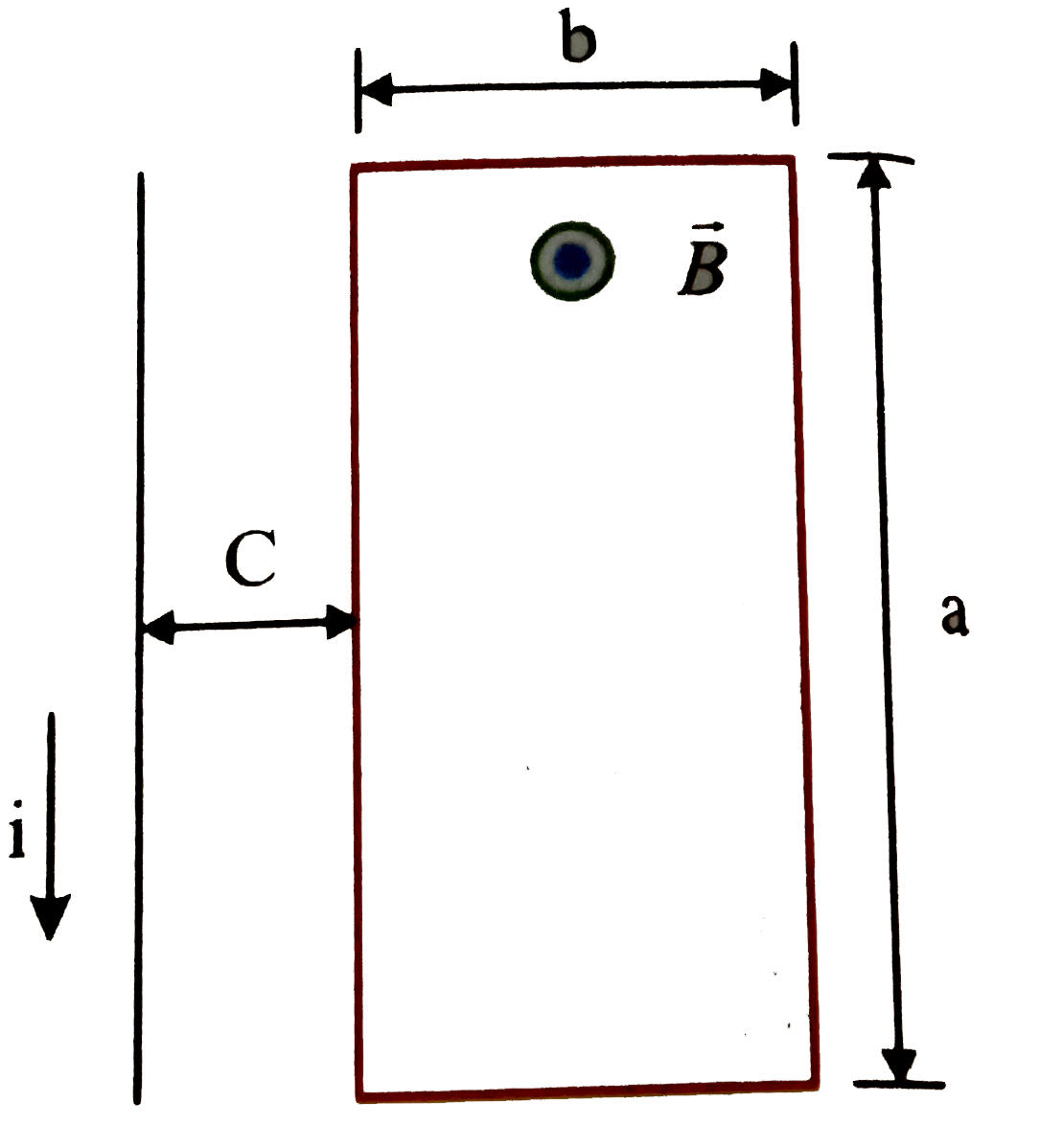 AM= zeroBM=μ0a2πln(1+cb)CM=μ0a2πln(a+bb)DM=μ0a2πln(1+bb)
AM= zeroBM=μ0a2πln(1+cb)CM=μ0a2πln(a+bb)DM=μ0a2πln(1+bb)A loop and an infinite wire are placed side by side as shown in the figure. If the current I in the wire is increased then the induced current in the loop is
 AclockwiseBanticlockwiseCzeroDcannot determine
AclockwiseBanticlockwiseCzeroDcannot determine
Find the mutual inductance of a straight long wire and a rectangular loop, as shown in the figure.
View SolutionThe induced emf in the loop, if the long wire carries a current of 50A and the loop has an instantaneous velocity v=10ms at the location x=0,2m as shown in figure is e microvolt. Caluclate 3ε (Take a=0.1m)
'(##CEN_KSR_PHY_JEE_CO23_E01_038_Q14##)'
View SolutionFigure shows a conducting loop placed near a long straight wire carrying a current i as shown, if the current increases continuously, find the direction of the induced current in the loop.
View Solutiona rectangular loop, as shown in Fig. 3.66, moves away from an infinitely long wire carrying a current I. Find the emf induced in the rectangualr loop.

View SolutionA square loop of side l carries a current i. it is placed as shown in figure. Find the magnetic moment of the loop.
View Solution
