Question

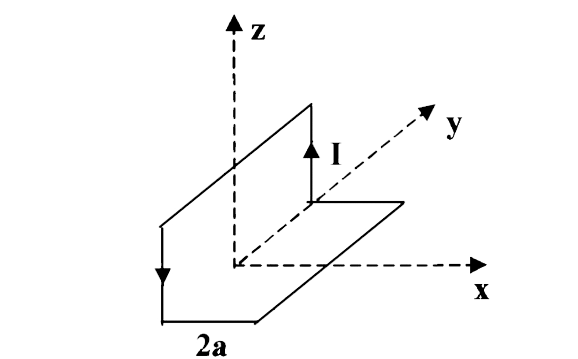
A square loop of side l carries a current i. it is placed as shown in figure. Find the magnetic moment of the loop.

Explanation

To find the magnetic moment of a square loop of side $$l$$ carrying a current $$i$$, we can follow these steps:

Step 1: Understand the Magnetic Moment Formula
The magnetic moment \( \vec{m} \) of a current-carrying loop is given by the formula:
\( \vec{m} = i \cdot \vec{A} \)
where \( \vec{A} \) is the area vector of the loop.

Step 2: Calculate the Area of the Loop
The area \( A \) of a square loop with side length \( l \) is:
\( A = l^2 \)
The direction of the area vector \( \vec{A} \) is perpendicular to the plane of the loop, following the right-hand rule.

Step 3: Determine the Orientation of the Loop
Given that the loop is placed at an angle of \( 30^\circ \) with respect to the x-axis and lies in the YZ plane, we need to resolve the area vector into its components.

Step 4: Find the Components of the Area Vector
The area vector \( \vec{A} \) can be expressed in terms of its components along the x, y, and z axes. Since the loop is tilted, we can find the components as follows:
- The component along the x-axis is \( A_x = A \cos(30^\circ) = l^2 \cdot \frac{\sqrt{3}}{2} \).
- The component along the z-axis is \( A_z = A \sin(30^\circ) = l^2 \cdot \frac{1}{2} \).
- The component along the y-axis is negative since it is directed in the negative y direction.

Thus, we can express the area vector as:
\( \vec{A} = A_x \hat{i} - A_y \hat{j} + A_z \hat{k} = \left(l^2 \cdot \frac{\sqrt{3}}{2}\right) \hat{i} - 0 \hat{j} + \left(l^2 \cdot \frac{1}{2}\right) \hat{k} \)

Step 5: Substitute into the Magnetic Moment Formula
Now substituting \( \vec{A} \) into the magnetic moment formula:
\( \vec{m} = i \cdot \vec{A} = i \left( l^2 \cdot \frac{\sqrt{3}}{2} \hat{i} + 0 \hat{j} + l^2 \cdot \frac{1}{2} \hat{k} \right) \)
This simplifies to:
\( \vec{m} = i \cdot l^2 \left( \frac{\sqrt{3}}{2} \hat{i} + \frac{1}{2} \hat{k} \right) \)

Step 6: Final Expression for the Magnetic Moment
Thus, the magnetic moment of the loop is:
\( \vec{m} = \frac{i l^2}{2} \sqrt{3} \hat{i} + \frac{i l^2}{2} \hat{k} \)

Summary
The magnetic moment of the square loop is given by:
\( \vec{m} = \frac{i l^2}{2} \left( \sqrt{3} \hat{i} + \hat{k} \right) \)