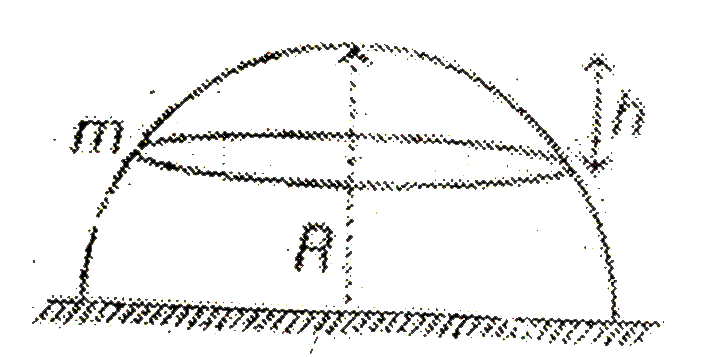
Figure shows a coil of single turn would on a sphere of radius R and mass m. The plane of the coil is parallel to the plane and lies in the equatorial plane of the sphere. What is the value of B if the sphere is in equilibrium when current in the coil is I?

Video Solution

Text Solution

Verified by Experts

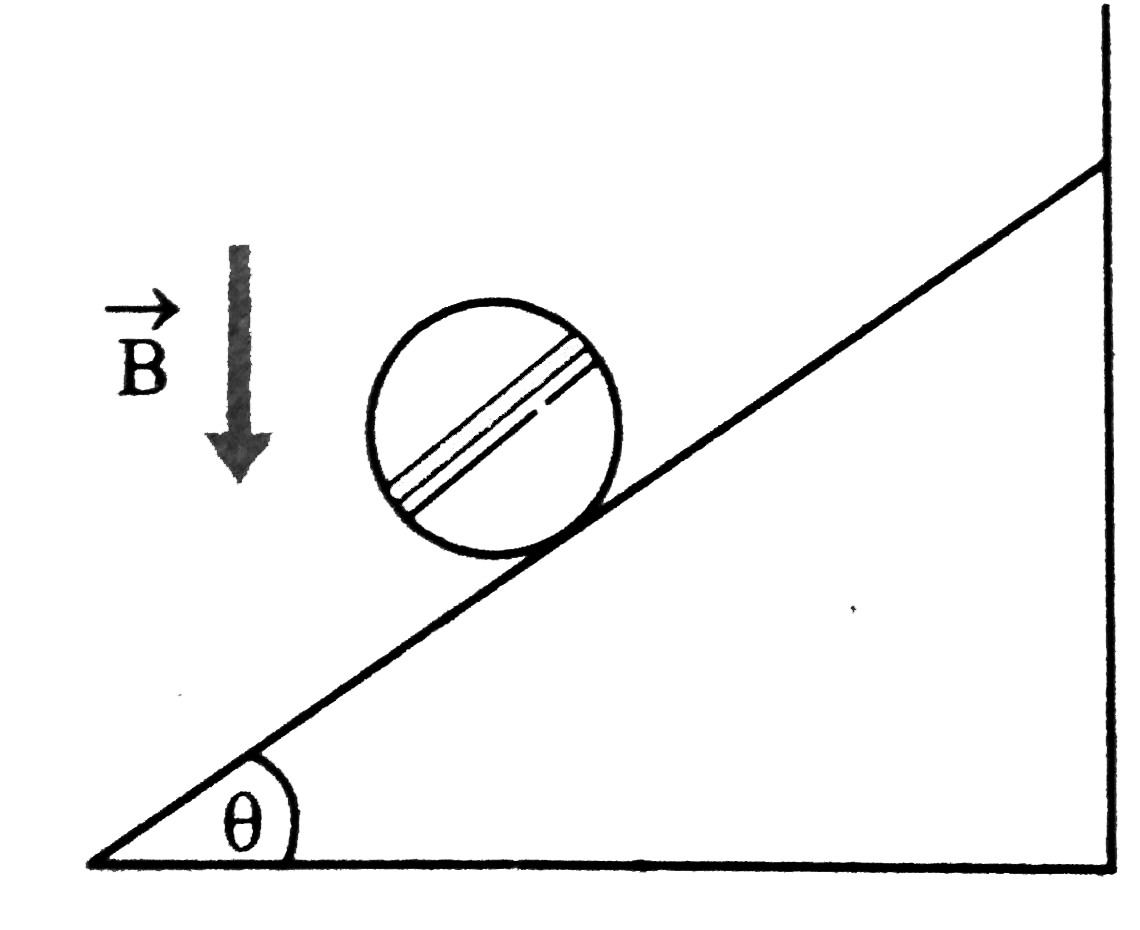
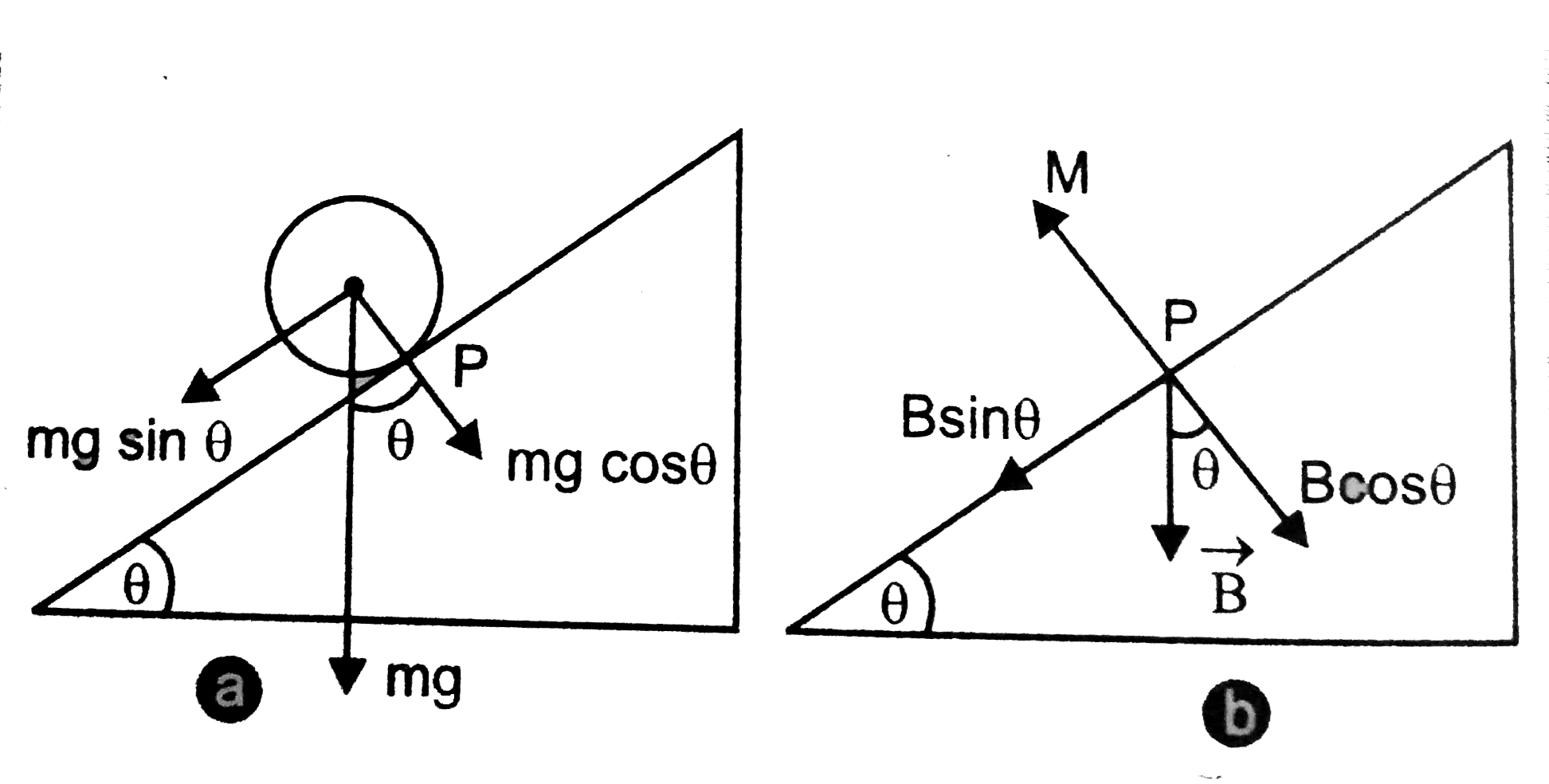
Magnetic dipole of moment of the coil carrying current, $M = I π R^{2}$
Its direction is perpendicular to the plane acting upwards. Resolving $\to B$ into two rectangular components, we have $B cos θ$ acting $⊥ r$ to the plane downwards and $B sin θ$ acting along the plane downwards, Fig.
For rotational equilibrium of sphere about point P is
$(m g sin θ) R = M (B sin θ)$ or $m g R sin θ = I π R^{2} B sin θ$ or $B = \frac{m g}{π I R}$

Updated on:21/07/2023

In Fig. a coil of single turn is wound on a sphere of radius r and mass m. The plane of the coil is parallel to the inclined plane and lies in the equatorial plane of the sphere. If the sphere is in rotational equilibrium, the value of B is [Current in the coil is i]

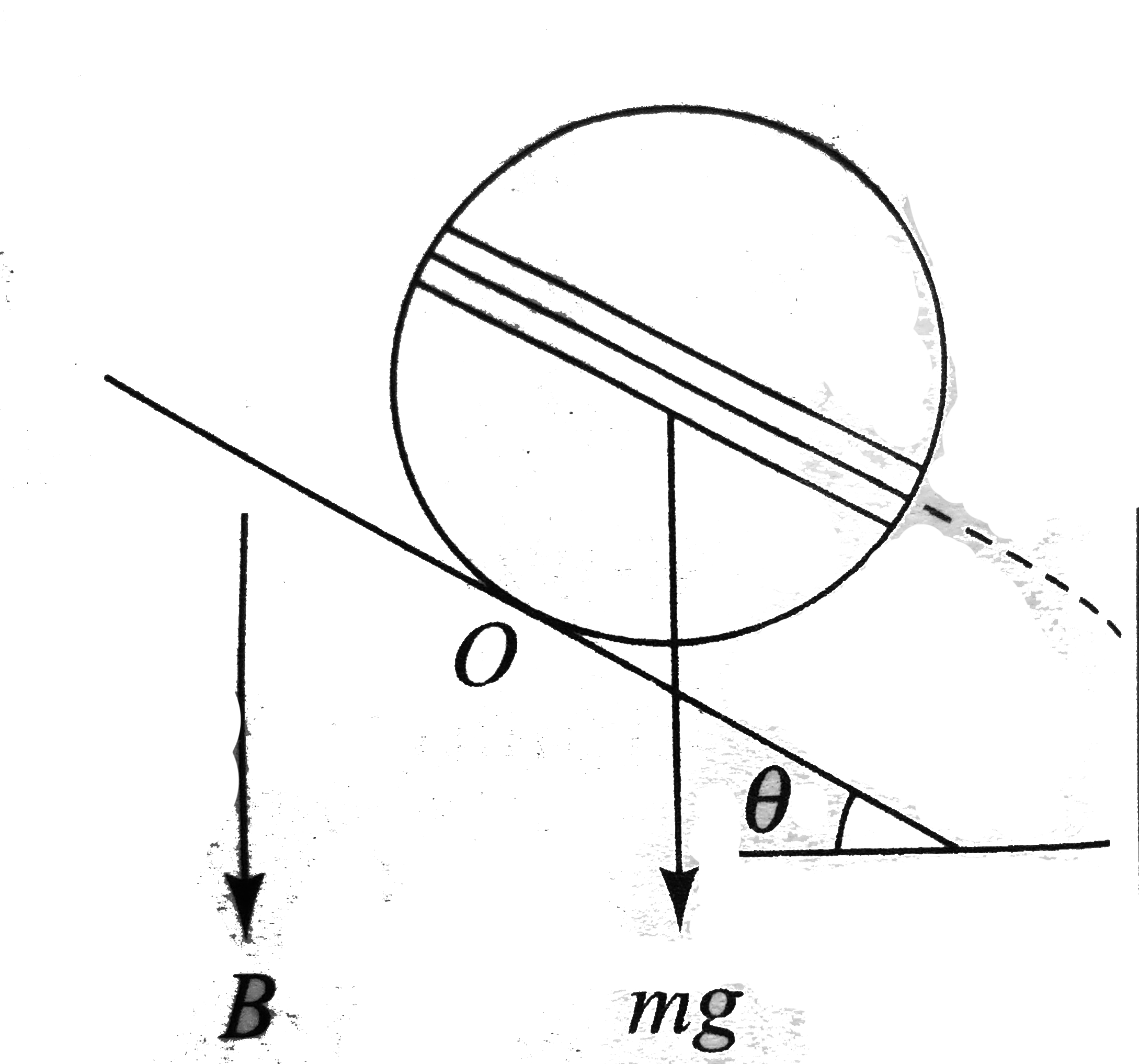A $\frac{m g}{π i r}$
B $\frac{m g sin θ}{π i}$
C $\frac{m g sin θ}{π i}$
DNone of these
Current $I$ is flowing through a circular coil of radius r. It is placed in a magnetic field B0 in such a way that the plane of the circular coil is perpendicular to B0. The force acting in it will be -
AParallel to B
BIs perpendicular to B
CAt $45^{\circ}$ with B
DAt $60^{\circ}$ with B
Three mutually, tangent spheres of radius 1 rest on a horizontal plane. A sphere of radius 2 rests on them. What is the distance from the plane to the top of the larger sphere?
A $3 + \frac{\sqrt{30}}{2}$
B $3 + \frac{\sqrt{69}}{3}$
C $3 + \frac{\sqrt{123}}{4}$
D $\frac{52}{9}$