A fine pencil of $β$ -particles, moving with a speed v, enters a region (region I), where a uniform electric field and a uniform magnetic field are both present. These $β$ -particles then move into region II where only the magnetic field, (out of the two fields present in region I) exists. The path of the $β$ -particles, in the two regions is as shown in the figure.

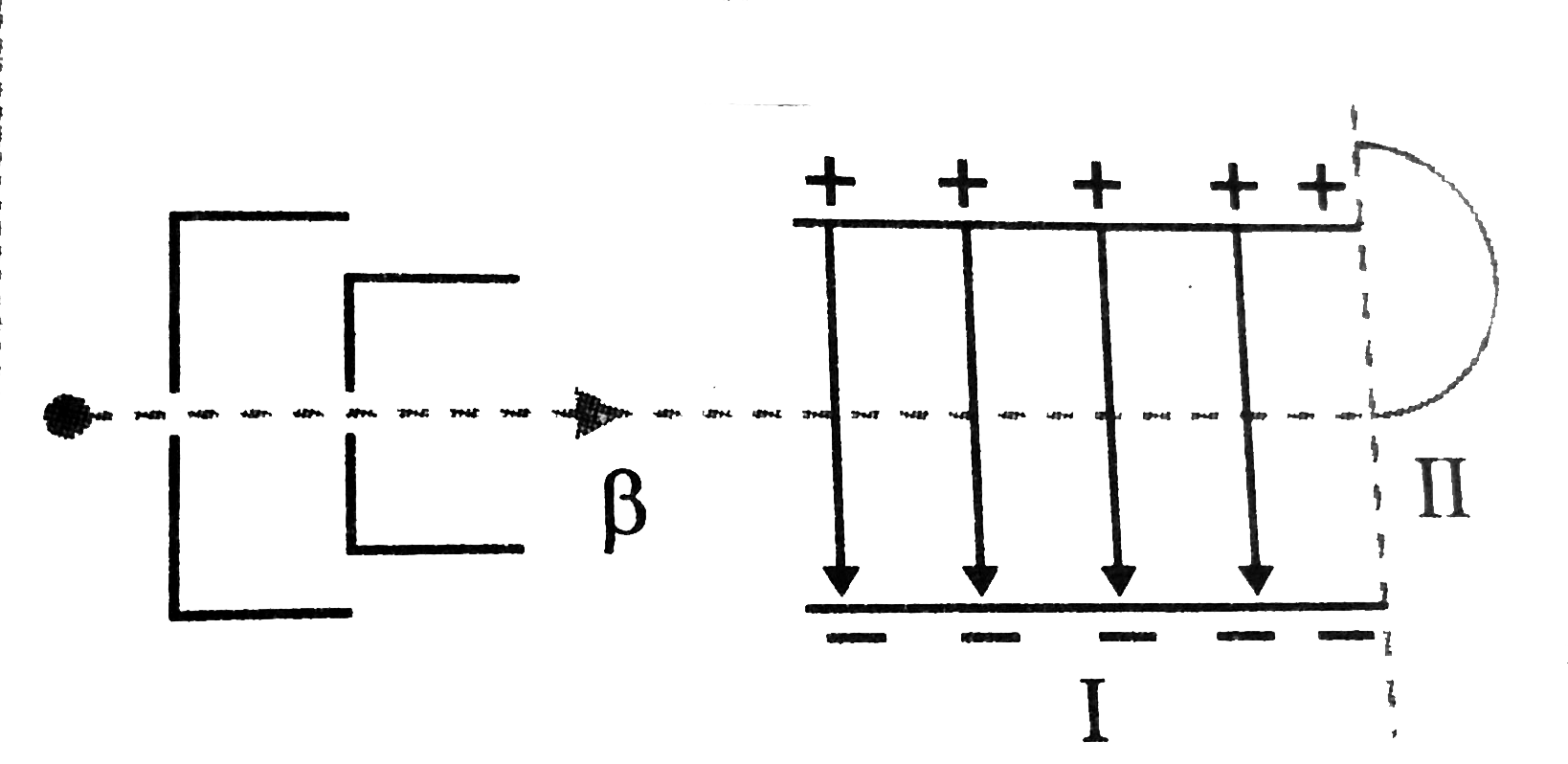
(i) state the direction of magnetic field.
(ii) state the relation between E and B in region I.
(iii) Drive the expression for the radius of the circular path of the $β$ -particle in region II.
(iv) If the magnitude of magnetic field, in region II is changed to n times its earlier value, (without changing the magnetic field in region I) find the factor by which the radius of this circular path would change.

Video Solution

Text Solution

Verified by Experts

(i) As the $β$ -particle goes undeviated while passing through the electric and magnetic fields, so the force on moving $β$ -particle due to electric field $(- e \to E)$ must be equal and opposite to the force due to magnetic field $[- e (\to v \times \to B)]$ . Due to it, the direction of $\to B$ must be perpendicular to plane of paper downwards.
(ii) In region I as $β$ -particle goes undeviated
$- e \to E = - e (\to v \times \to B)$ or $e E = e v B$ or $v = E / B$
(iii) If r is the radius of circular path of the $β$ -particle in region II, then
$e v B = m v^{2} / r$ or $r = \frac{m v}{e B}$
(iv) If $B^{'} = n B$ , then $r^{'} = \frac{m v}{e B^{'}} = \frac{m v}{ne B}$
$∴ \frac{r^{'}}{r} = \frac{1}{n}$ or $r^{'} = \frac{r}{n}$

Updated on:21/07/2023