A mass $m = 100$ gram is attached at the end of a light spring which oscillates on a frictionless horizontal table with an amplitude equalt to $0.16 m$ and time period equal to 2 second. Initially the mass is released from rest at $t = 0$ and displacement $x = - 0.16$ metre. The expression for the displacement of mass at any time $(t)$ is

A

$x - 0.16 cos (π t)$

B

$x = - 0.16 cos (π t)$

C

$x = 0.16 cos (π t + π)$

D

$x = - 0.16 cos (π t + π)$

Video Solution

Text Solution

Generated By DoubtnutGPT

The correct Answer is:B, C

To find the expression for the displacement of the mass attached to the spring at any time $t$ , we can follow these steps:
Step 1: Identify the parameters
We have the following parameters:
- Mass $m = 100$ grams (which is $0.1$ kg)
- Amplitude $A = 0.16$ m
- Time period $T = 2$ seconds
- Initial displacement $x (0) = - 0.16$ m (released from rest)
Step 2: Calculate the angular frequency
The angular frequency $ω$ is given by the formula:
$ω = \frac{2 π}{T}$
Substituting the time period:
$ω = \frac{2 π}{2} = π rad/s$
Step 3: Write the general equation for SHM
The general equation for simple harmonic motion (SHM) can be expressed as:
$x (t) = A sin (ω t + ϕ_{0})$
where $ϕ_{0}$ is the phase constant.
Step 4: Determine the phase constant $ϕ_{0}$
At $t = 0$ , the displacement is given as $x (0) = - 0.16$ m. Thus, we can write:
$- 0.16 = 0.16 sin (ϕ_{0})$
This simplifies to:
$sin (ϕ_{0}) = - 1$
The angle for which $sin (ϕ_{0}) = - 1$ is:
$ϕ_{0} = \frac{3 π}{2} rad$
Step 5: Substitute $ω$ and $ϕ_{0}$ into the equation
Now we can substitute $ω$ and $ϕ_{0}$ back into the general equation:
$x (t) = 0.16 sin (π t + \frac{3 π}{2})$
Step 6: Simplify the equation
Using the sine addition formula, we can express $sin (π t + \frac{3 π}{2})$ as:
$sin (π t + \frac{3 π}{2}) = - cos (π t)$
Thus, the equation becomes:
$x (t) = 0.16 \cdot (- cos (π t)) = - 0.16 cos (π t)$
Final Expression
The expression for the displacement of the mass at any time $t$ is:
$x (t) = - 0.16 cos (π t)$
---

|

Updated on:7/8/2024

Class 11 PHYSICS SIMPLE HARMONIC MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A bead of mass m is attached to the mid-point of a taut, weightless string of length l and placed on a frictionless horizontal table.

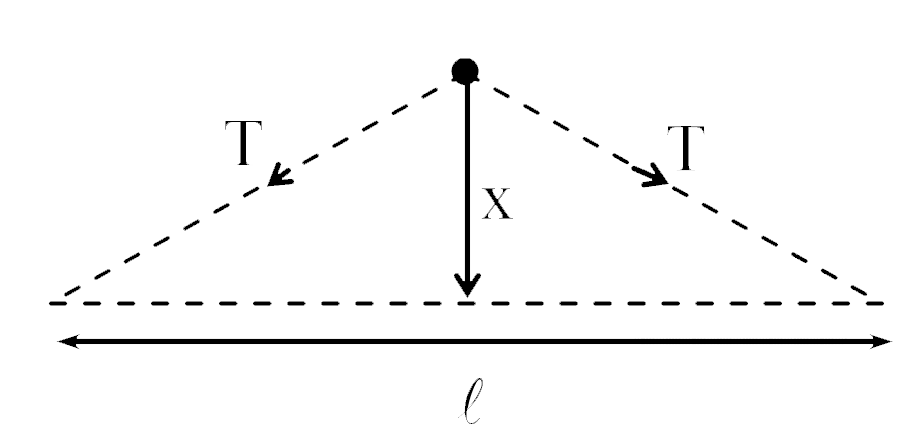
Under a small transverse displacement x, as shown, if the tension in the string is T, then the frequency of oscillation is-
A $\frac{1}{2 π} \sqrt{\frac{2 T}{m l}}$
B $\frac{1}{2 π} \sqrt{\frac{4 T}{m l}}$
C $\frac{1}{2 π} \sqrt{\frac{4 T}{m}}$
D $\frac{1}{2 π} \sqrt{\frac{2 T}{m}}$
Question 2 - Select One
A mass $m$ , which is attached to a spring with spring constant $k$ , oscillates on a horizontal table, with amplitude $A$ . At an instant when the spring is streched by $\sqrt{3} A / 2$ , a second mass $m$ is dropped vertically onto the original mass and immediately sticks to it. what is the amplitude of the resulting motion-
A $\sqrt{\frac{3}{2}} A$
B $\sqrt{\frac{7}{8}} A$
C $\sqrt{\frac{13}{16}} A$
D $\sqrt{\frac{2}{3}} A$
Question 3 - Select One
Two masses $m_{1}$ and $m_{2}$ connected by a spring of spring constant k rest on a frictionless surface. If the masses are pulled apart and let go, the time period of oscillation is-
A $T = 2 π \sqrt{\frac{1}{k} (\frac{m_{1} m_{2}}{m_{1} + m_{2}})}$
B $T = 2 π \sqrt{\frac{1}{k} (\frac{m_{1} + m_{2}}{m_{1} m_{2}})}$
C $T = 2 π \sqrt{(\frac{m_{1}}{k})}$
D $T = 2 π \sqrt{(\frac{m_{2}}{k})}$

PRADEEP-OSCILLATIONS AND WAVES-JEE mains adv..(multiple choice quection)

Home
Ask Doubt
Profile