The pressure of a gas is increased by 50% at constant temperature. The decrease in volume will be nearest to
66%
33%
17%
8%
The correct Answer is:B
To solve the problem, we will use the ideal gas law and the relationship between pressure and volume at constant temperature.
1. Understanding the Problem:
- We are given that the pressure of a gas is increased by 50% at constant temperature. We need to find the decrease in volume.
2. Initial and Final Pressure:
- Let the initial pressure be P.
- An increase of 50% means the final pressure P2 is:
P2=P+0.5P=1.5P
3. Using the Ideal Gas Law:
- According to the ideal gas law, at constant temperature, the product of pressure and volume is constant:
P1V1=P2V2
- Here, P1=P, V1 is the initial volume, and V2 is the final volume.
4. Substituting Values:
- Substitute P1 and P2 into the equation:
PV1=(1.5P)V2
5. Cancelling Pressure:
- We can cancel P from both sides (assuming P≠0):
V1=1.5V2
6. Rearranging for Final Volume:
- Rearranging gives:
V2=V11.5=23V1
7. Finding the Decrease in Volume:
- The decrease in volume ΔV can be calculated as:
ΔV=V1−V2=V1−23V1=13V1
8. Calculating the Percentage Decrease:
- The percentage decrease in volume is given by:
Percentage Decrease=(ΔVV1)×100=⎛⎝13V1V1⎞⎠×100=1003≈33.33%
Final Answer:
The decrease in volume will be nearest to 33.33%.
---
- We are given that the pressure of a gas is increased by 50% at constant temperature. We need to find the decrease in volume.
- Let the initial pressure be P.
- An increase of 50% means the final pressure P2 is:
P2=P+0.5P=1.5P
- According to the ideal gas law, at constant temperature, the product of pressure and volume is constant:
P1V1=P2V2
- Here, P1=P, V1 is the initial volume, and V2 is the final volume.
- Substitute P1 and P2 into the equation:
PV1=(1.5P)V2
- We can cancel P from both sides (assuming P≠0):
V1=1.5V2
- Rearranging gives:
V2=V11.5=23V1
- The decrease in volume ΔV can be calculated as:
ΔV=V1−V2=V1−23V1=13V1
- The percentage decrease in volume is given by:
Percentage Decrease=(ΔVV1)×100=⎛⎝13V1V1⎞⎠×100=1003≈33.33%
The decrease in volume will be nearest to 33.33%.
Topper's Solved these Questions
- THERMODYNAMICSBook:PRADEEPChapter:THERMODYNAMICSExercise:Interger Type questions
Explore 11 Videos
- THERMODYNAMICSBook:PRADEEPChapter:THERMODYNAMICSExercise:Assertion- Reason Type Questions
Explore 19 Videos
- THERMODYNAMICSBook:PRADEEPChapter:THERMODYNAMICSExercise:Multiple choice questions (NCERT)
Explore 10 Videos
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBook:PRADEEPChapter:SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONExercise:Assertion- Reason Type questions
Explore 20 Videos
- WORK, ENERGY AND POWERBook:PRADEEPChapter:WORK, ENERGY AND POWERExercise:Assertion-Reason Type Questions
Explore 24 Videos
Similar Questions
If the pressure of a gas is increased by 10% and the volume is increased by 20%, then
View SolutionA given mass of ideal gas has volume V at pressure P and room temperature T. Its pressure is first increased by 50% and then decreased by 50% (both at constant temperature). The volume becomes
View Solution
Knowledge Check
Assertion : On reducing the value of a gas at constant temperature, the pressure of the gas increase.
Reason : At constant temperature according to Boyle's law, volume is inversely proportional to pressure.AIf both assertion and reason are true and the reason is correct explanation of the assertion.BIf both assertion and reason are true but reason is not the correct explanation of assertion.CIf assertion is true, but the reason is false.DIf assertion is false but the reason is trueIf the volume of an ideal gas decreased by 5% at constant pressure, the increase of pressure is
A0.05B0.0518C0.0526D0.0541When the volumn of a gas is decreased at constant temperature the pressure increases because the molecules
Astrike the unit area of the walls of the container more often.Bstrike the unit area of the walls of the container with higher speedCstrike the unit area of the wall of the container with lesser speed.Dmove with more kinetic energy
The percentage change in the pressure of a given mass of a gas at constant temperature is 50% (increases). Calculate the percentage in the pressure of the gas.
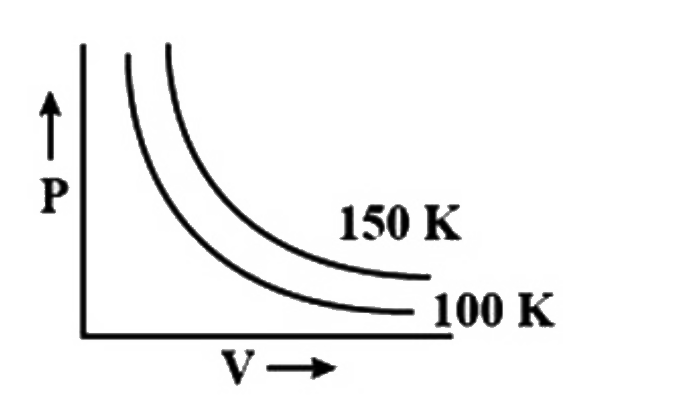
View SolutionA graph is plotted between pressure and volume at different temperature. On the basis of the graph what changes will you observe in the volume if
(i) the pressure is increased at constant temperature
(ii) the temperature is decreased at constant pressure
View Solution" When the pressure on a gas is decreased to "1/4" and the absolute temperature is increased four-fold the volume of the gas "
View SolutionIf the height of a cone is increased by 50%, then what is the percentage increase in the volume of the cone?
View SolutionIf the temperature of a gas is increased by 1K at constant pressure its volume increase by 0.0035 of the initial volume. The temperature of the gas is
View Solution
