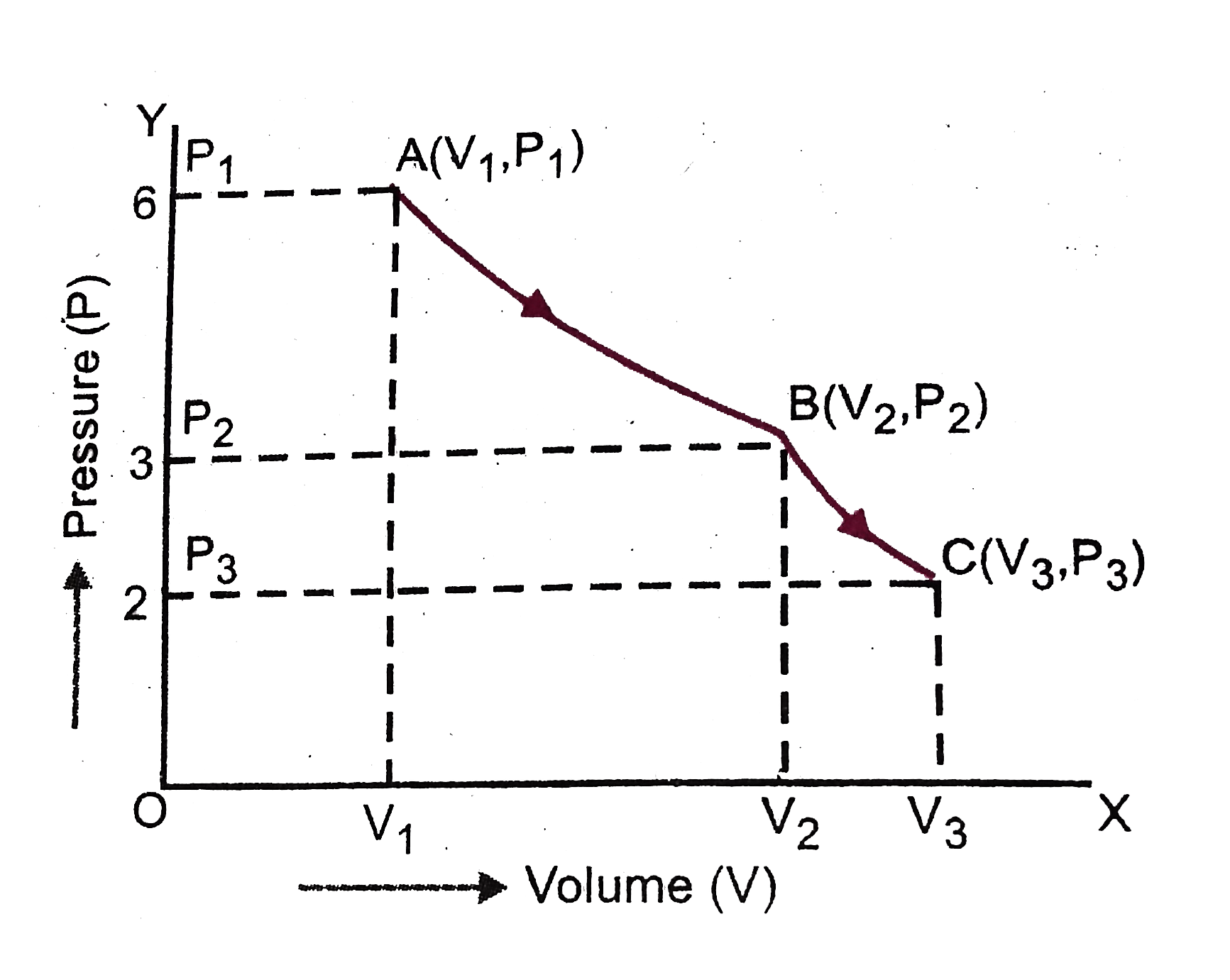
The initial volume of an ideal gas is $V_{1}$ and the initial pressure is $P_{1} = 6$ atmosphere. It expands isothermally to a volume $V_{2}$ and pressure $P_{2} = 3 a t m$ , and then adiabatically to a volume $V_{3}$ and pressure $P_{3} = 2 a t m$ . Draw a rough sketch of these changes. Calculate the value of $V_{2} / V_{1} and V_{3} / V_{2}$ .
Take $γ = 1.4$ .

Video Solution

Text Solution

Verified by Experts

In (figure), initial state of gas is represented by A $(V_{1}, P_{1})$ . AB represents isothermal change to B $(V_{2}, P_{2})$
BC represents adiabatic change from B $(V_{2}, P_{2})$ to $C (V_{3}, P_{3})$ .
As change from A to B is isothermal
$∴ P_{1} V_{1} = P_{2} V_{2} or \frac{V_{2}}{V_{1}} = \frac{P_{1}}{P_{2}} = \frac{6}{3} = 2$
As change from B to C is adiabatic,
$∴ P_{2} V_{2}^{γ} = P_{3} V_{3}^{γ} or {(\frac{V_{3}}{V_{2}})}^{γ} = \frac{P_{2}}{P_{3}}$
or $\frac{V_{3}}{V_{2}} = {(\frac{P_{2}}{P_{3}})}^{1 / γ} = {(\frac{3}{2})}^{1 / γ} = {(1.5)}^{1 / γ} = {(1.5)}^{1 / 1.4} = {(1.5)}^{5 / 7} = 1.33$

Updated on:21/07/2023

A monatomic gas at a pressure P, having a volume V expands isothermally to a volume 2 V and then adiabatically to a volume 16 V. The final pressure of the gas is ( take $γ = \frac{5}{3}$ )
A64 P
B32 P
C $\frac{P}{64}$
D16 P
A monatomic gas at a pressure P, having a volume V expands isothermally to a volume 2 V and then adiabatically to a volume 16 V. The final pressure of the gas is ( take $γ = \frac{5}{3}$ )
A64 P
B32 P
CP/64
D16 P
An ideal gas with pressure P, volume V and temperature T is expanded isothermally to a volume 2V and a final pressure $P_{1}$ . The same gas is expanded adiabatically to a volume 2V, the final final pressure is $P_{A}$ . In terms of the ratio of the two specific heats for the gas $γ$ , the ratio $P_{I} / P_{A}$ is
A $2^{γ - 1}$
B $2^{1 - γ}$
C $2^{γ}$
D $2 γ$