Three identical rods A, B and C are placed end to end. A temperature difference is maintained between the free ends of A and C. The thermal conductitvity of B is thrice that of C and half that of A. The effective thermal conductivity of rod A)
13KA
3KA
2KA
23KA
The correct Answer is:A
To find the effective thermal conductivity of the three rods A, B, and C placed end to end, we can follow these steps:
Step 1: Define the thermal conductivities
Let:
- KC = thermal conductivity of rod C
- KB=3KC = thermal conductivity of rod B (given that it is thrice that of C)
- KA=2KB = thermal conductivity of rod A (given that it is half that of A)
From the above relationships, we can express KA in terms of KC:
- KB=3KC
- KA=2KB=2(3KC)=6KC
Step 2: Calculate the thermal resistance of each rod
The thermal resistance R of a rod can be calculated using the formula:
R=LK⋅A
where L is the length of the rod, K is the thermal conductivity, and A is the cross-sectional area.
For the three rods:
- RA=LKA⋅A=L6KC⋅A
- RB=LKB⋅A=L3KC⋅A
- RC=LKC⋅A=LKC⋅A
Step 3: Calculate the total thermal resistance
Since the rods are in series, the total thermal resistance Rtotal is the sum of the individual resistances:
Rtotal=RA+RB+RC
Substituting the values:
Rtotal=L6KC⋅A+L3KC⋅A+LKC⋅A
Step 4: Simplify the expression
To simplify, we can find a common denominator, which is 6KC⋅A:
Rtotal=L6KC⋅A+2L6KC⋅A+6L6KC⋅A
Rtotal=L+2L+6L6KC⋅A=9L6KC⋅A=3L2KC⋅A
Step 5: Calculate the effective thermal conductivity
The effective thermal conductivity Keff can be calculated using the formula:
Rtotal=LKeff⋅A
Equating the two expressions for Rtotal:
3L2KC⋅A=LKeff⋅A
Cancelling L and A from both sides:
32KC=1Keff
Thus, rearranging gives:
Keff=2KC3
Final Answer
The effective thermal conductivity of the three rods is:
Keff=2KC3
Let:
- KC = thermal conductivity of rod C
- KB=3KC = thermal conductivity of rod B (given that it is thrice that of C)
- KA=2KB = thermal conductivity of rod A (given that it is half that of A)
- KB=3KC
- KA=2KB=2(3KC)=6KC
The thermal resistance R of a rod can be calculated using the formula:
R=LK⋅A
where L is the length of the rod, K is the thermal conductivity, and A is the cross-sectional area.
- RA=LKA⋅A=L6KC⋅A
- RB=LKB⋅A=L3KC⋅A
- RC=LKC⋅A=LKC⋅A
Since the rods are in series, the total thermal resistance Rtotal is the sum of the individual resistances:
Rtotal=RA+RB+RC
Substituting the values:
Rtotal=L6KC⋅A+L3KC⋅A+LKC⋅A
To simplify, we can find a common denominator, which is 6KC⋅A:
Rtotal=L6KC⋅A+2L6KC⋅A+6L6KC⋅A
Rtotal=L+2L+6L6KC⋅A=9L6KC⋅A=3L2KC⋅A
The effective thermal conductivity Keff can be calculated using the formula:
Rtotal=LKeff⋅A
Equating the two expressions for Rtotal:
3L2KC⋅A=LKeff⋅A
Cancelling L and A from both sides:
32KC=1Keff
Thus, rearranging gives:
Keff=2KC3
The effective thermal conductivity of the three rods is:
Keff=2KC3
Topper's Solved these Questions
- PROPERTIES OF BULK MATTERBOOK - PRADEEPCHAPTER - PROPERTIES OF BULK MATTEREXERCISE - Integer Type Questions
12 Videos
- PROPERTIES OF BULK MATTERBOOK - PRADEEPCHAPTER - PROPERTIES OF BULK MATTEREXERCISE - Asseration - Reason Type Question
18 Videos
- PROPERTIES OF BULK MATTERBOOK - PRADEEPCHAPTER - PROPERTIES OF BULK MATTEREXERCISE - Multiple choice questions-II
14 Videos
- PHYSICAL WORLD AND MEASUREMENTBOOK - PRADEEPCHAPTER - PHYSICAL WORLD AND MEASUREMENTEXERCISE - Competiton Focus Jee Medical Entrance
18 Videos
- RAY OPTICSBOOK - PRADEEPCHAPTER - RAY OPTICSEXERCISE - Problem For Practice(a)
25 Videos
Similar Questions
Three identical metal rods A, B and C are placed end to end and a temperature difference is maintained between the free ends of A and C. If the thermal conductivity of B(KB) is twice that of C(KC) and half that of A(KA), (KA=49w/mK) calculate the effective thermal conductivity of the system ?
View SolutionOne end of a uniform brass rod 20 cm long and 10cm2 cross-sectional area is kept at 100∘C. The other end is in perfect thermal contact with another rod of identical cross-section and length 10 cm. The free end of this rod is kept in melting ice and when the steady state has been reached, it is found that 360 g of ice melts per hour. Calculate the thermal conductivity of the rod, given that the thermal conductivity of brass is 0.25cal/scm∘CandL=80cal/g.
View Solution
Knowledge Check
Three metal rod A, B and C of same length and cross-section are placed end to end and a temperature difference is maintained between the free ends of A and C. If the thermal conductivity of B(KB) is twice that of C(KC) and half that of A(KA), then the effective thermal conductivity of the system will be
AKA7B6KB7C7KB3D7KCTwo rods A and B of different materials are welded together as shown in figure. Their thermal conductivities are K1 and K2. The thermal conductivity of the composite rod will be
 AK1+K22B3(K1+K2)2CK1+K2D2(K1+K2)
AK1+K22B3(K1+K2)2CK1+K2D2(K1+K2)Two rods A and B of different materials are welded together as shown in figure. Their thermal conductivities are K1 and K2. The thermal conductivity of the composite rod will be
 A3(K1+K2)2BK1+K2C2(K1+K2)DK1+K22
A3(K1+K2)2BK1+K2C2(K1+K2)DK1+K22
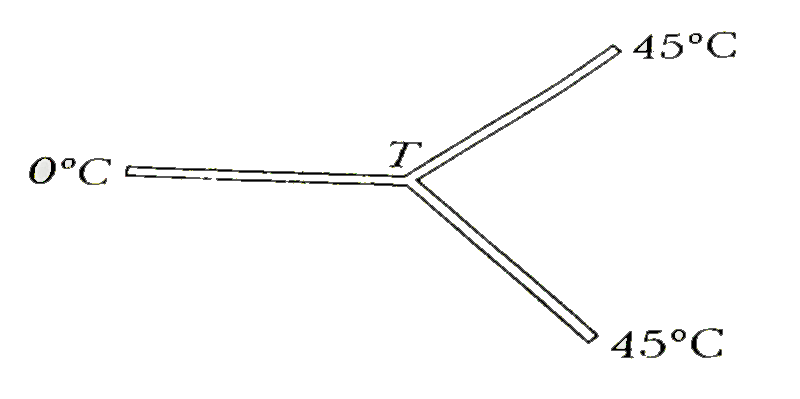
Three identical rods have been joined at a junction to make it a Y shape structure. If two free ends are maintained at 45∘C and the end is at 0∘C, then what is the junction temperature T?
View SolutionTwo rods A and B of different materials are welded together as shown in figure. Their thermal conductivities are K1 and K2. The thermal conductivity of the composite rod will be

View SolutionA composite rod made of three rods of equal length and cross-section as shown in fig. The thermal conductivites of the materials of the rods are K/2, 5K and K respectively. The end A and end B are at constant tempertures. All heat entering the face A goes out of the end B there being no loss of heat from the sides of the bar. The effective thermal conductivity of the bar is

View SolutionFour identical rods of same material are joined end to end to form a square. If the temperature difference between the ends of a diagonal is 100∘C, then the temperature difference between the ends of other diagonal will be
View SolutionTwo rods of copper and brass having the same length and cross - section are joined end to end. The free end of the copper is at 0∘C and of brass is at 80∘C in steady-state. If thermal conductivity of copper is 4 times of that of brass find the temp. at junction of two rods
View Solution
