The two thigh bones (femurs) each of cross-sectional are 10cm2 support the upper part of a human bodu of mass 40 kg . Estimate the average pressure sustained by the femurs. g=10m/s2
To solve the problem of estimating the average pressure sustained by the femurs, we can follow these steps:
Step 1: Identify the given data
- Cross-sectional area of each femur: A=10cm2
- Mass of the body: m=40kg
- Acceleration due to gravity: g=10m/s2
Step 2: Convert the cross-sectional area from cm² to m²
To convert the area from cm² to m², we use the conversion factor 1cm2=10−4m2:
A=10cm2=10×10−4m2=10−3m2
Step 3: Calculate the total area for both femurs
Since there are two femurs, the total cross-sectional area Atotal is:
Atotal=2×A=2×10−3m2=2×10−3m2
Step 4: Calculate the force exerted by the body
The force F due to the weight of the body can be calculated using the formula:
F=m×g
Substituting the values:
F=40kg×10m/s2=400N
Step 5: Calculate the average pressure sustained by the femurs
Pressure P is defined as force per unit area:
P=FAtotal
Substituting the values:
P=400N2×10−3m2=4000.002=200000N/m2
Thus, the average pressure is:
P=2×105N/m2=2×105Pa
Final Answer:
The average pressure sustained by the femurs is 2×105Pa.
---
- Cross-sectional area of each femur: A=10cm2
- Mass of the body: m=40kg
- Acceleration due to gravity: g=10m/s2
To convert the area from cm² to m², we use the conversion factor 1cm2=10−4m2:
A=10cm2=10×10−4m2=10−3m2
Since there are two femurs, the total cross-sectional area Atotal is:
Atotal=2×A=2×10−3m2=2×10−3m2
The force F due to the weight of the body can be calculated using the formula:
F=m×g
Substituting the values:
F=40kg×10m/s2=400N
Pressure P is defined as force per unit area:
P=FAtotal
Substituting the values:
P=400N2×10−3m2=4000.002=200000N/m2
Thus, the average pressure is:
P=2×105N/m2=2×105Pa
The average pressure sustained by the femurs is 2×105Pa.
Topper's Solved these Questions
- PROPERTIES OF BULK MATTERBook:PRADEEPChapter:PROPERTIES OF BULK MATTERExercise:Conceptual Problems
Explore 103 Videos
- PROPERTIES OF BULK MATTERBook:PRADEEPChapter:PROPERTIES OF BULK MATTERExercise:Advanced problems for competitions
Explore 21 Videos
- PROPERTIES OF BULK MATTERBook:PRADEEPChapter:PROPERTIES OF BULK MATTERExercise:Long Answer questions
Explore 33 Videos
- PHYSICAL WORLD AND MEASUREMENTBook:PRADEEPChapter:PHYSICAL WORLD AND MEASUREMENTExercise:Competiton Focus Jee Medical Entrance
Explore 18 Videos
- RAY OPTICSBook:PRADEEPChapter:RAY OPTICSExercise:Problem For Practice(a)
Explore 25 Videos
Similar Questions
The cross-sectional area of water pipe entering the basement is 4cm−2 . The pressure at the point is 3.5×105Nm−2 and the speed of water is 2.2ms−1 . This pipe tapers to a cross-sectional area of 2cm2 when it reaches the second floor 10 m above . Calculate the speed and pressure at the second floor. use g=10m/s2.
View SolutionThe foot of an elephant has an area of 275cm2. If the mass of the elephant is 2200 kg, find the pressure exerted by the on the ground. (g=10ms−2)
View Solution
Knowledge Check
The two femurs each of cross-sectional area 10cm2 support the upper part of a human body of mass 40kg.theavera≥pressuresusta∈edbythefeμrsis(takeg=10 ms^(-2))
A2×103Nm−2B2×104Nm−2C2×105Nm−2D2×106Nm−2The two thigh bones each of cross-sectional area 15cm2 support the upper part of a person of mass 70 kg. The pressure sustained by these thigh bones is
A2.5×105Nm2B1.33×105Nm2C4.66×105×105Nm2D2.33×105Nm2A steel rod of cross-sectional area 16cm2 and two brass rods each of cross-sectional area 10cm2 together support a load of 5000kg as shown in the figure. ( Given, Ysteel=2×106kgcm−2andYbrass=106kgcm−2). Choose the correct option(s).
 AStress in brass rod=121kgcm−2BStress in steel rod=161kgcm−2CStress in brass rod =141kgcm−2DStress in steel rod =141kgcm−2
AStress in brass rod=121kgcm−2BStress in steel rod=161kgcm−2CStress in brass rod =141kgcm−2DStress in steel rod =141kgcm−2
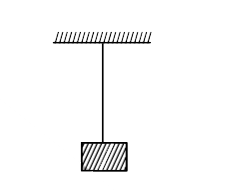
A 'block' of mass 10 kg is suspended with string as shown in figure. Find tension in the string. (g=10m/s2)
View SolutionFig show two bodies A and B of masses 2.5 kg and 2.8 kg respectively from a rigid support by two inextensible wires each of length 1.8 m . The upper wire is of negligible mass and lower wire is of mass 1.5kg/m . If the entire system moves upwards with an acceleration of 2m/s2, find tension (i) at middle point p of upper wire (ii) at middle point Q of lower wire . Take g=10m/s2
.
View SolutionA catapult consists of two parallel rubber cords each of length 20cm and cross-sectional area 5cm2. When stretched by 8cm, it can throw a stone of mass 4gm to a vertical height 5m, the Young's modulus of elasticity of rubber is [g=10m/sec2]
View SolutionFind the potential energy gained by a body of mass 50 kg, if it is raised by 10m. (use g = 10m/s2)
View SolutionAt the mount of the tap area of cross-section is 2.0cm2 and the speed of water is 3m/s. The area of cross-section of the water column 80 cm below the tap is (use g=10m/s2)
View Solution